Selection of the geometric and materials parameters in piezoelectric sensors level

1. Principle and the structure of the piezoelectric level sensor

Piezoelectric level sensor detects the presence of powder when the vibrated sensor element, so-called fork, comes into contact with powder or liquid and the vibrational forks are covered. Described sensor has both oscillations plates and plate work as sensors, for this reason it use both simple and inverse piezoelectric effect. Output signal is a binary signal, transmitted to the automation systems via a relay.

Fig. 1a) Computer model of level sensor and b) photo of piezoelectric bimorph with PZT plate

a)

b)

Analyzed sensor manufactured by the “Nivomer” company from Gliwice were shown on Fig. 1. The sensors consist of receiving plates and supplying plates, connected in a bimorphic system. Voltage, which is connected to the supply plates cause change in their thickness proportionally to the value of applied voltage. Changes of the plate thickness causing mechanical vibrations of the element. When the “forks” are not covered, full deformation of supplying plates are transferred to the receiving ones. The value of this voltage is transformed by an electronic system. In case of covered “forks”, the receiving plates are no longer stretched. At the same time the potential is not generated on the facing of the plates. In design of level sensors, the selection of parameters such as: well-chosen size plate and their number is very important.

2. Object under examination – mathematical model

In order to determine the matrix of piezoelectric flexibility, the piezoelectric is modelled as an element described with nine external parameters. Considering the mechanical system continuous in sections, where important parameters are: thickness, area and density of plates. Basis for the analysis of piezoelectric systems are constitutive equations presented in Eq. (1):

where: $E$ – the modulus of longitudinal elasticity, $E_P$ – the intensity value of electric field, $\epsilon$ – deformation, ${\epsilon }^S$ – the electric permeability, $D$ – the electric induction.

Assuming the expansion of the plate, mainly in the plane perpendicular to the axis, the following boundary conditions are written:

Mechanical stress by force was replaced, using the formula: $F=A\sigma \text{,}$ where $A$ is a piezoelectric plate surface, and $\sigma$ is the stress of piezoelectric plate. In analyzing the phenomena existing in the piezoelectric plate it is necessary consider electrical parameters such as voltages on the piezoelectric plates and current value. The voltage is therefore expressed as a function of electric field strength:

where: $U$ – value of the voltage generated on the plates of piezoelectric, $E_p$ – value of the electric field.

After conducting transformations presented in previous work of the authors [5, 8-11] it was obtained the relations between the mechanical parameters as force and displacement taking into account the voltage generated on the plates:

which are also written in a matrix form:

The matrix Eq. (7) was written as respond of system for operating extortion. The individual elements of matrix, presented as a flexibility, admittance and characteristics were recorded as follows:

• Mechanical dependences; force-displacement;

• Other dependences between mechanical and electrical parameters;

• Electrical parameters, so dependences voltage and the current flowing in the electrical circuit.

The mechanical dependencies were called flexibility, electrical dependencies were called conductivity, while the electrical and mechanical dependencies was called just characteristics.

3. Influence of piezoelectric plates number and material parameters on characteristics of sensor

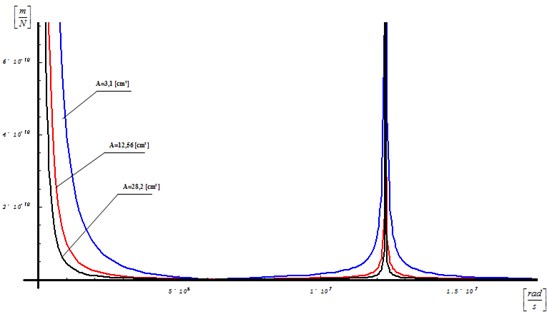
In this paragraph, graphical form of characteristics of piezoelectric plates in frequency domain were shown on Fig. 2, 3. The base for generating charts was equation of input and output dependences Eq. (7). In order to generate characteristic physical parameters of standard PZT material was adopted from Table 1. With presented mathematical model Eq. (7) of a single plate piezoelectric plate and already known methods for the analysis of complex systems [7, 11, 12]. On figures graphical charts of characteristics of fixed piezoelectric plates were shown with influence of different parameters, such as: the thickness (Fig. 2), the elastic modulus (Fig. 3) or surface area (Fig. 4).

It was noted that the characteristics of a single plate with thickness $d=$1 mm are the same as two connected piezoelectric plates with thickness $d=$ 0,5 mm each. This statement is correct only by assuming ideal connection of plates without for example taking the glue thickness layer into examination (Fig. 2).

Fig. 2Characteristics of one side fixed plates in the frequency domain with thickness d1=1 [mm], d2= 2 [mm], d3= 4 [mm]

Fig. 3Characteristics of fixed plates in the frequency domain with different elasticity modulus

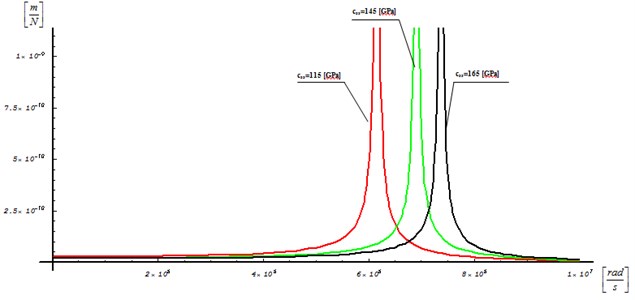
Fig. 4Graph of piezoelectric plate in the frequency domain, depending on the surface area

4. Application of mathematical model in industry sector

This article presents how to determine the flexibility of piezoelectric plates in vibration sensor level. In vibration level sensors bimorph systems are often used to increase the relative elongation, and thus obtain larger displacements. By properly mounting of piezoelectric element, there is the possibility of changes in the characteristics of a frequency function. While designing both the sensor systems and actuators, it is important to correctly interpret the signals transmitted by the piezoelectric. Until now, the sensors producers have to manual tune the frequency of the generator so that the vibration fork of sensor were correct. Currently, using the derived mathematical formula, faster and easier method for assessing the resonant frequency of physical and material the parameters was allowed. This paper indicates solution of engineering problem which is the choice of parameters of piezoelectric plates by using scientific methods of mathematical description.

5. Conclusions

The proposed analysis of the piezoelectric system at the design level can determine the parameters of piezoelectric plates. Well-chosen dimensions of the plates, and their number is crucial when performing other mechanical parts.

Following that, a preliminary analysis of the construction of the sensor with the Gliwice company “Nivomer” found that by introducing a variable number of platelets in the system may select a frequency generator and the maximum deflection of the fork-carrying vibrations.

Proposed in the methods and algorithms relating to complex systems can be applied to the piezoelectric stacks of tiles the design presented in the sensing level.