Rolling bearing defect detection and diagnostics

3.1. Time histories of acceleration and theirs spectrums

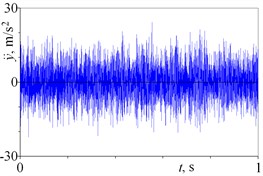
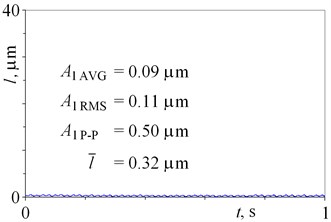
The time histories of acceleration are presented in Fig. 2, for the three directions $x$-$y$-$z$, and two time instances. As is evident from this figure, the vibration amplitudes rise and are bigger for $t_2=$65.472 h than for $t_1=$0 h. This shows that vibration amplitudes reflect the degradation of the rolling bearings. In this case, the amplitude in $z$-direction is the largest. Three amplitudes are widely used: average amplitude ($A_{AVG}$, $A_{xAVG}=\mathrm{\Sigma }\left|x_i-x_{mean}\right|/j$), root mean square amplitude ($A_{RMS}$), and peak-to-peak amplitude ($A_{P-P}$, $A_{xP-P}=(x_{max}-x_{min})$); thus they were calculated for the presented time histories (Table 1). As is evident from the obtained results, the presented amplitudes rise over two times. Moreover, the increases are the largest for peak-to-peak amplitude and $z$-direction, thus, these amplitudes should be used as a state indicator (e.g. $A_{\ddot{x}P-P}$ and $A_{\ddot{z}P-P}$,).

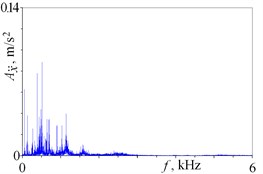
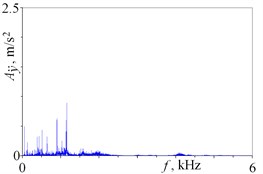
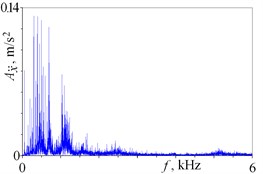
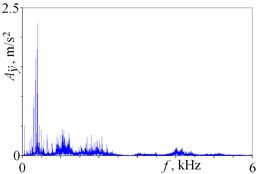
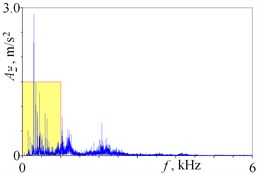
The time histories can be analysed with the Fourier transform, which provides an opportunity to find more state indicators and defects. As is evident from the obtained results, the largest amplitudes are observed for frequencies being below $f<$1 kHz; thus these peaks should be studied (Fig. 3). Some peaks are observed for frequencies being lager than $f>$1 kHz, and a number of them reflect natural frequencies, but they are not studied in this article. The spectrums obtained for $y$-direction and $z$-direction are similar. Nevertheless, the largest amplitude is observed for $z$-direction, and as $A_{\ddot{z}P-P}$ was indicated as a failure mode, this spectrum is studied below; whereas the shape of the spectrum presented in Fig. 3(d) suggests wear.

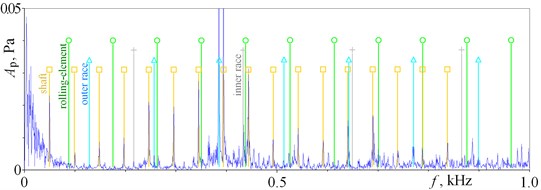
The magnified part of the spectrum obtained for the time history of acceleration $\ddot{z}$ and $t_2=$65.472 h (Fig. 3(f)) is depicted below (Fig. 4). Characteristic defect frequencies (CDF) are indicated with vertical lines, which usually show good agreement with peaks in the spectrum. First, the shaft frequencies are indicated with squares and yellow lines; good agreement with peaks leads to conclusion, that the shaft was unbalanced or curved. Next, ball spin frequencies are indicated with circles and green lines; but they do not match, thus the rolling elements should be in good shape. Nevertheless, if a defect of ball does not touch a race, which is possible, then this defect is not observed. Then, the ball bearings passing frequencies outer race are indicated with triangles and sky blue lines. Good agreement between these frequencies and peaks suggests clearance and outer race defects. Finally, ball bearings passing frequencies of an inner race are indicated with crosses and grey lines, but they do not match well, because sleep changes frequencies. Summarising, defects of the shaft and outer race were found with spectral analysis.

Fig. 2Time histories of acceleration obtained for two time instances: a), b) c) t1= 0 h and d), e), f) t2= 65.472 h, and three directions x-y-z

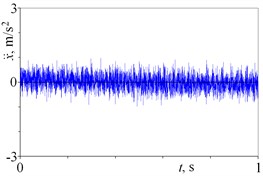
a)

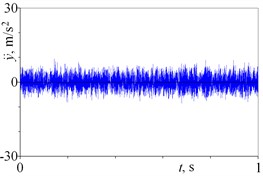
b)

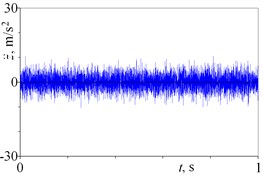
c)

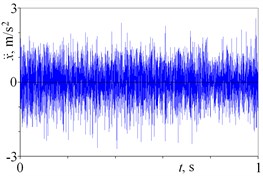
d)

e)

f)

Fig. 3Spectrums of time histories of acceleration obtained for two time instances: a), b) c) t1= 0 h and d), e), f) t2= 65.472 h and three directions x-y-z

a)

b)

c)

d)

e)

f)

3.2. Time histories of relative displacement

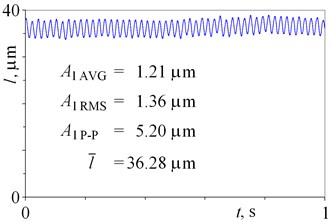
The time histories of the relative displacement between two housings $l$, which were measured with a LVDT (Linear Variable Differential Transformer) sensor (Fig. 1(b)), are depicted in Fig. 5. As is evident from the obtained results, amplitudes of vibration and average relative displacement $\stackrel{-}{l}$ rise. These amplitudes are over ten times lager for $t_2=$65.472 h than for $t_1=$0 h, and thus are good state indicators. Moreover, the average relative displacement $\stackrel{-}{l}$ provides an opportunity to estimate clearance. Nevertheless, shaft displacement is difficult to measure because the displacement is small, the shaft rotates, and the sensors are relatively large, thus proper sensors should be used. Because of these difficulties, this parameter is not usually measured.

Fig. 4Spectrum of time history of acceleration z¨ obtained for t2= 65.472 h

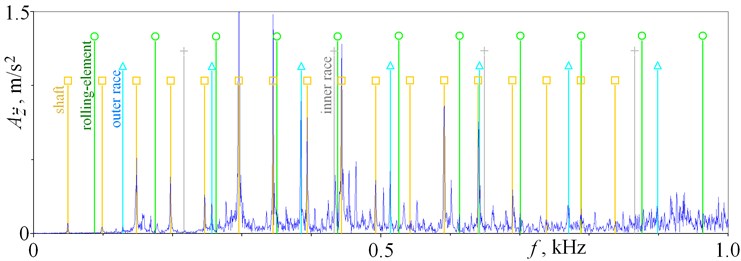
Fig. 5The time histories of the relative displacement between two housings obtained for two time instances a) t1= 0 h and b) t2= 65.472 h

a)

b)

3.3. The time histories of acoustic pressure and their spectrums

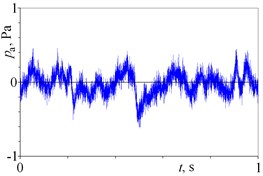
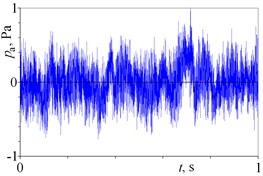
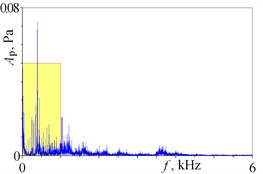
Acoustic pressure (noise) is usually neglected in machinery diagnostics, but in this section it has been studied. The time histories of acoustic pressure are depicted in Fig. 6(a), (b). As is evident from the obtained results, high frequency sounds are louder for $t_2=$65.472 h than for $t_1=$0 h. Moreover, low frequency noise is large (Fig. 6(a)), thus filtration and the Fourier transform should be used to obtain more information. The spectrums of the time histories of acoustic pressure (Fig. 6(c), (d)) are similar to the spectrums of the time histories of acceleration (Fig. 3), and thus should be analysed. The spectrum presented in Fig. 6(e) is very similar to the one in Fig. 4, and shows good agreement with characteristic defect frequencies. Defects of the shaft, outer race and inner race are obvious, whereas defects of the rolling elements are difficult to find. As is evident from the presented results, sounds emitted by this test stand provide valid information, which was not expected. Nevertheless, a microphone measures sounds emitted by all test stand, thus indication of defective rolling bearings is difficult. This is a disadvantage of this method.

3.4. Thermo-graphic analysis

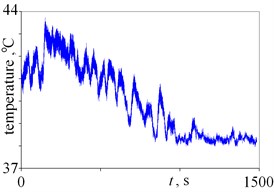
Temperature distribution is depicted in Fig. 7(a), (b). As is evident from the obtained results, the maximal temperature of a rolling bearing dropped from 38.4 °C to 36.3 °C. Moreover, the natural fluctuation of temperatures observed during twenty minutes is 5.8 °C (Fig. 7(c)). Finally, a number of factors influence the obtained results, for example, the loading force, lubricant parameters, air temperature, surface effects and thermal emissivity; thus temperature is not the best state indicator.

Fig. 6The time histories of acoustic pressure obtained for two time instances: a) t1= 0 h and b) t2= 65.472 h and their spectrums obtained for time instances: c) t1= 0 h and d), e) t2= 65.472 h

a)

b)

c)

d)

e)

Fig. 7Thermo-graphic pictures obtained with a FLIR AX5 camera for a) t1= 0 h and b) t2= 65.472 h, and temperature fluctuation of rolling bearings measured for c) t= 3.61 h

a)

b)

c)

Fig. 8Surfaces of: a) a ball, b) outer race, c) inner race

a)

b)

c)

3.5. Microscopic investigation

Microscopic investigation of rolling bearings, which was executed after the tests, provides valid information. It provides an opportunity to see the real defects. Fatigue of the balls (pitting) is depicted in Fig. 8(a). In consequence, defective balls and their fragments defect the inner race and outer race, thus indentations are observed in Fig. 8(b), (c). Finally, wearing of the shafts was found. These defects-apart from the defected balls, were found with spectral analysis (see Figs. 4, 6(e)), which shows good agreement between signals and the found defects. Thus, spectral analysis is very useful.