Research methodology for vibration isolation materials

2.1. Determination of volumetric density ${\mathit{\rho }}_0$

Volumetric density ${\rho }_0$ of the material should be determined in accordance with applicable standards in this case with Polish norm PN-EN ISO 845:2000 “Rubber and porous plastic material. Determination of volumetric density” [4] from the relationship:

where: $m_0$ – sample weight, $v_0$ – sample volume.

2.2. Determination of volumetric porosity ${\mathit{p}}_{\mathit{V}}$

Determination of volumetric porosity $p_V$ was carried out in accordance with international standard ISO 865:2005 “Plastics porous. Determination of the percentage volume of open and closed pores in rigid materials” [1, 2]. The volumetric porosity of the material $p_V$ is a measure of the volume fraction of the pores in the material and is calculated from the relationship:

where: $v_p$ – pore volume in the sample.

Due to the particle size rubber granules and a small thickness of the boards surveyed the vast majority of the pore is open. In this case, the specific by testing the open porosity virtually equal to the porosity volume $p_V$ plate. This observation has been used in this study to determine $p_V$. The test involves the expulsion of air from the open pores and filling them with water. This is achieved by placing the samples in boiling distilled water and then after cooling to define the change $∆m$ the mass of the sample. In view of the above described properties of the panel material:

where: $∆m$ – change of mass of sample after soaking in water, ${\rho }_{H_{2}O}$ – H₂O density.

2.3. Determination of shore A hardness

Determination of Shore A hardness was performed according to standard DIN EN ISO 868: 2005 “Plastics and hard rubber. Determination of hardness by pressing method using a durometer (Shore hardness)”. Hardness $\stackrel{-}{{Sh}_A}$ Shore A has been determined by carrying out at least 30 measurements of hardness for each sample with a durometer Shore Sauter HBA 100-0 scale A. Tests were performed at 20±2°C. On the basis of the individual results calculated the size of the arithmetic average hardness $\stackrel{-}{{Sh}_A}$ and its standard deviation ${Ϭ}_{Sh}$ the following formulas:

where: ${Sh}_{Ai}$ – individual result of measurement of hardness Shore A, $n$ – number of measurements ($n\ge$30).

Because of the large surface roughness, the porosity of the slabs and large pore size of the indenter relative to the measurements do not reflect the hardness of the plate on a macroscopic scale, but only the hardness of webs of the glued rubber granules used to perform a specific disc. Available on the market hardness for rubber and elastomeric materials allow for spot measurements and do not provide authoritative study feasibility of porous materials. On the basis of the average $\stackrel{-}{{Sh}_A}$ bridges of rubber and porosity volume $p_V$ board, the weighted average hardness $\stackrel{-}{{Sh}_{Aplate}}$. It is described as follows dependency and a reliable indicator of the hardness of the porous plate in terms of macroscopic:

where: $p_V$ – porosity by volume of the plate material vibration isolating.

2.4. Determination of the mechanical properties of vibration isolation plates based on the stabilized chart of hysteresis loop mechanical work

The modulus of elasticity (Young’s modulus) is calculated from secant of stabilized hysteresis loop mechanical work based on relation $E=\partial \sigma /\partial \epsilon$. In practice Young’s modulus can be calculated from equation $E=\mathrm{t}\mathrm{g}\alpha$ where $\alpha$ is the angle of inclination secant with respect to the horizontal axis of deformation $\epsilon$ coordinate system stress $\sigma$ and strain $\epsilon$. The above definition refers to the case of linear-elastic materials, the course of which resembles the hysteresis loop “spindle”, as illustrated in Fig. 2 and are successfully used for materials with low loss, and so a small span between the curves load and relief. In the case of rubber, the spread is large, so the averaged size of the $E$ individually relative to the curve progression or digression would be burdened with large errors.

The rubbers and other elastomeric materials exceeding a certain magnitude of deformation is the phenomenon of a non-linear elasticity. The hysteresis loop changes shape to guide individual sellers within the loop becomes impossible, therefore, cannot be set a modulus based on the above cited rule. The load curve of the hysteresis loop reflects the vibro-isolator response to external mechanical force the curve to relieve it reflects the relaxation of the material. For engineering applications of vibration isolating materials more important is knowledge of the load curve. Given the need to define the contractual elastic modulus $E$ it is therefore reasonable to base it on course load curve. An important size for evaluating the vibration isolating properties of the materials is their ability damping expressed by diffuse $\psi$ defined as:

where: $W_{loop}$ – the amount of work losses contributed by vibro-isolator due to internal friction and dispersed into the environment as heat. $W_{loop}$ is the difference between work $W_{load}$ is made in step progression in accordance with the load curve of the hysteresis loop, and the operation of the vibro-isolator dedicated phase in accordance with the decrease of the unloading curve, $W_{load}$ – work done by the external force F to deform the vibro-isolator by an set point deflection $\mathrm{\Delta }l$.

Fig. 2The hysteresis loop and damping coefficient of nonlinear-elastic materials

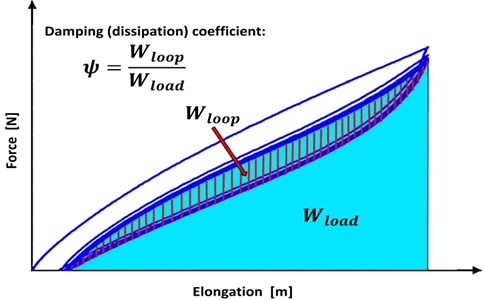
The definition of the physical work is expressed by the relation:

The coordinate system force $F$ – deflection $l$, $W_{load}$ corresponds to the size of the area under the load curve of the hysteresis loop and $W_{loop}$ is the difference between the size of the area under the curve of the load and the size of the area under the curve relief as illustrated in Fig. 3.

Fig. 3A measure of vibro-isolator damping

2.5. Determination of contractual linear deformation ${\mathit{\epsilon }}_{\mathit{L}}$

Contractual ${\epsilon }_L$ linear deformation is the maximum relative deformation $\epsilon$ rubber load curve in a compression test area corresponding to the linear elasticity of rubber in the scope of applicability of Hooke’s law. Contractual linear deformation ${\epsilon }_L$ size can calculated from the equation:

where: $∆l_L$ – change of the height of the sample during the compression test in area of linear elasticity stable hysteresis loop, $l_0$ – initial thickness of the sample.

2.6. Determination of contractual quasi-static and dynamic linear elastic modulus ${\mathit{E}}_{\mathit{L}}$ for a range of linear deflection $\mathit{\epsilon }=0÷{\mathit{\epsilon }}_{\mathit{L}}$

Contractual modulus of elasticity of rubber $E_L$ for a range of linear deflection is determined based on the size of the straight section of the load curve stable hysteresis loop compression mechanical work, corresponding to the area studied linear elasticity of rubber in the scope of applicability of Hooke’s law. $E_L$ value can be determined based on the data from the chart stabilized hysteresis loop based on the following equation:

where: $F_L$ – change of the value of force $F$ on the load curve hysteresis loop during compression corresponding to the region of linear elasticity, $S_0$ – output cross-section of the sample.

2.7. Determination of measurement of the damping coefficient $\mathit{\psi }$

The damping coefficient $\psi$ – also known as diffuse – is a dimensionless measure of the size of mechanical work loss due to internal friction in the body elastic and in accordance with Section 2.4 and Fig. 3 can be calculated with the relation:

In order to determine value of damping coefficient first the hysteresis loop chart has been digitized. Then the value of the area within the hysteresis loop corresponding to a stable work $W_{loop}$ and area under the curve corresponding to the work load $W_{load}$ were calculated and based on these values the ${\mathrm{\Psi }}_{\epsilon =20\%}$ value was calculated.

2.8. Determination of quasi-static and dynamic stiffness factor $\mathit{k}$

Volume stiffness coefficient $k$ is defined as a derivative of the force inducing strain relative to deformation. In practice it is a measure of the force increment $\mathrm{\Delta }F$ acting on the elastic body under the influence of the individual absolute strain $\mathrm{\Delta }l$:

Dynamic stiffness factor $k$ is determined, directly from the graph of the hysteresis loop in the coordinate system of the force $F$ and the absolute deformation $\mathrm{\Delta }l$, while the modulus of elasticity $E$ is determined from the same graph of hysteresis, but in a coordinate system stress $\sigma$ and the strain $\epsilon$. The coefficient $k$ depends on the geometrical dimensions of the test object and whenever they change requires the calculation of new values of $k$. Thus, in contrast to the Young’s modulus $E$ stiffness ratio $k$ is not a material constant.