Comparing the performance of suspension system of semi-trailer truck with two air suspension systems

2.1. Full vehicle dynamic model

A 5-axle semi-trailer truck with a dependent suspension system for the front axle and a walking beam suspension system for the rear axles are developed for comparing the performance of suspension system. A semi-trailer truck dynamic model with 14 degrees of freedom is established for comparing the performance of the traditional and new air suspension systems, as shown in Fig. 1(a), 1(b). Dynamic modes of two different types of the air suspension systems including a tradition dynamic model and new dynamic model for the second axle and the third axle of semi-trailer truck is respectively established, as shown in Fig. 1(c), 1(e) and the new air spring model is shown in Fig. 1(d).

Fig. 1Vehicle and air suspension system dynamic model

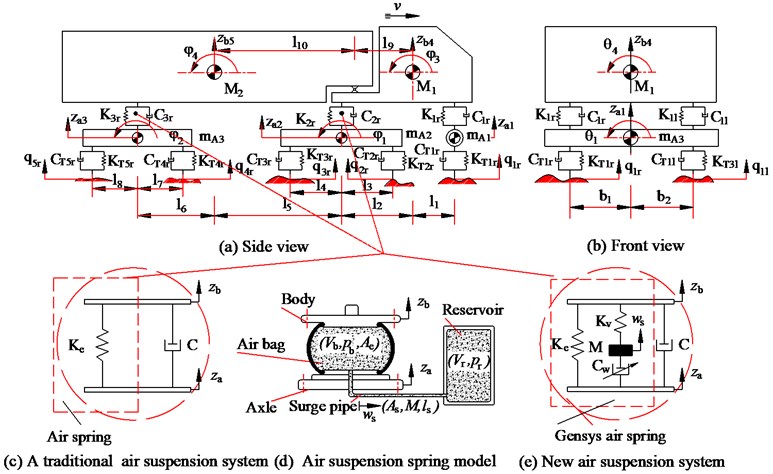
In Fig. 1, $K_{ij}$ are the suspension stiffness coefficients; $C_{ij}$ are the suspension damping coefficients; $K_{Tkj}$ are the stiffness coefficients of tires; $C_{Tkj}$ are the damping coefficients of tires; $M_1$ and $M_2$ are the sprung mass of the tractor and trailer, respectively; $m_{Ai}$ are the unsprung mass of the front axles, respectively; $l_n$ and $b_m$ are the distances; $z_{ai}$ and $z_{bm}$ are the vertical displacements at the centre gravity of the axles tractor and trailer; ${\theta }_k$ are the angle deflection at the centre gravity of the axles, tractor and trailer; ${\phi }_h$ are the angle deflection at the centre gravity of tractor and trailer; $v$ is the speed of vehicle; $K_e$ is the air spring stiffness coefficient; $C$ is the passive damping coefficient; $C_w$ is the nonlinear viscous damper of the air spring; $K_v$ is the viscous stiffness constants; $M$ is the mass of the surge pipe; $A_e$ is the effective area of air bag, $p_b$ is the pressure in air bag; $A_s$ is the cross section area of the pipeline, $w_s$ is the displacement of air in surge pipe; $l_s$ is the length of the surge pipe; $V_b$ is the volume of air bag. $V_r$ is the volume of reservoir ($i=$1, 2, 3; $j=r$, $l$; $k=$1, 2,..., 5; $n=$1, 2,..., 10; $m=$1, 2; $h=$1, 2, 3, 4).

In order to facilitate the description of vehicle vibration systems using computer simulation, a combined method of the multi-body system theory and D’Alembert’s principle is chosen in this study. The general dynamic differential equation for 3-axle semi-trailer truck is given by the following matrix form:

where $M$, $C$, $K$, $C_T$ and $K_T$ are the mass matrix of the vehicle, damping matrix of the suspension system, stiffness matrix of the suspension system, damping matrix of the wheel system and stiffness matrix of the wheel system, respectively; $z$ is the vector of displacement; $Q$ is the vector of excitation of road surface.

2.2. Air suspension system dynamic model

1) Traditional dynamic model. The air suspension dynamic model has been proposed by many researchers such as Nishimura, VAMPIRE, SIPPAC. In this study, the dynamic model of the traditional air suspension system is shown in Fig. 1(c). The vertical force of the air suspension is defined as:

where, the air spring stiffness coefficient $K_e$ is determined based on the laws of thermodynamics method [3] and the air spring stiffness coefficient is developed a new method based on the variation of the volume, area, and other structural parameters of the air spring. Therefore, the interior air pressure of the air spring is also changed to calculate the air spring stiffness. The air spring stiffness can be obtained by the derivative of ratio elastic force and displacement of the air spring, as follows:

The effective volume and area are defined as:

Based on the laws of thermodynamics [6], if the compression or the expansion stroke of the air spring is rapid enough, it can be regarded as an adiabatic process. Thus, the air state of the air spring can be defined as:

where, $V_0$, $A_0$ are the initial effective volume and area; ${\alpha }_1$ and ${\alpha }_2$ are the change of the effective volume and area with respect to $z$; $p_a$ is the standard atmospheric pressure (0.1 MPa), $n$ is specific heat ratio ($n=$1.33). Substituting Eqs. (4), (5) into Eq. (3) and apply the Eq. (3) for the stiffness of air springs.

2) New dynamic model. In this study, the new air spring model is proposed by GENSYS [5] and new dynamic model of air suspension system is shown in Fig. 1(e). The vertical force of the air suspension is defined as:

where, the static, viscous stiffness constants ($K_e$, $K_v$) and the mass ($M$) can be respectively written by:

where $\lambda$ is the polytropic rate (1 $<\lambda <$ 1.4); $p_0$ is the initial pressure in air bag; $\rho$ is the air density; $V_{b0}$ and $V_{r0}$are the initial volume of air bag and reservoir

The nonlinear viscous damper $C_w$ is related to the velocity over the damper $C_w$ and not related to the velocity in the surge pipe [7]. Therefore, the vertical viscous force is expressed as:

The Eq. (8) can be rewritten as:

The relationship between the nonlinear damping $C_w$ and the damping $C_s$ caused by the flow in the surge pipe is:

where $C_{st}$ is total loss coefficient, $C_{fr}$ is the loss coefficient due to friction, $C_{en}$ is the loss coefficient due to enlargement, $C_c$ is the loss coefficient due to contraction and $C_b$ is the loss coefficient due to bends in the pipe, respectively.

2.3. Road surface roughness

The random excitation of road surface roughness can be represented with a periodic modulated random process. The general form of the displacement PSD of the road surface roughness is determined by the experimental formula [7]:

where space frequency $n$ is the reciprocal of the wavelength $\lambda$. It means wave numbers in a meter. $n_0$ is reference space frequency, it’s defined as 0.1 m^-1. $S_q\left(n\right)$ is PSD of road surface under the reference space frequency $n_0$ known as the road surface roughness coefficient and $\omega$ is the frequency index which decides the frequency configuration of PSD of road surface $(\omega =\text{2})$.

The road surface roughness is assumed to be a zero-mean stationary Gaussian random process. It can be generated through an inverse Fourier transformation:

where ${\varphi }_i$ is random phase uniformly distributed from 0 to 2$\pi$.

In this study, typical road surface roughness is adopted according to the standard ISO 8068 [8].

4.1. Speed conditions

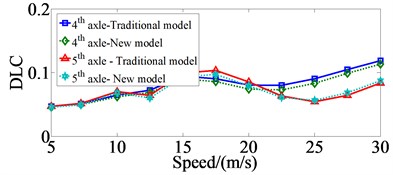
The vehicle speeds of 5 m/s, 7.5 m/s, 10 m/s, 12.5 m/s, 15 m/s, 17.5 m/s, 20 m/s, 22.5 m/s, 25 m/s, 27.5 m/s and 30 m/s were considered to compare the performance of two different types of the air suspension systems for reducing the negative impacts on the road surface when vehicle moves on the ISO level B road surface and full loaded. The DLC values at 2nd, 3rd and 4th, 5th axles of vehicle with the traditional and new dynamic models of the air suspension systems are shown in Fig. 2 and Fig. 3.

Fig. 2-3 show that the DLC values of wheels at 2nd, 3rd and 4th, 5th axles of vehicle with the new air suspension system are negligibly reduced at low- and high-speed conditions and which it is significantly reduced at average speed conditions, especially reduced by 9.3 %, 7.8 %, 7.0 % and 5.4 % at the vehicle speeds of 15 m/s, 17.5 m/s, 20 m/s and 22.5 m/s in comparison with the traditional air suspension system.

Fig. 2Comparing of the DLC values at 2nd and 3rd axles with traditional dynamic model and new dynamic model

Fig. 3Comparing of the DLC values at 4th and 5th axles with traditional dynamic model and new dynamic model

4.2. Road surface conditions

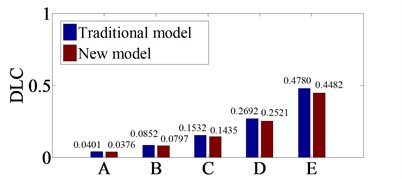
Five road surface conditions from level A (very good) to level E (very poor) in ISO/TC 80686 are used to compare the performance of two different types of the air suspension systems for reducing the negative impacts on the road surface when vehicle moves with a velocity of 20 m/s and fully loaded. The DLC values at 3rd and 5th axles of vehicle with the traditional and new dynamic models of the air suspension systems when vehicle moves on five different road surface conditions are shown in Fig. 4 and Fig. 5.

Fig. 4-5 show that the DLC values of wheels at 3rd and 5th axles of vehicle with the new air suspension system are reduced by 6.7 %, 7.0 %, 7.4 %, 7.7 %, 8.5 % and 6.6 %, 6.9 %, 6.8 %, 6.9 %, 6.6 %, respectively in comparison with the traditional air suspension system, when vehicle moves on the change of road surface condition from the ISO level A to level E road surface with a velocity of 20 m/s and fully loaded.

Fig. 4Comparing of the DLC values at 3rd axle with traditional and new dynamic model

Fig. 5Comparing of the DLC values at 5rd axle with traditional and new dynamic model