Research on the influence of underwater vehicle double shell spacing on vibration and sound radiation

1. Introduction

At present, the research on different vibration and sound radiation problems caused by single-shell structure and double-shell structure at home and abroad is deeply studied. Qi Libo [1] described underwater cylindrical shell under the influence of low-frequency sound radiation by the excitation force and characteristics. Xia Qiqiang [2] realized the structural acoustical design of double-shell by studying and changing the transmission angle of the vibration between the inner and outer layers. Jin Guangwen [3] compared the sound radiation of single-shell structure and double-shell structure. The result shows that different ribs spacing and shell length and thickness under different parameters, and single-shell structure and double-shell structure have their own vibration mean square acceleration. Tang Junjuan [4] and Li Bing [5] researched the response of radiation of double-shell structure under different excitation modes, but in the excitation mode, it is not considered under the action of tail-paddle excitation.

Aiming at the problem of underwater vibration sound radiation with complex structure and multi-source, it is very difficult to solve underwater acoustic radiation problem only by analytic method. Wu You-sheng [6] put forward the theory of three-dimensional hydro-elasticity based on the assumption that water is an incompressible medium and apply the related theories of structural mechanics to the three-dimensional structure. Zou Mingsong [7] proposed a method for calculating the near-field sound pressure and far-field acoustic radiation of underwater elastomers according to the theory of hydro-elasticity mechanics. Simultaneously, the accuracy and validity of the simulation method were verified by the model Developed three-dimensional water-elastic acoustic simulation analysis software Thafts-acoustic.

Since the double-shell structure inside and outside the shell coupled to each other, the impact of sound from the inner shell spacing is complex. In this paper, based on the internal and external structures of underwater vehicle, a simplified model of the propeller-shaft-shell with double-shell is established. At the same time, Thafts-acoustic, a hydro-elastic acoustic software is used. The acoustic source sound power level of the double-shell structure under three different excitation sources of external fluid excitation, tail-paddle excitation and mechanical equipment excitation in the shell is calculated respectively. The acoustic source sound power level of double-shell structure under different inner-shell spacing are compared.

2. Three-dimensional hydro-elasticity theory

According to the modal superposition method, the displacement of a discrete system node of an underwater vehicle can be expressed as follows:

where $q_r$, $\left(r=\mathrm{1,2},\dots ,m\right)$ is the principal coordinate component of the $r$th order mode.

It is assumed that the shell is an elastic body, and the surrounding fluid medium belongs to the non-sticking ideal acoustic medium and is uniformly compressible. It is assumed that both the acoustic field generated by structural vibration and the perturbed flow field generated by underwater driving are slightly linear within the underwater vehicle. The velocity potential of the total flow field can be linearly superposed by the velocity of each acoustic radiation:

The acoustic velocity potential $\varphi$ satisfies the Helmholtz equation. The structural fluid wet surface boundary conditions:

where $u_r$, $v_r$, $w_r$ is the $r$th order dry modal displacement. $\stackrel{⃑}{n}$ is the unit normal vector of the wet-solid surface.

The Green function corresponding to the boundary condition in the free surface is:

where:

$k=\omega /c_0$ is the number of acoustic waves in the fluid. ($\xi$, $\eta$, $\zeta$) is the coordinates of the source point $Q$. ($x$, $y$, $z$) is the coordinates of the source point $P$.

The simple source boundary integral equation:

The acoustic-elastic coupling dynamics equation in the frequency domain is:

where, $\left\{q\right\}$ is the generalized displacement vector. $\left\{\mathrm{\Xi }\right\}$ is the generalized force vector. $\left[a\right]$, $\left[b\right]$, $\left[c\right]$ are the generalized mass matrix, the generalized damping matrix and the generalized stiffness matrix of the dry structure. $\left[A\right]$, $\left[B\right]$, $\left[C\right]$ are the additional mass matrix, the additional damping matrix and the additional coefficient of restitution force matrix of the generalized fluid.

Radiation wave pressure in the flow field is:

3. Calculation model of paddle-shaft-shell

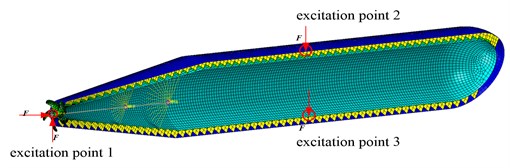
According to the internal and external structure of the underwater vehicle, using the Abaqus software a simplified model of the propeller-shaft-shell with double-shell structure was established. At the same time, the wet-surface structure was also constructed. As shown in Fig. 1, the simplified model structure mainly includes propulsion shafts, thrust bearing, double shell, solid floor, ring rib and paddle. Among them, the paddle is a five-blade paddle and is simulated by a three-dimensional element. The real rib, the ring rib and the double shell are all simulated by a two-dimensional shell element, and the shafting is simulated by a one-dimensional beam element. The simplified model includes a front bearing, a rear bearing, and a thrust bearing. All bearings are assumed to be spring units, in which the rear bearing and front bearing are simplified with horizontal and vertical bidirectional springs, while thrust bearings are simplified by a three-way spring that is horizontal, vertical, and longitudinal.

Fig. 1Calculation model

a)

b)

4. Paddle-shaft-shell calculation model

The noise source of underwater structure can be divided into hydrodynamic noise, propeller noise and mechanical noise. In this paper, three excitation locations are selected and used to simulate noise sources of three noises by applying the relevant excitation. As shown in Fig. 1, the excitation point 1 is set at the hub. The excitation point 2, is set on the outer surface of the underwater vehicle hull, for simulating the excitation of the external medium fluid. The excitation point 3, is set in the underwater vehicle shell, for simulating hull Internally generated by the mechanical equipment incentives. All the force amplitude is set to 1 N, underwater vehicle shell spacing set to $L$ ($L=$ 0.8 m). using acoustic-elastic response simulation software-Thafts-acoustic, the radiation power of double shell model is calculated in the frequency range of 10-150 Hz under different excitation, in infinite depth of the fluid medium. The radiation power is converted to sound source level by Eq. (10):

where $P$ is the radiated sound power value. Its reference value is $P_0=$ 0.65×10^-18 W/Hz.

At the excitation point 1, under the excitation of the lateral force of the paddle, the radiation source level of the double-shell paddle-shaft-shell structure with different shell spacing are shown in Fig. 2. As shown in the figure, under the horizontal excitation of the paddle, the sound radiation of the underwater structures with different spacing shell shows small difference. The total sound level of the underwater structure with the spacing of 0.5L/L/1.5L are 103.2 dB, 101 dB and 105 dB respectively in the frequency range of 10-150 Hz. Therefore, the total sound level, when the inner and the outer shell spacing is L, is slightly lower than that of the other two sizes.

At the excitation point 1, under the excitation of the longitudinal force of the paddle, the radiation source level of the double-shell paddle-shaft-shell structure with different shell spacing are shown in Fig. 3. As shown in the figure, under the longitudinal excitation of the propeller, the first peak and the second peak of the curve of the model with the shell spacing 1.5L are obviously lower than the other two curves. The total sound level of the underwater structure with the spacing of 0.5L/L/1.5L are 104.2 dB, 103 dB and 105 dB respectively in the frequency range of 10-150 Hz. Therefore, the total sound level, when the inner and the outer shell spacing is L, is slightly lower than that of the other two sizes.

Fig. 2Sound source level of different shell spacing under transverse propeller exciting

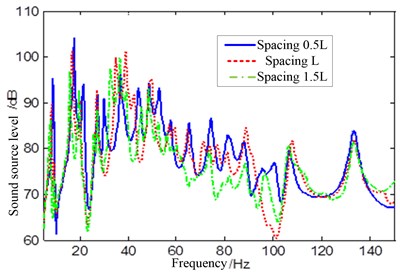
Fig. 3Sound source level of different shell spacing under longitudinal propeller exciting

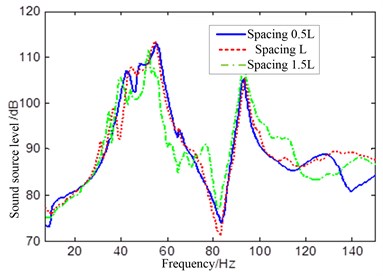
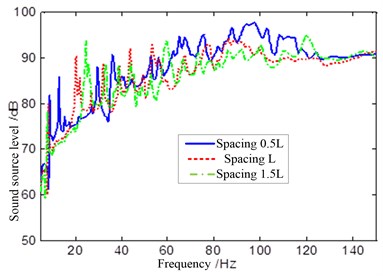
At the excitation point 2, under the excitation of the surface of the external fluid medium, the radiation source level of the double-shell paddle-shaft-shell structure with different shell spacing are shown in Fig. 4. As shown in the figure, under the excitation of the surface of the external, the low frequency band and the high frequency band of the model with the shell spacing 0.5L are obviously higher than the other two curves. The total sound level of the underwater structure with the spacing of 0.5L/L/1.5L are 101.2 dB, 103 dB, 105 dB respectively in the frequency range of 10-150 Hz. Therefore, the total sound level, when the inner and the outer shell spacing is 0.5L, is slightly lower than that of the other two sizes.

At the excitation point 3, under the excitation of the internal mechanical equipment, the radiation source level of the double-shell paddle-shaft-shell structure with different shell spacing are shown in Fig. 5. As shown in the figure, under the excitation of the internal mechanical equipment, the low frequency band and the high frequency band of the model with the shell spacing 0.5L are obviously higher than the other two curves. The total sound level of the underwater structure with the spacing of 0.5L/L/1.5L are 102.8 dB, 109 dB and 104 dB respectively in the frequency range of 10-150 Hz. Therefore, the total sound level, when the inner and the outer shell spacing is 0.5L, is slightly lower than that of the other two sizes.

Fig. 4Sound source level of different shell spacing under external fluid exciting

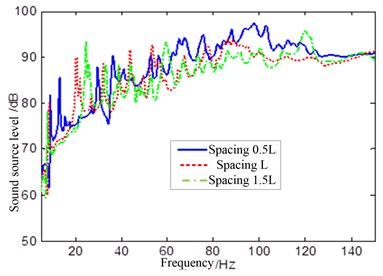
Fig. 5Sound source level of different shell spacing under internal mechanical exciting

5. Conclusions

1) Under the excitation of lateral force and longitudinal force of propeller, the radiation source level of the double-shell paddle-shaft-shell structure with different shell spacing has little difference. The total sound level, when the inner and the outer shell spacing is 0.5L, is slightly lower than that of the other two sizes.

2) Under the excitation of external medium fluid excitation force and internal mechanical excitation force, the low frequency band and the high frequency band of the model with the shell spacing 0.5L are obviously higher than the other two curves, but opposite at mid band. However, the total sound level, when the inner and the outer shell spacing is 0.5L, is slightly lower than that of the other two sizes.