Indian railway track analysis for displacement and vibration pattern estimation

2.1. Beam on viscoelastic foundation (BOVF)

The beam on viscoelastic foundation is considered. A spring-damper element COMBIN40 is attached to a 15 m long beam (Beam element: BEAM188) in vertical direction ($Z$-axis). The ground nodes of foundation are constrained for all degree of freedom. Beam element is 0.1 m long and spring damper is attached at extremities of the beam elements.

The governing equation of motion for a Euler- Bernoulli beam on viscoelastic foundation can be given by Eq. (1), (Mallik et al. [8]):

In case of moving load:

where, $E$ is the Young’s modulus of elasticity of the beam material, $I$ is the second moment of area of the beam cross-section about its neutral axis, $\rho$ is the mass per unit length of beam, $c$ is the coefficient of viscous damping per unit length, $k$ is spring constant of foundation per unit length, $F\left(x,t\right)$ is the applied moving point load per unit length, $x$ is the space coordinate measured along the length of beam, $t$ is the time in seconds, $v$ is the constant speed of point load and $\delta$ is the Dirac delta function.

Fig. 1Beam elements on viscoelastic foundation

Consider an element $ij$ of length 2$a$, having a uniform cross section as shown in Fig. 2. To generalize the equation of motion for the track finite element model may be written as:

where $M$, $C$ and $K$ are the global mass, damping and stiffness matrix for the finite element model and $\left\{z\right\}$ is the vector of vertical displacement of the model. $\left\{P\right\}$ is a vector of force and moments acting on the nodes of the model. The mass matrixand stiffness matrixof the beam elements is determined using Euler-Bernoulli beam theory as described by Petyt [15].

2.2. Beam on discrete elastic support

Fig. 2 shows a 2D model of beam on discrete elastic support. Rail is modeled as Euler-Bernoulli beam supported by 6 mm rail pad which act as massless spring and damper between rail and sleeper. Sleeper is considered as concentrated mass and ballast as spring- damper of 0.2 m depth. The sub-grade layer is neglected. The track is modeled as beam element (BEAM 188) of length 0.6 m. The mass and stiffness matrix of rail is same as the above. The mass, stiffness and damping matrix for a rail pad element is described by Croft [13].

Each rail pad element connects a rail node and sleeper node. The degree of freedom of the model allows vertical displacement and rotation of half sleeper mass and vertical displacement of ballast layer.

The ballast is modeled as massless spring and damper. Any damping in the rotational degree of freedom due to the ballast layer is neglected. The ground nodes of ballast layer are constrained for any displacement and rotation. The mass, stiffness and damping matrix for the foundation is described by Croft [13].

Fig. 2Beam on discrete elastic support

3.1. Comparison of models for vertical displacement at different speed

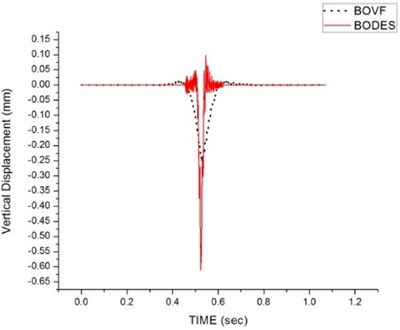
Computational analyses are carried out for the models described in section 2.1 and 2.2. The dynamic response in term of vertical displacement and acceleration is obtained with respect to time and the position of the beam. The input parameters are as per the Indian railways standard. Fig. 3 shows the vertical displacement response at the midpoint for beam on viscoelastic foundation and discrete elastic support at different velocities. The maximum deflection for beam on viscoelastic foundation is 0.25 mm for 50 and 250 km/h. However, the maximum deflection for beam on discrete elastic support is 0.6 mm for 50 and 250 km/h. The maximum deflection is higher in case of beam on discrete elastic support, which is attributed to the fact that elastic support is provided at every 0.6 m from left end of beam whereas for viscoeleatic foundation, elastic support is at every 0.1 m.

The result also shows that the influence of the travelling speed is quite insignificant on the vertical displacement of relatively larger foundation stiffness. The negative value of deflection signifies the downward deflection with the moving load. A small amount of positive deflection occurs in distance away from the load. The positive deflection is about 0.05, 0.07 and 0.1 mm for discrete elastic support shows that with increase in speed, upward deflection and vibration of track increases, which may lead to dynamic instability for the moving train and deterioration of the track life. Such variation in upward deflection at different speed is not seen for beam on viscoelastic foundation.

Fig. 3Comparison of deflection for beam on viscoelastic foundation and discrete elastic support: a) 50 km/h, b) 250 km/h

a)

b)

3.2. Comparison of both models for vertical acceleration at different speed

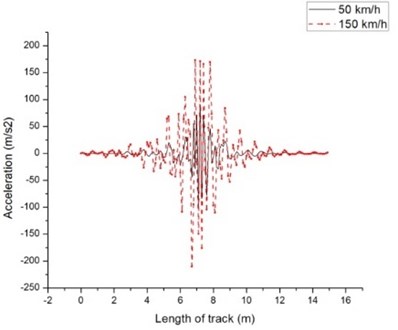
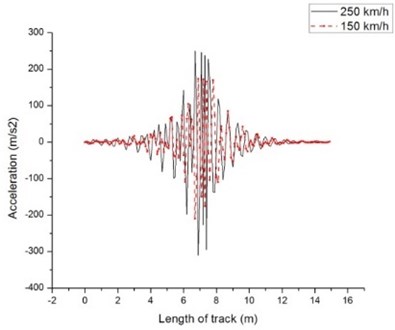
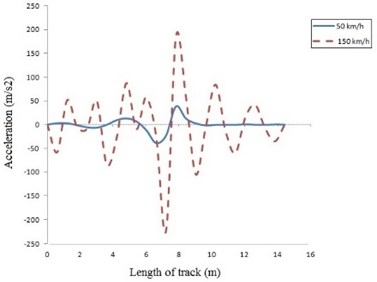
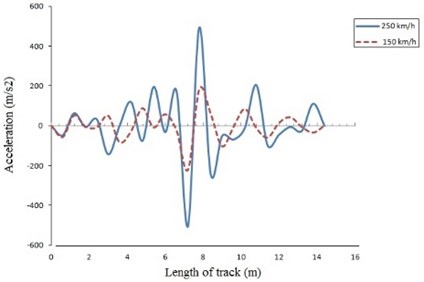
The variation of vertical acceleration at the midpoint of the track for both models at different speeds is shown in Fig. 4 and 5. Similar to deflection response, the maximum acceleration is calculated for the midpoint of the beam. The maximum acceleration for beam on viscoelastic foundation is –102.92, –210.2 and –310 m/s² at 50,150 and 250 km/h respectively. The maximum acceleration for the discrete elastic support is –38.5, –223.2 and –504.5 m/s² at 50, 150 and 250 m/s² respectively.

The acceleration for discrete elastic support is higher than the beam on viscoelastic foundation. For beam on viscoelastic foundation, acceleration away from the midpoint can be neglected, but for discrete elastic support it accelerates significantly. The stability of the foundation depends upon the acceleration of the track and for given Indian railways standard, it is not suitable to go beyond 250 km/h.

The change in acceleration may attenuate the rail pad and ballast stiffness and also the dynamic stability of the rail. High speed trains can be achieved by proper maintenance of track, by changing the level of ballast foundation and by introducing new higher stiffness rail pad.

Fig. 4Acceleration for beam on viscoelastic foundation at: a) 50 and 150 km/h, b) 150 and 250 km/h

a)

b)

Fig. 5Acceleration of beam on discrete elastic support at: a) 50 and 150 km/h, b) 150 and 250 km/h

a)

b)