Modelling the powertrain rubber coupling under dynamic conditions

3.1. Experimental determination of static properties of rubber element

To determine the characteristics of the entire coupling in a given configuration requires analysis of static properties of a single rubber element in dependence on deformation, temperature and frequency. The design of the current coupling includes eight individual rubber elements embedded into the steel disc, see Fig. 1. These elements allow the formation of various configurations of couplings with defined properties.

Fig. 1Elastic coupling connecting engine and electric generator

The nonlinear behavior of the rubber elements of the coupling has to be included in the model. Rubber element nonlinearities, due to amplitude dependence, are discussed by Harris and Stevenson [9]. These impacts can be caused by the geometrical design of the rubber component, as well as, by the intrinsic material behavior. The references present nonlinear stress of the material – strain relations for finite strain ranging approximately from 20 to 500 %. Components also show nonlinear properties for small to intermediate amplitudes – ranging approximately up to 5 % of component strain due to the material behavior.

Rubber element properties are analyzed separately by technical experiments and computational models. Subsequently, the whole coupling properties are derived. Static deformation properties of rubber element assume the following:

• Deformation in the radial direction (corresponding to the load of the element perpendicular to the axis of the element).

• Deformation in the axial direction (corresponding to the load of the element in the axis of the element).

• Angular deformation perpendicular to the axis of the element (corresponding to the moment perpendicular to the axis of the element).

A determination of these components is carried out employing experimental procedure for computational model calibrations and using computational procedure for radial, axial and angular directions. The results of the radial loading are based on basic technical experiments which are used to tune computational finite element (FE) model properties.

The static characteristics of the rubber element are determined by using the universal testing machine, see Fig. 2.

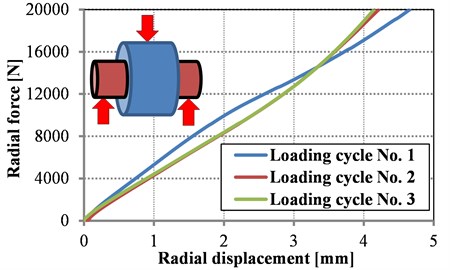
The radial loading characteristics of the rubber element is presented in Fig. 3. It shows three repetitions of loading, the first cycle is slightly different due to element positioning, therefore this case is not assessed.

Fig. 2Arrangement of a technical experiment to determine the static characteristics of the rubber element

Fig. 3Measured force vs. radial deformation

3.2. Computational determination of static properties of rubber element

It is not possible to measure all the necessary elastic properties of a rubber element. However, there is a way how to obtain all the properties to use computational determinations of static properties of rubber elements based on the selected experimental results. The experimental results are used to tune the computational model of the rubber element. The computational model is solved by entering the displacement in a given direction and by evaluation of the overall force required for implementation of the displacement.

The computational determination of the deformation properties of the rubber element is performed using the finite element method (FEM). Mooney-Rivlin’s two-parameter hyperelastic model is used as rubber material model. The Mooney-Rivlin’s parameters taken from measured results presented in Fig. 3. The resultant material constants characterizing the deformation of the deviatoric stress are $c_{10}=$ 1.11 and $c_{01}=$ 0.27 and incompressibility parameter is $d=$ 0.018.

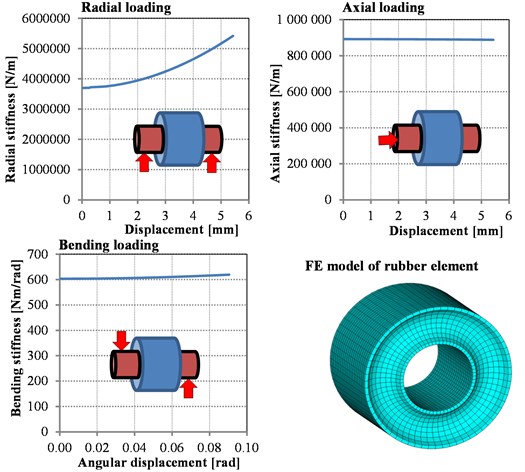
The calculation results, including the introduction of the FE model, are presented in Fig. 4.

Fig. 4Calculated radial, axial and bending stiffness of single rubber element and FE mesh of computational model

Nonlinear deformation behavior of the rubber element is considered only in the radial direction and it can be approximated as:

where $c_R$ is radial stiffness for current radial loading, $c_{R0}$ is radial stiffness for no loaded state and $x_R$ radial deformation of the rubber element center under current loading. Current radial stiffness can be evaluated as:

where $F_R$ is a current radial force.

Axial stiffness $c_A$ and bending stiffness $c_b$ of the rubber element are considered as constant with respect to the values of the axial forces or bending moments. The nonlinear deformation behavior is not presumed.

3.3. Experimental determination of dynamic characteristics of rubber element

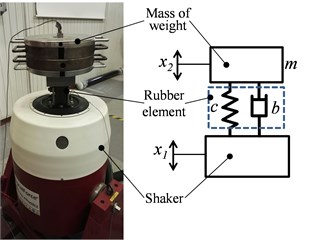
Material components made of rubber present a significant dependence on the loading frequency. These frequency dependent properties are determined experimentally by a vibration exciter device. The assembly of the technical experiment to determine the dynamic characteristics of rubber element, is presented in Fig. 5.

The technical experiment is carried out in above-resonance area of the system consisting of the rubber element and a weight. The following parameters are evaluated:

radial dynamic stiffness $c_R$ of rubber element and relative damping $\xi$ of rubber.

The description of the experimental arrangement used for measurement can be simplified to a system with one degree of freedom. Therefore, the equation of the motion, of a system presuming only harmonic loading, is:

where $x_1$_is a defined time dependent displacement of the vibration excitation, $x_2$ is a response of weight mass $m$, $b_R$ is radial viscous damping coefficient of a rubber element, $c_R$ is radial stiffness of a rubber element, $\omega$ is angular velocity and $t$ is time.

Fig. 5Arrangement of technical experiment to determine the dynamic characteristics of the rubber element

The system can be solved by introducing complex variables. Then the complex dynamic stiffness of the rubber element is defined as:

The quantities with vinculum are complex; therefore, the rubber element radial stiffness can be calculated as:

And radial damping coefficient of the rubber element is:

Relative damping is believed to be independent of the excitation frequency:

Measuring and evaluating the radial stiffness and the relative damping is performed with the excitation frequency ranging from 50 Hz to 300 Hz and for temperatures from 25 °C to 55 °C. The measured stiffness dependent on excitation frequency, is expressed in the form of frequency correction coefficients. These correction coefficients are defined as:

where ${\alpha }_f$ is a frequency correction coefficient for stiffness and ${\alpha }_{f\xi }$ is a frequency correction coefficient for relative damping. The measured frequency correction coefficient for stiffness is presented in Fig. 6.

The measured frequency correction coefficient for stiffness can be approximated by second order polynomial as:

And measured frequency correction coefficient for relative damping is a constant equal to 1.

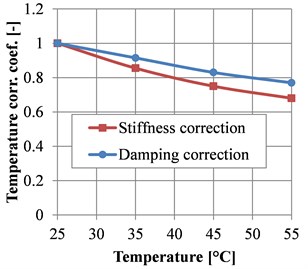
The influence of temperature can be expressed by temperature correction coefficient for stiffness ${\alpha }_{Tc}$ and relative damping ${\alpha }_{T\xi }\text{.}$ The measured values of temperature correction coefficients are presented in Fig. 7.

The values of the temperature correction coefficients for stiffness and relative damping can be expressed by second order polynomial as:

Fig. 6Measured frequency correction coefficient for stiffness

Fig. 7Measured temperature correction coefficient for stiffness and relative damping

3.4. Determination of dynamic nonlinear characteristics of elastic coupling

The resultant static torsional stiffness of the whole elastic coupling can be calculated as follows:

where $D$ is a pitch diameter of rubber elements, $n_1$ is a number of rubber elements on one side and $n$ is a total number of rubber elements.

The resulting dependency of coupling dynamic stiffness on the loading frequency and element temperature can be written as:

where $c_{Tstat}$ is static coupling stiffness at temperature of 25 °C and under static load. Relation of viscous damping coefficient $b_T$, relative damping ${\xi }_T$ and frequency $\omega$ is:

Relative damping dependent on temperature can be expressed as:

where ${\xi }_0$is relative damping at temperature of 25 °C. It is supposed that the dependence of relative damping on the loading frequency is minimal; and therefore, the frequency dependence is not included in the relative damping Eq. (15).

The coupling model is extended by coupling flexibility for load in axial direction and for bending moments. These properties are considered as independent of the deformation. The bending stiffness of the whole coupling can be approximated as:

And coupling bending damping reads:

Similarly, the axial stiffness of the whole coupling is defined as:

And the axial damping can be calculated as:

where $v$ is axial deformation velocity.

4.1. Implementation of computational models

The computational model is solved in time domain for steady state conditions. Model of crank train and generator system, including the elastic coupling assembled in multibody system, is shown in Fig. 8. Full description of crank train computational models are presented in Novotny [6].

The computational model of the elastic coupling comprises of two bodies (discs) connected by nonlinear general forces enabling flexibility for torsional, bending and axial loading. The two coupling bodies are constrained by primitive joint enabling one axial translational movement and three rotational movements. The computational model of crank train is excited by gas forces in combustion chambers considering operational states of the ICE.

The computations of the engine-generator system are done for steady states starting from engine speed 400 rpm to engine speed 2000 rpm with step of 100 rpm.

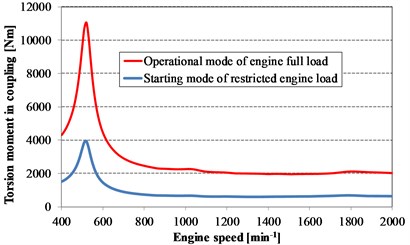
The computed results presented in Fig. 9 show torsional resonance of the 3rd order near the engine speed $n=$ 520 rpm, this engine speed is highly critical; however, the resonance occurs below engine operating speed range.

Operating speeds show no torsional resonances of the engine-generator system. In general, a lack of resonances near the operating engine speeds is critical in terms of the coupling design.

Fig. 8Model of crank train and generator system including elastic coupling assembled in multibody system

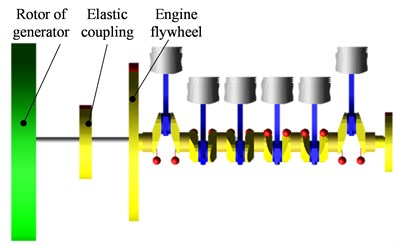
Fig. 9Computed torsional moments in the elastic coupling for engine speeds including starting and operational engine modes

4.2. Experimental verification of computational models

Technical experiments (see Fig. 10) are proposed to verify the final design of the elastic coupling, as well as, to validate computational models. The verification of the coupling design is performed in the engine operating speed range under full engine load conditions. Resonant speed of the engine-generator system cannot be directly verified because in such low speeds, below idling speed, the engine does not operate.

Fig. 10Measurement of crankshaft pulley angular vibrations

Fig. 11Dominant harmonic orders vs. engine speed for computed (“Comp.”) and measured (“Exp.”) angular displacements

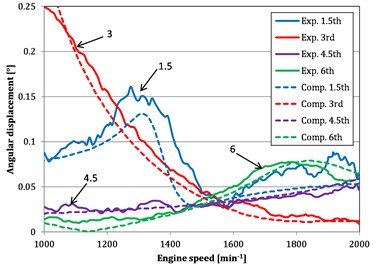
Dominant harmonic orders vs. engine speed for computed and measured angular displacements of flywheel pulley are presented in Fig. 11. The 3rd harmonic order is closely connected with vibration of ICE and the electric generator system. The coupling is always designed to avoid resonances in operational engine speeds.