Flow behaviour on aerofoils using CFD

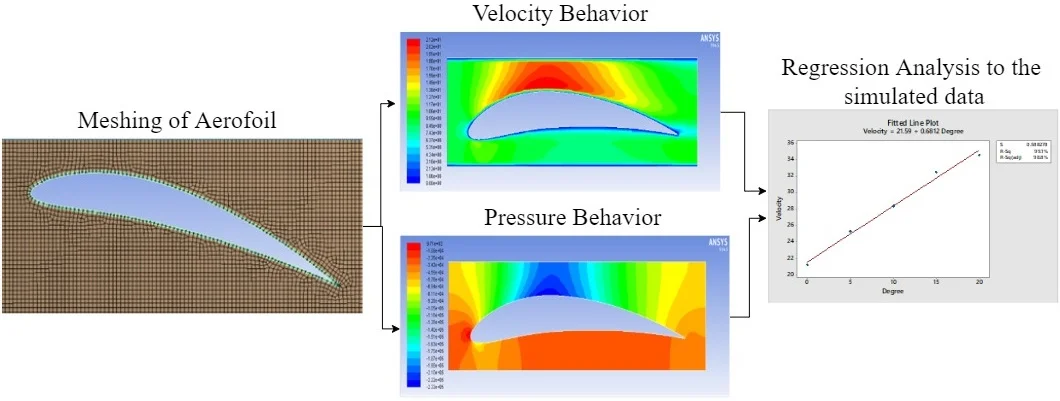
1. Introduction

A steam turbine is an extended form of a heat engine which drives most of its performance from the thermodynamic efficiency from different stages of steam generation. The steam turbine blades are aerofoils which are light in weight, durable in nature, provide a better performance, efficient in working, and many other factors can be counted on [10]. The aerofoils move through the fluid and generate an aerodynamic force which provides a characteristic shape to work with more efficiency. The CFD analysis has provided the viability of simulating for the required contours regarding the flow behaviour. The CFD study over the aerofoils has been exaggerated rapidly with vast development. Naga babu et al. [1] carried out a CFD study for analysing the efficiency of the utility steam turbine, which involves the behaviour of the flow path in various components of the turbine. The overall efficiency of the turbine was increased by modifying the selected aerofoil properties. Sivakumar et al. [2] carried out a CFD study for analysing the efficiency of the utility steam turbine. The turbine geometries are analyzed for the parameters like temperature, pressure and power output. The obtained results have helped in predicting the turbine performance. Ali Raza Abid et al. [3] analyzed the turbine blades prepared from GE with twisted geometry along its length and applied with reverse engineering techniques. The blades were analyzed in under the wind tunnel conditions at lower velocities to reduce the vibrational and blade stall effects. On comparing the results, on comparing the results, variations have been identified as the ideal conditions cannot be maintained when it’s done experimentally. Sivakumar et al. [4] carried out the CFD study of turbine blades. In this, the study was conducted on a typical intermediate pressure cylinder. On evaluation and comparison among the CFD results and 2D experimental values, it is brief that the flow and performance of the equipment affect the velocity pressure and temperature lineation. The coefficient of lift/drag increases up to some extent of the angle of attack and after decreases. The coefficient of lift and coefficient of drag are the parameters that describe the performance of an aerofoil [5-7]. According to Bernoulli's principle, the pressure experienced by the lower surface is higher than the upper surface; then it can cause lift effectively [8-9]. Juliana et al. [10] worked on the performance of the wind turbine using a 2D model of NACA 4420 aerofoil with modifications in an angle of attack from 3° to 10° at the inlet of the geometry. They found the best results at an angle of 4o with maximum $L$/$D$ ratio, which ultimately contributed to the performance of the turbine.

The conception of the blade in the turbine is considered to be the critical module in terms of the performance. The velocity when increased develops a force along the surface, which modulates the flow behaviour and depicts a more significant impact over the aerofoil geometry. Due to this, the pressure lineation gets reduced and contributes to increasing the performance of the aerofoil ultimately. The primary grail of the work is to fractionate the discernment of the flow along the surfaces of an aerofoil and also to evaluate the velocity and pressure lineation concerning the design of the geometry.

2. Detailed procedure

The aerofoil geometries are of various series depending upon the height of the upper chamber and lower chamber. Based on the respective conditions, the aerofoil geometries are considered in three series, i.e. 6412, 7412, 8412, with a total number of 81 plot points [11, 12]. The aerofoil have been a reliable source for an airstream inside the geometry to develop the aerodynamic force [8]. The change in the angle of attack at the inlet of the aerofoil geometry will take a considerable effect on the potential of the turbine [10]. The change in the angle of attack or the angle of inclination is to increase the lift to drag ratio, which ultimately affects the efficiency of the aerofoil attached and also contribute to the potential of the turbine [10]. On comparing the three aerofoil series in terms of the chord length, the curvature of the aerofoil, thickness in terms of the upper chamber and lower chamber, smoothness of the leading edge for the flow [20, 21]; the aerofoil geometry with 8412 series was considered to be the best one and was proceeded with further conceptions and examinations.

The geometries are developed from the plot points generated using Auto CAD software. These plotted geometries are imported to ANSYS Fluent software to analyze for the velocity lineation and pressure lineation. The considered geometries are pertained with the energy equation, $k$ – epsilon equation with different inlet velocities to solve for the required conditions. The geometries concerning the 8412 series are placed at various inclination angles namely 0°, 5°, 10°, 15°, 20°. The inlet velocity has been kept constant at 9 m/s for all the models. These different geometries are named as mentioned in the Table 1 so as for the convenience in the successive discussions.

The considered geometries are conceptualized and examined in a predefined manner as mentioned.

The geometries are applied with principles such as the continuity principle, Bernoulli’s principle and equations such as energy equation, kappa – epsilon equation. The calculation and evaluation are termed as follows.

2.1. Continuity equation

The constitute of the fluid entering the intended profile must be same as the constitute of the fluid leaving the profile:

1

$m_1=m_2,$

2

$\frac{d{m}_1}{dt}=\frac{d{m}_2}{dt},$

3

${\rho }_1{A}_1{U}_1={\rho }_2{A}_2{U}_2,$

4

$A_1{V}_1=A_2{V}_2.$

Fig. 1Reference plot of the 8412 – aerofoil

Fig. 2Conditions applied for the generation of the aerofoil

Fig. 3Mesh for the geometry of model – M4

3. Results and discussion

The principal aim of the proposed work is to investigate the flow behaviour on aerofoil-based turbine blade for different velocities and angle of attacks. The design of the aerofoil, along with the behaviour of the flow over the surface depicts a tremendous impact over the performance of the turbine. The sensitivity of the flow along the surface of the geometry and the better performance of the turbine using the aerofoil geometry as the turbine blades is to be analysed, using the CFD with modifications in terms of the angle of attack [10]. The geometries are appertained with energy equation and kappa – epsilon equation and then provided with the inlet velocity and temperatures. After providing the geometries with necessary boundary conditions, they are evaluated accordingly also studied the accuracy of the simulated values using regression analysis.

3.1. Flow behaviour over aerofoil

It is observed that the generated simulated values are increased by increasing the attack angles, and the velocity parameter also increases concerning the inlet velocities as represented in Table 2 and as shown in Fig. 4. It is clear that as inlet velocities increase the Maximum velocity also increases up to 150 angles of attack, later for 200 it drops. This pattern is noticed at velocity 10 m/s at 200 angles of attack, as shown in Table 2 and Fig. 4.

Fig. 4Velocity variations for different angles of attack

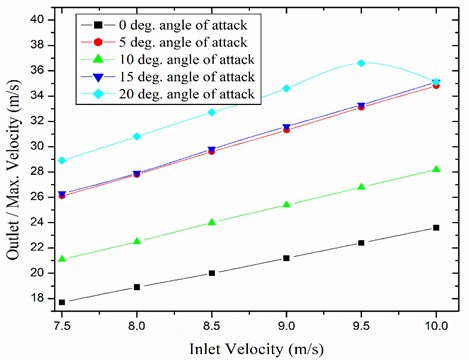
Table 2Outlet/maximum velocities in terms of angle of attack

Angle of attack	Inlet velocity (m/s)	Velocities (m/s)
		7.5	8	8.5	9	9.5	10
0°	Min. outlet velocity (m/s)	0	0	0	0	0	0
	Maximum outlet velocity (m/s)	17.7	18.9	20.0	21.2	22.4	23.6
5°	Min. outlet velocity (m/s)	0	0	0	0	0	0
	Maximum outlet velocity (m/s)	26.1	27.8	29.6	31.3	33.1	34.8
10°	Min. outlet velocity (m/s)	0	0	0	0	0	0
	Maximum outlet velocity (m/s)	21.1	22.5	24.0	25.4	26.8	28.2
15°	Min. outlet velocity (m/s)	0	0	0	0	0	0
	Maximum outlet velocity (m/s)	26.3	27.9	29.8	31.6	33.3	35.1
20°	Min. outlet velocity (m/s)	0	0	0	0	0	0
	Maximum outlet velocity (m/s)	28.9	30.8	32.7	34.6	36.6	35.1

Based on the above observations, the study is carried out for inlet velocity 9 m/s and the angle of attack for 150. Fig. 5-9 represents the velocity contours and Fig. 10-14 represents pressure contours. The more negative pressure is developed in the first region of the aerofoil. As the angle of attack increased, the negative pressure is moved towards the starting point of the aerofoil. Since the pressure and the velocity are inversely proportional to each other. The velocity is more at the top region and goes on shifting towards the starting of an aerofoil, as shown in Fig. 5-9. But for the modal M5, the zero-velocity flow is developed on the back region of the aerofoil, which was affected by the next blade, as shown in Fig. 9. Hence there is a drop in velocity parameter at 10 m/s for 200 angles of attack.

From Fig. 5-9 and table 2, we can observe the changes in the velocity parameters accordingly for each model. From the model M1 to M2, it is observing an improvement of 32.3 %. Similarly, for model M3 to M4, it is observed a rise of 19.85 %, and for model M4 to M5 it is 8.8 % increase in terms of the velocity parameters.

Fig. 5Velocity contours for the model M1

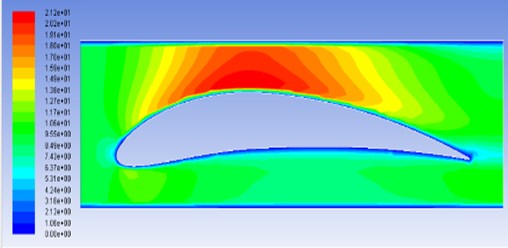
Fig. 6Velocity contours for the model M2

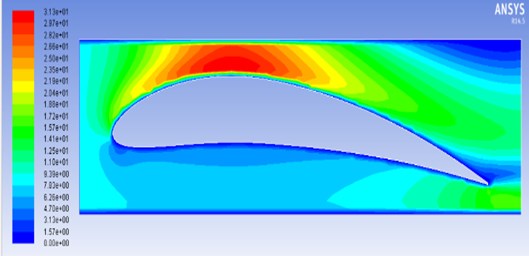
Fig. 7Velocity contours for model M3

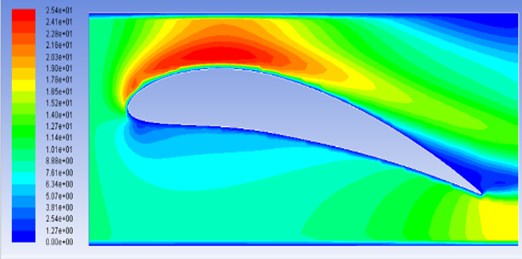
Based on the Bernoulli’s principle, the top region of the aerofoil is exposed to low pressure (negative value) and the bottom region is having the higher pressure (positive value) as shown in Figs. 10-14. Similarly, the more velocity is observed in the top region of aerofoil and bottom region with low velocity. Hence it leads to pushing the blade in the upward direction usually to the inlet flow, which affects the performance of the turbine.

Fig. 8Velocity contours for model M4

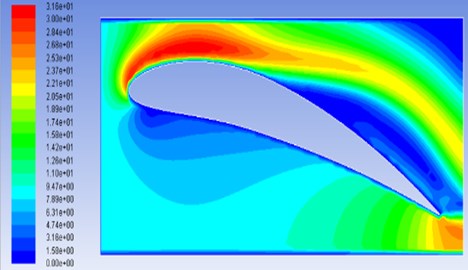
Fig. 9Velocity contours for the model M5

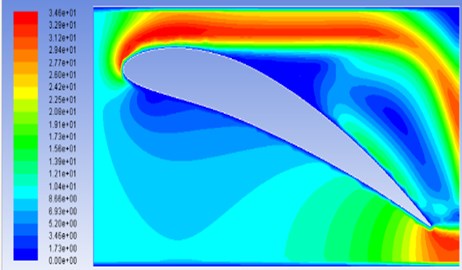
Fig. 10Pressure contours for the model M1

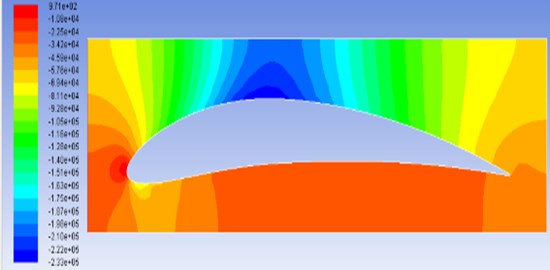
Fig. 11Pressure contours for the model M2

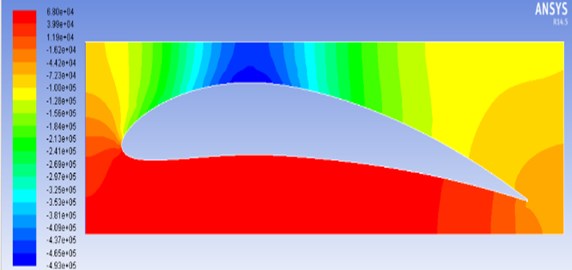
Fig. 12Pressure contours for model M3

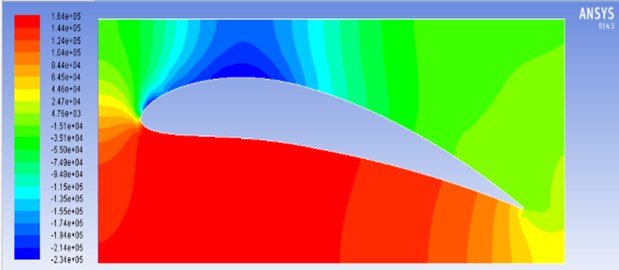
Fig. 13Pressure contours for model M4

Fig. 14Pressure contours for the model M5

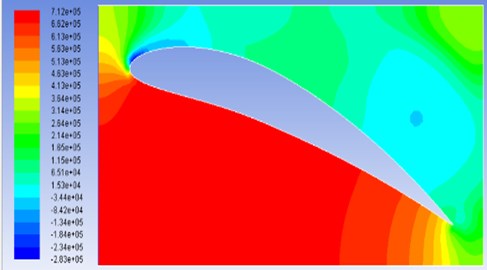
Table 3Minimum/maximum pressure in terms of angle of attack

Angle of attack	Inlet Vel. (m/s)	Pressure (pascal)
		7.5	8	8.5	9	9.5	10
0°	Min. Pressure	–161851.1	–184272.5	–208145.9	–233489.3	–260294.1	–288554.8
	Max. Pressure	718.1	798.9	884.1	971.3	1061.7	1155.8
5°	Min. Pressure	–342134.7	–389388.2	–439716.6	–493082.7	–549520.5	–609020.8
	Max. Pressure	46816.6	53429.2	60487.7	67986.2	75926.0	84320.6
10°	Min. Pressure	–162637.9	–185025.0	–208857.3	–234135.1	–260848.3	–289000.7
	Max. Pressure	113565.0	129346.8	146168.1	164021.3	182912.2	202844.3
15°	Min. Pressure	–179797.7	–204593.0	–230986.9	–258996.4	–288601.2	–319808.8
	Max. Pressure	356690.2	405963.1	458404.5	514108.4	572970.2	635032.9
20°	Min. Pressure	–196871.3	–223903.3	–252725.1	–283270.2	–315565.3	–337515.4
	Max. Pressure	495084.7	563149.9	635306.8	712057.0	793136.9	871364.8

The minimum and maximum pressure contour concerning the inlet velocity for a different angle of attack are shown in Table 3. As the inclination angle at the inlet of the geometry is increased, it leads to varying in the velocity and pressure in several means of the fluid which allowed through the geometry. From the simulated data, it is clear that the model M4 has higher rates of velocity and pressure contours when compared to the other models by 14.86 % in terms of velocity and 12.28 % in terms of the pressure. The maximum outlet velocity obtained with higher amount of difference was for the model M4, and the value was 31.5669 m/s and the pressure developed was also for the model M4, and the value was 514108.4002 Pa.

3.2. Regression analysis

To find the error for the simulated data, regression analysis is done. Base on the R2 and adj R2 value, the error can be defined as shown in Fig. 15. The experimental values close to 1, i.e. is 0.98, which indicates that the regression date is in good agreement with simulated data, as shown in table 4. The simulated values are compared to the regression values, as shown in Table 4 [13, 16]. The equation is developed based on the angle of attack and inlet velocity of the aerofoil: Final Equation for the regression Velocity = 21.59 + 0.6812 Degree.

Fig. 15Graphical plot of the angle of inclination and the outlet velocities

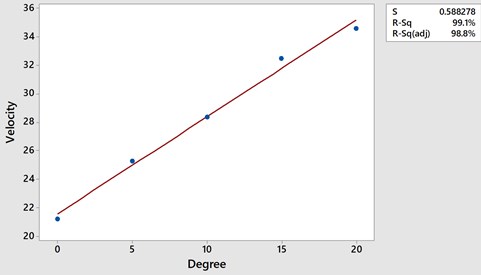
A conventional method is used to obtain the accuracy the optimum statistical methods such as the regression analysis. The regression describes the intended relationship between the dependent variable and the independent variable or other remaining variables. The simulated data is referred for generating the equation for finding the final velocities and temperature contours for the considered models with different angles of inclination. An error if any, can be identified by replacing the simulated values in the below equation. On comparing the generated data from the simulation to the regression data generated from the data given, the accurate final velocity and temperature co-efficient can be defined [13-18].

Table 4Comparison plot between the simulated data and the regression data

Regression values
Degree	Software data	Regression data
0	21.2218	21.2218
5	25.28092	31.31092
10	28.37354	25.37354
15	32.5069	31.5669
20	34.63762	34.63762

4. Conclusions

The work aims to analyse the flow behaviour along the surface of the aerofoil in terms of velocity and pressure lineation. And also, to find the optimum angle of inclination for the better performance of the turbine with considerable lineation. The aerofoil geometry with an angle of 15° inclination has been depicted as the best one with maximum deviation in the respective lineation. The aerofoil geometry, when placed at this particular angle of inclination and with a peculiar inlet velocity, leads to an increase in the efficiency and performance of the turbine. The generated results when compared to the regression analysis found to correlate in good agreement with each other.