Research on improvement of Terzaghi’s bearing capacity equation for coral sand foundation

2.1. Test program

Based on a container terminal project in East Africa, the strata disclosed at the site of geotechnical investigation are mainly coral reef limestone and its debris formed by biochemical sedimentation, as shown in Fig. 1. According to the geological survey and SPT, the SPT blow count of the surface coral reef backfill is about 8 to 12; the second stratum, consisting of 2 to 8 m thick undisturbed coral reef limestone residual soil, has an SPT blow count of about 18, and a local SPT blow count of less than 8. The stratum downward is strongly weathered coral reef limestone, with an SPT blow count of about 30. The coral reef backfill is bulky and powdery granular soil. The foundation composed of such type of soil is generally treated through vibration rolling, dynamic compaction, etc.

Fig. 1Core sample of coral reef sandy soil foundation

The coral reef backfill was treated by dynamic compaction and vibration rolling of different energy levels, and shallow plate loading tests were conducted on the coral reef sandy soil before and after reinforcement, as shown in Fig. 2 and Fig. 3.

Fig. 2Field plate loading test

Fig. 3Plate Size 0.5×0.5 m

The tests were conducted according to the requirements, procedures and machinery of the loading test as specified in the 2018 edition of Code for Investigation of Geotechnical Engineering [11]. The test site was divided into four test zones, i.e. vibration rolling zone, and 500 kJ, 1000 kJ and 3000 kJ dynamic compaction zones and in each zone, eight points were selected. For further determination of the effect of reinforcement, the plate loading tests were conducted in the test zones respectively before and after the reinforcement. During the test, the measuring points were arranged in the center of the vibration rolling zone and between the rammers in the dynamic compaction test zones. The deformation modulus was calculated by using Eq. (1) as follows:

where $E_0$ is the deformation modulus (MPa); $I_0$ is the shape factor of the loading plate (0.886 for a square loading plate); $P$ is the total load (kPa); $s$ is the settlement (mm) under the effect of total load $P$ imposed on the plate; $d$ is the side length of the plate (0.50 m here); $\mu$ is the Poisson’s ratio (0.27 for gravelly soil and 0.30 for sandy soil).

The 0.5 m×0.5 m plate used in the field loading test was loaded in levels according to the actual situation, and a load of 100 kPa was added at each level. It was decided to end the test (that is, to stop loading) when the soil body extruded significantly around the loading plate or the soil body around the loading plate presented obvious uplift or radial crack propagation. The P-S value was summarized and the P-S curve as shown in Fig. 4 was drawn to evaluate the reinforcement effect of the backfilled foundation.

Fig. 4P-S Curve of loading test before and after reinforcement of test zone

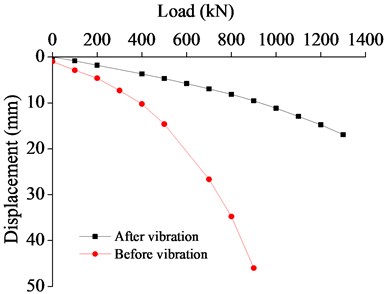
a) Before and after vibration

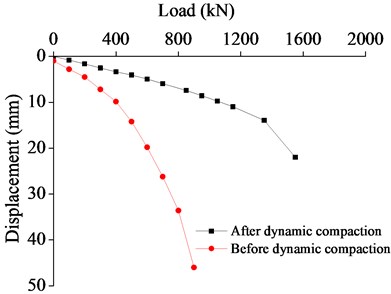
b) Before and after dynamic compaction (500 kJ)

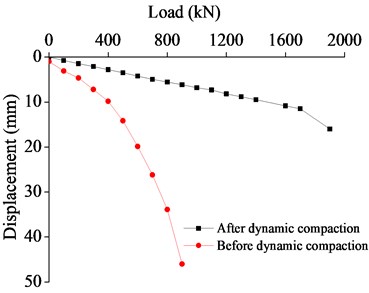
c) Before and after dynamic compaction (1000 kJ)

d) Before and after dynamic compaction (3000 kJ)

As can be seen from the above figures, the P-S curve of coral reef backfill gradually drops and nearly becomes a straight line, lacking the three typical and obvious stages of the typical P-S curve and making it difficult to acquire the proportional limit.

2.2. Determination of bearing capacity

The bearing capacity of coral reef foundation soil can be determined according to the relevant specifications on coral reef geology in the Code for Investigation of Geotechnical Engineering [11] and the Code for Geotechnical Investigation on Port and Waterway Engineering [12], that is, for the relatively flat section of the P-S curve, the stress corresponding to a relative settlement of not greater than 0.02 is taken as the allowable bearing capacity; if the ultimate load $Pu$ is smaller than twice the $P_{s/b=0.02}$, $Pu/2$ is taken as the allowable bearing capacity. Considering the high compressibility of coral sand, $Pu=min$ ($P_{s/b}=$0.02, 1/2$Pu$) for general clay or soft soil is taken as the eigenvalue of bearing capacity $f_a$. Then, the ultimate bearing capacities obtained from the plate loading test on the foundation soil before and after reinforcement are shown in the Table 1 and Table 2.

3.1. Modification of Terzaghi's bearing capacity equation for foundation before reinforcement

The theoretical equation is mainly modified with the mathematical statistics method. The coefficients in the original equation are modified based on the mathematical statistics analysis for the plate loading test. According to previous research results, there is a nonlinear relationship between the bearing properties of the foundation soil and the mechanical parameters of the soil body including cohesion and internal friction angle. Therefore, the power function fitting is used to modify the bearing capacity parameter, as shown in Eq. (7) and Eq. (8):

The power law constants $\alpha$ and $\beta$ vary in the range of 0.5 to 1.0. For $P_u$, the acting forms of cohesion and internal friction angle remain unchanged. $N_c$, $N_q$, $N_r$ are calculated using Eqs. (4), (5) and (6). After the relationship between $P_u$ and the two variables is analyzed, Terzaghi’s ultimate bearing capacity equation is kept unchanged for comparison with the theoretical equation. The variables are included in a trinomial equation to acquire the hypothetical regression equation, as shown in Eq. (9):

where, $b_1$, $b_2$, and $b_3$ are regression coefficients, and other parameters have the same meanings as defined above. Regression analysis is conducted based on the eight bearing capacity test values in the Table in combination with mathematical statistics.

To simplify the analysis steps, the following assumptions are made: $x_1=c{N}_c$, $x_2={\gamma }_1{L}^{\alpha }{N}_q$, $x_3=\gamma d^{\beta }{N}_{\gamma }$. Then the above equation can be transformed into the Eq. (10):

The above equation is a multivariate linear equation. With different values assumed for $\alpha$ and $\beta$, the corresponding regression coefficients $b_1$, $b_2$ and $b_3$ are solved according to the linear regression equation. Then the significance test is conducted to select the optimal regression equation. It is assumed that the ultimate bearing capacity value is a dependent variable and $x_1$, $x_2$ and $x_3$ are independent variables that vary with the variation of the internal friction angle $\psi$. Thus, the internal friction angle $\psi$ is defined as the variable factor of the multivariate linear equation. Considering that all three independent variables are selected through the correlation coefficient method, the screening step is not considered during the regression analysis.

“INPUT” is selected as the regression method for full regression analysis. By comparing the linear correlation coefficient, likelihood ratio, regression equation fitting information and its significance level, and the significance level of the linear coefficient standard value, it is found that when $\alpha =$0.6 and $\beta =$1.0, the regression equation has the highest significance level and the best goodness of fit; the coefficient of multiple determination $R^2$ is 0.981; the linear regression coefficients are as follows: $b_1=$0, $b_2=$2.203, $b_3=$0.029. Thus, the regression equation is shown in Eq. (11):

The multiple linear regression model passes the F-test and the significance level of the linear regression equation is found to be very high according to the residual statistics. In addition, the standardized predicted value based on regression analysis also passes the T-test and presents a high significance level. Through the T-test with a 90 % confidence level, it is determined that the significance levels of regression coefficients $b_1$, $b_2$ and $b_3$ meet the requirements.

Based on the above analysis results, Terzaghi's bearing capacity equation for foundation before reinforcement is shown in Eq. (12):

where ${\gamma }_1$ is the equivalent volumetric weight of the soil body above the foundation in kN.m^-3; $d$ is the diameter of the pile foundation in m; ${\gamma }_{\mathrm{}}$ is the volumetric weight of the foundation soil in kN/m^-3; $c$ is the cohesion of the foundation soil.

Terzaghi’s ultimate bearing capacity coefficients remain unchanged as $N_c$, $N_q$, $N_{{\rm Y}}$, and their values can be calculated with Eqs. (4), (5) and (6) respectively. The internal friction angle and cohesion are put into the fitting equation of the bearing capacity for foundation before reinforcement, and the calculated results are compared with the actual measured values. It can be seen from Fig. 5 that the calculated values are different from the actual measured values. Most results of the modified Terzaghi’s theoretical bearing capacity equation is larger than the measured values, but the values differ from each other generally within a small range, not exceeding 10 %.

Fig. 5Comparison between modified value and measured value curves before reinforcement

3.2. Modification of Terzaghi’s bearing capacity equation for foundation before reinforcement

The field loading test values of the bearing capacity for the foundation after reinforcement are summarized as shown in Table 5. It can be found that after vibration rolling and dynamic compaction, the cohesion of the soil body has increased compared with that before reinforcement, but limited to a small scale, and its value is taken within the range of 0-1; the internal friction angle has increased significantly compared with that before reinforcement, indicating that the strength of the soil body has been further improved, and the ultimate bearing capacity of the foundation has increased significantly. Now Terzaghi's bearing capacity equation for foundation is modified, mainly by the same steps as stated above.

It can be seen from Table 5 that the calculation results of the bearing capacity are rather large by using the original Terzaghi’s bearing capacity equation for coral reef sandy soil foundation after reinforcement. They deviate largely from the measured data and are about 2 times larger, and some 3 times larger. The calculation results of the unmodified Terzaghi’s bearing capacity equation for coral reef sandy soil present large errors and are inconsistent with the actual engineering conditions.

The assumed regression equation is the same as Eq. (11). When different values are assumed for $\alpha$ and $\beta$, the corresponding regression coefficients $b_1$, $b_2$ and $b_3$ are solved according to the linear regression equation, and then the significance testing is conducted to select the optimal regression equation. It is assumed that the ultimate bearing capacity value is the dependent variable and $x_1$, $x_2$ and $x_3$ are independent variables and vary with the variation of the internal friction angle $\psi$, so the internal friction angle ψ is defined as the variable factor of the multivariate linear equation. Considering that all three independent variables are selected through the correlation coefficient method, the screening step is not considered during the regression analysis. Inputs are selected in the regression method for full regression analysis. By comparing the indicators including linear correlation coefficient, likelihood ratio, regression equation fitting information and its significance level, and the significance level of the linear coefficient standard value, it is found that when $\alpha =$0.6, $\beta =$1.0, the regression equation is at the highest significance level. At this time, the fitting of the equation is optimal. The coefficient of multiple determination $R^2$ is 0.827, and the linear regression coefficients are as follows: $b_1=$18.799, $b_2=$0.0816, $b_3=$0.735, then the modified Terzaghi’s foundation bearing capacity equation after reinforcement are shown in Eq. (13) and Eq. (14):

where ${\gamma }_1$ is the equivalent volumetric weight of the soil body above the foundation in kN.m ^-3; $d$ is the diameter of the pile foundation in m; $\gamma$ is the volumetric weight of the foundation soil in kN/m^-3; $c$ is the cohesion of the foundation soil.

The multiple linear regression model passes the F-test and the significance level of the linear regression equation is found to be very high according to the residual statistics. In addition, the normal expected value of regression analysis also passes the T-test and presents a high significance level. Through the T-test with the confidence level at 90 %, it is determined that the significance levels of regression coefficients $b_1$, $b_2$ and $b_3$ meet the requirements.

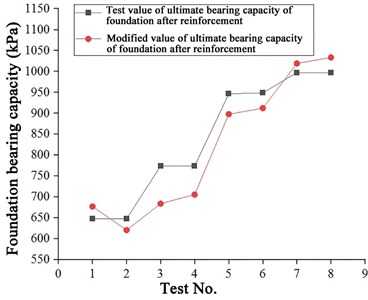
Terzaghi’s ultimate bearing capacity coefficients remain unchanged as $N_c$, $N_q$, $N_{{\rm Y}}$. After calculation, the internal friction angle and cohesion are put into the fitting equation of the foundation bearing capacity after reinforcement, and the calculated results are compared with the measured values. It can be seen from Fig. 6 that the calculated values are different from the measured values. Most results of Terzaghi’s theoretical bearing capacity equation are smaller than the field measured values. Among them, the modified value of bearing capacity after 500 kJ dynamic compaction differs from the test value largely, nearly 10 %. The modified values after other reinforcement measures are basically consistent with the test values, generally with a small deviation. This modified equation can provide a feasible method for calculating the bearing capacity of the coral reef sandy soil or calcareous soil foundation after reinforcement.

Fig. 6Comparison between modification value and measured value curves after reinforcement