Numerical simulation of the propagation and distribution of explosion shock waves in trenches

2.1. Model establishment

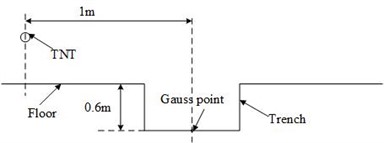
The numerical simulation analysis was conducted based on the testing scheme under actual damage conditions. The model mainly consists of the visible air domain, TNT explosives, and sand soil. A two-dimensional axisymmetric modeling approach was adopted to simplify the model algorithm. The size of the visible air domain is 20000 mm×8000 mm, with a specific grid cell size of 20 mm×20 mm. The size of the sand soil region is 20000 mm×2000 mm, with the same grid cell size as the air domain. According to typical high-energy warhead tests, a cylindrical TNT explosive weighing 50 kg was placed at the center of the air domain. The length-to-diameter ratio of the explosive column is 1:1, and the detonation was initiated at the center with a height of 2000 mm. Two sets of comparative experiments were set up: Experiment 1 focused on the impact of trench depth on explosion shock waves. The TNT explosive was placed 1 m away from the start of the trench, with a trench length of 400 mm. Trench depths of 0 mm, 200 mm, 400 mm, 600 mm, 800 mm, and 1000 mm were selected. Gaussian monitoring points were set at the middle of the trench, along the edges of the trench, and 200 mm away from the edge in the direction of the TNT explosive and the ground, resulting in a total of 5 pressure measurement points. Experiment 2 focused on the impact of trench length on explosion shock waves. The trench depth was set at 600 mm, and the trench lengths were set as 400 mm, 600 mm, 800 mm, 1000 mm, and 1200 mm. The measurement points were distributed at the middle position of the trench bottom, with a lateral distance of 1000 mm from the TNT explosive. A rigid interface was set at the bottom of the model, and outflow boundaries were applied to the left and top of the air domain to ensure normal air flow within the region and to avoid the reflection of shock waves caused by boundary conditions. The computational model is shown in Fig. 1, and the layout of sensor measurement points is illustrated in Fig. 2 and Fig. 3.

Fig. 1Finite element numerical simulation model

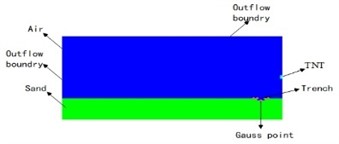
Fig. 2Layout of sensor measurement points

Fig. 3Layout of sensor measurement points

2.2. Material model

In the model, the TNT material in the explosive filling is described using the JWL state equ ation, as shown by the following expression [10-12]:

where, $P$ represents the pressure of TNT explosive during detonation; $V$ represents the relative volume; $E$ represents the specific internal energy of unit mass of TNT explosive; $A$, $B$, $R_1$, $R_2$, and $w$ are the five parameters of the JWL state equation, where $A$ and $B$ are material parameters, and $R_1$, $R_2$, and $w$ are constants. The specific forms of these parameters are shown in Table 1 [13].

The air medium is described using the ideal gas state equation, as shown by the following equation:

where, $P$ represents the pressure of the detonation products; $\gamma$ is the adiabatic index, with a value of $\gamma =$1.4; ${\rho }_g$ represents the air density, with a value of ${\rho }_g=$1.225 kg/m³; $e_g$ represents the initial specific internal energy of air, with a value of $e_g=$2.068×10⁵ J/kg.

3.1. Comparison of shock wave pressure curves

To characterize the influence of trenches on the overpressure of shock waves, detonations were initiated at the center of the ground surface and a trench with a length of 400 mm and a depth of 600 mm, using a 50 kg TNT explosive. The pressure-time curves of the shock waves at measurement points 3 and 5 on both the ground surface and the trench were obtained, as shown in Fig. 4.

Fig. 4Pressure curves of shock waves on flat ground and in trenches

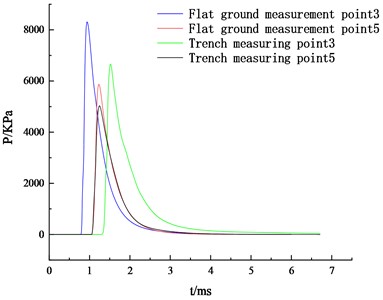
TNT explosions generate spherical waves that propagate outward. When the shock wave approaches a trench, the propagation space suddenly increases and the airflow expands continuously, creating a pressure difference between the front and back of the trench. This causes the shock wave to travel downward, impacting the interior of the trench. By analyzing Fig. 4, it can be observed that at measurement point 3 located at the center of the trench bottom, the shock wave pressure decreases relatively smoothly. The same applies to measurement point 5, but with a smaller decrease in magnitude. Meanwhile, compared to the same position inside the trench, measurement point 3 on the flat ground experiences an earlier arrival time for the peak of the shock wave. Combining this with the evolution of shock wave pressure contour maps at different times, it can be inferred that when the propagation time reaches 0.8 ms, the shock wave reaches the edge of the trench. At 1.25 ms, refraction and reflection occur within the trench, and the shock wave reaches measurement point 5 before reaching measurement point 3, reaching its peak pressure. At 1.5 ms, after propagating and undergoing successive reflections, the shock wave reaches measurement point 3, where the energy of the shock wave is focused and reaches its peak value. At the same time, at measurement point 5, the shock wave undergoes reflection and absorption by the ground, resulting in a decrease in pressure. At 1.6 ms, the shock wave pressure at measurement point 3 also begins to decrease. The time-varying shock wave pressure contour is shown in Fig. 5.

Fig. 5Pressure contour of shock wave pressure variation with time

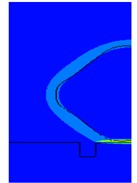
a) 0.8 ms

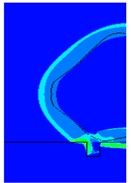
b) 1.2 ms

c) 1.5 ms

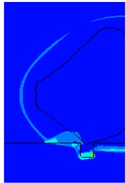
d) 1.6 ms

3.2. Influence of depth on peak overpressure of shock wave

To reflect the impact of different trench depths on the explosive shock wave, six different ground conditions were set up: a flat ground and trenches with depths of 200 mm, 400 mm, 600 mm, 800 mm, and 1000 mm, all with a length of 400 mm. The pressure of the shock wave at various measurement points was obtained, as shown in Table 2.

From the table, it can be observed that the peak overpressure at measurement point 1 remains unchanged, indicating that the pressure of the shock wave on the flat ground in front of the trench is not affected. When the shock wave reaches the edge of the trench, the propagation space suddenly increases, resulting in a larger dispersed area of shock wave energy and a decrease in peak pressure. Therefore, at measurement point 2 on the trench floor, the pressure is significantly reduced compared to on the flat ground. However, as the depth of the trench increases, the peak overpressure of the shock wave at measurement point 2 on the trench floor remains unchanged. As the shock wave continues to propagate forward into the trench, reflection and refraction occur within the trench, leading to a continuous attenuation of energy and a decrease in shock wave pressure. From the table, it can be observed that at different depths in the middle of the trench bottom, the peak overpressure of the shock wave decreases compared to on the flat ground, but the extent of attenuation varies. As the depth of the trench increases, the peak pressure of the shock wave initially decreases and then increases. Additionally, it can be seen that when the depth of the trench is 200 mm, as the shock wave propagates to the back of the trench, compared to on the flat ground, the shock wave focuses within the trench, resulting in an increase in energy density and an increase in the peak overpressure of the shock wave. At measurement point 4, the shock wave pressure significantly increases compared to on the flat ground. However, as the depth increases, the internal space of the trench becomes larger, leading to more energy dispersion and a decrease in shock wave pressure. When the depth exceeds 600 mm, the pressure begins to stabilize. When the shock wave propagates to the flat ground at the rear of the trench, at the same measurement point location, the peak pressure of the shock wave decreases. As the depth increases, the pressure value remains constant, and the increasing depth has no effect on the shock wave pressure at measurement point 5.

3.3. Influence of length on peak overpressure of shock wave

In order to study the effect of trench length on the peak overpressure of the shock wave, the pressure-time curves of the shock wave at different trench lengths located at the middle position of the trench bottom with the same depth and TNT equivalent of 50 kg were obtained, as shown in Fig. 6.

Fig. 6Pressure-time curves of chock wave for trenches of different lengths

As you can see from Fig. 6, as the length of the trench increases, the shock wave overpressure peak at the same position in the middle of the trench bottom first decreases and then increases. When the trench length is 400 mm to 600 mm, the shock wave pressure continues to decline, and the length is 600 mm to 1200 mm. When the shock wave pressure starts to increase. Combined with specific data analysis, the peak pressure at the center of the bottom surface of the trench of different lengths is 8.23 KPa (400 mm), 4.41 KPa (600 mm), 4.70 KPa (800 mm), 5.42 KPa (1000 mm), 5.94 KPa (12000 mm), and the peak pressure attenuation range of the shock wave at the bottom center of the trench length is 46.42 % (600 mm), 42.89 % (800 mm), 34.14 % (1000 mm), 27.82 % (1200 mm). This indicates that for trenches of the same depth, as the length increases, the shock wave pressure at the same location in the middle of the trench bottom is not consistent. The peak overpressure of the shock wave decreases and then increases with the increase in trench length.