Nonlinear dynamics of a two-stage gear system with consideration given to the torsional stiffness of the countershaft

1. Introduction

Analyses of gear dynamic, besides the pure cognitive aspect, should have the utilitarian aspect. Gears, among whole group of mechanical gears have numerous advantages and major implementation in machine driving systems.

According to the above-mentioned fact, gear dynamic investigations are performed since many years in numerous research centers all around the world. Knowledge acquired in this area in connection with appropriate implementation of available technical measures enables reduction of component value in dynamic interdental force, what could directly influence increase in durability and reliability.

Gear dynamics investigations are conduced on existing objects (prototypes), laboratory models and dynamic gear models. Especially the latest measure is in recent years frequently used. It is caused due the fact, that gears are strongly nonlinear systems, what induces problems in obtaining accurate results from experimental studies. Measurement of dynamic work effects in gear is measurement conducted indirectly, with most prevalently unknown or scarce known transition function between the starting point and the information collecting point [1].

Overview of international technical literature may suggest, that the main objects of dynamic analysis are one-stage gears [2-8]. Low number of analyses concern multi-stage gears, despite the fact that the multi-stage gears constitute the majority of all working gears. Numerous investigators who explored dynamic phenomena [9-14] showed that forces acting on one stage could have an influence on dynamic state of adjacent stage. The occurrence of interstage dynamic interactions results from design of gear stages coupled by an intermediate shaft. Dynamic in two-stage gear system seems to be different from dynamic in isolated gear stages [13, 15].

In the aspect of interstage dynamic action occurring in multi-stage gear, torsional stiffness of the countershaft assumed to be the constructional parameter of greatest significance [9, 10, 13]. Considerations presented in further part of the study concern influence of this parameter on nonlinear action of particular gear stages belonging to the simulated two-stage gear.

2. Methodology and research object

In order to identify the influence of countershaft torsional stiffness on nonlinear gear behavior conducted was a number of computer simulation prepared on discrete dynamic model of two-stage gear. The model due to geometrical combination of gear stages enables conduction of system dynamic investigation in holistic aspect with consideration given to dynamic interstage coupling. In the model considered were, among others function of stiffness variable in the course of time, depending on the number of teeth couples existing temporary in mesh (the course of function was established according to [16]), damping in mesh and occurrence of backlash.

Interdental backlash provides appropriate smearing of collaborating teeth and prevents teeth blockade, what saying another words is indispensable in optimal gear collaboration. On the other hand interdental baclash is responsible for loss of contact between collaborating teeth, when strong gears vibrations occur and the resonance band is passed. Possibility of collision with neighboring tooth belonging to collaborating gear depends on the vibrations level and degree of interdental backlash. In a case of recurrent fitting in mesh collision also occurs, but in this case is characterized by collision of nominal collaborating teeth. As a consequence of the above-mentioned facts the gear is nonlinear dynamic system.

Discrete dynamic model with describing this model normal differential equations with variable factors was characterized previously in details by [13] and [17]. In order to obtain model solution computer program was prepared. After validation and verification process described in [13, 18, 19] dynamic model together with computer program were accepted for investigations.

Results obtained directly from model equations relying on fifth order Runge-Kutta-Felhberg numerical method with optimized step size do not supply clear technical information. Regarding the above-mentioned fact so-called intrinisic dynamic factor $K_V$ according to ISO 6336 [21] norm was determined. The factor describes relation between total tooth burden, including influence of dynamic burden and nominal tooth burden. Its value is calculated according to the dependence:

where $\mathrm{m}\mathrm{a}\mathrm{x}\left\{F^{\mathrm{\Sigma }}\right\}$ is maximal value of interdental force comprising sum of static and dynamic force, $F^{nom}$ is nominal (static) value of interdental force, resulting from theory of evolvent mesh.

As mentioned previously, gear model takes into consideration dynamic interdental bound. Neighboring gear stages are connected by common part – countershaft, on which gear belonging to the first step and pinion of the second step are placed. Countershaft play a role in transferring torque, saying another words it participates in transmission of vibrations connected with working gear. In the aspect of interstage dynamical interactions, constructional parameter having a pivotal role is a value of torsional stiffness of the countershaft [13]. Having regard to this fact, in further part of the study simulations were conducted with consideration given to torsional vibrations.

Value of torsional stiffness of the countershaft was referred in relation to mesh torsional stiffness as presented in Eq. (3). Owing this the two significant for system dynamic constructional parameters were bounded [10].

Value of mesh torsional stiffness $c_{\theta 2-3}$ is defined by equation (presented below descriptions are concordant with descriptions of dynamic model of two-stage gear presented among others in studies [13] and [17]):

where $c_{2-3}$ is efficient value of mesh stiffness belonging to first stage of gear, $r_{b3}$ is gear cardinal radius of first degree.

Relationship $\rho$ between the two stiffness values presents the dependence:

where $c_{\theta y3-4}$ is torsional stiffness of the gear countershaft.

As remarked at the beginning of the chapter, the object of the study was two-stage gear with straight uncorrected teeth; the basic geometrical data and load values from particular stages are presented in table beneath. It was presumed, that the gear has no errors.

3. Research results

Considering basic gear parameters (Table 1) relation between mesh torsional stiffness and countershaft described by the Eq. (3) was about 6.1. Regarding this fact, the countershaft was characterized by relative low stiffness when compared to mesh torsional stiffness. However, in typical multistage gear transmission systems are placed close to each other due to compact construction. In this case we have situation, when torsional stiffness of the countershaft is comparable with mesh torsional stiffness [10]. According to this simulations were conduced for proportion of stiffness ranging from 0.1 to 10. Therefore, countershaft had torsional stiffness higher and lower than efficient value of torsional mesh stiffness in the range mostly characterizing real constructions.

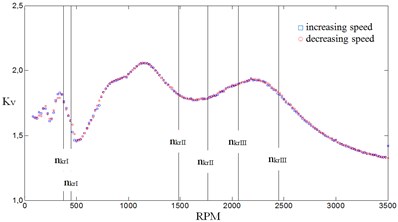
Simulations were prepared for the range of increasing and decreasing rotational speed (RPM) of input gear shaft, comprising the three main resonance frequencies delivered by the first and second stage. Vertical lines on presented charts show critical rotational speed corresponding with resonance extortive frequencies proceeded from mesh.

Data obtained in this way are presented on graphs illustrating factor value $K_V$ in the function of previously defined proportion of stiffness. The most representative courses of dynamic factor value in the function of rotational speed for proportion of stiffness 0.1, 1 and 10 are presented in Figs. 1-3.

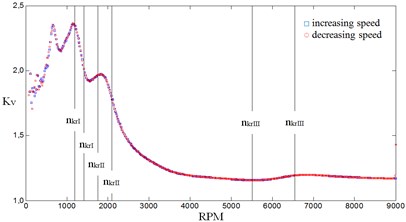
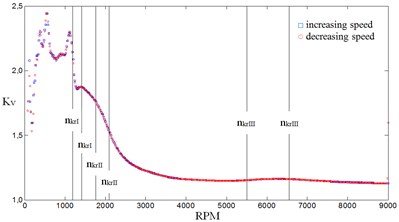
Fig. 1Values of dynamic factor Kv in first stage of gear in the function of input shaft rotational speed for stiffness proportion equaling 0.1: a) first stage gear, b) second stage gear

a)

b)

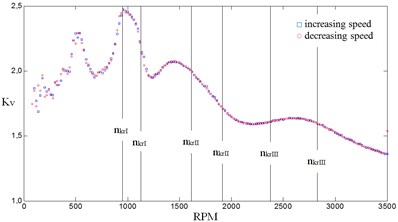
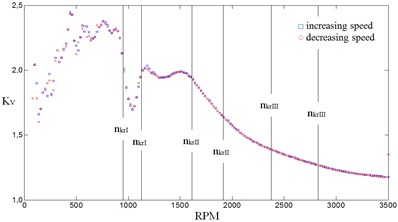
The investigation showed that dynamic of two-stage gear remains unchanged despite increase or decrease in rotational speed. It is represented by course of dynamic factors $K_V$ of both gear stages during increase or decrease in rotational speed, presented in Figs. 1-3. Disappearance of nonlinear phenomena is also observed.

Nonlinear behavior is observed dugapring transition through resonance band, when collaborating teeth tear off due to strong vibrations. Eventual collision with neighboring tooth and recurrent fitting in mesh causes occurrence of dynamic forces significantly exceeding nominal force value. This is particularly visible while rotational speed is decreasing. Dependence characterizing one-stage gears does not exist for two-stage gear in the investigated range of stiffness proportion.

Fig. 2Values of dynamic factor Kv in first stage of gear in the function of input shaft rotational speed for stiffness proportion equaling 1: a) first stage gear, b) second stage gear

a)

b)

Fig. 3Values of dynamic factor Kv in first stage of gear in the function of input shaft rotational speed for stiffness proportion equaling 10: a) first stage gear, b) second stage gear

a)

b)

Analyzing demonstrated study results it could be stated that increase in torsional stiffness of the countershaft results in increasing of dynamic interstage connexion. Dynamic activity of the first and second gear stage is also increased in the form of resonance reactions to the own vibrations frequency ($n_{krI}$). As a consequence, the highest values of dynamic factor $K_V$ are noted exactly for this resonance velocity at high torsional stiffness of the countershaft ($\rho <1$). In a case in which countershaft stiffness is lower than mesh torsional stiffness ($\rho >1$) the dynamic gear connexion decreases. However, the dynamic connexion still remains high, what enable dynamic behavior of gear stages as in the case of particular stages. It shows lack on nonlinear behavior in gear dynamic.

4. Conclusions

Computer simulation results showed that torsional stiffness of the countershaft is important in the aspect of dynamic state of two-stage gear. In presented range of proportion between torsional stiffness of the countershaft and efficient value of mesh torsional stiffness it was elucidated that interstage connexion is responsible for gear dynamical state different from dynamical state of one-stage gear. It is caused due the fact that passing through the resonance band is connected with loss of nonlinear behaviors.

Torsional stiffness of the countershaft significantly exceeding mesh stiffness leads to prominent coupling of both gear stages for critical velocity of input shaft corresponding with mesh extortion frequency equaling resonance frequency of countershaft.

This study presenting analysis of computer simulation results aims to take contribution to knowledge about multistage gear dynamics. Authors of presented publication are conscious, that in order to draw the final conclusions further studies in this area are needed. Into analysis should be taken numerous combinations of constructional gear parameters.