Fractional order magneto-thermoelasticity in a rotating media with one relaxation time

1. Introduction

Fractional calculus has been used successfully to modify many existing models of physical processes e.g., chemistry, wave propagation, visco-elasticity, electronics and biology. One can state that the whole theory of fractional derivatives and integrals was established in the second half of the nineteenth century. Various definitions and approaches of fractional derivatives have become the main purpose of many studies. There are some materials (e.g. porous materials, biological materials/polymers and colloids, glassy etc.) and physical situations (like low-temperature, amorphous media and transient loading etc.) where the conventional coupled dynamical theory (CD) [1] based on the classical Fourier’s law is unsuitable (see [2] for details). In such cases, one needs to use a generalized thermoelastic (and more generally thermos-viscoelastic) model based on an anomalous heat conduction theory involving fractional time-derivatives, see Ignaczak and Ostoja-Starzewski [3].

Fractional order derivatives have been employed for the description of visco-elastic materials by Caputo and Mainardi [4, 5] and Caputo [6] and they have successfully established the connection between the fractional order derivatives and the linear theory of visco-elasticity. They also have obtained a very good agreement with the experimental results successfully. Some applications of fractional calculus to various problems of mechanics of solids are reviewed in the literatures Rabotnov [7] and Mainardi [8]. A considerable research effort has been extended to study anomalous diffusion that is characterized by the time-fractional diffusion wave equation introduced by Kimmich [9].

Fractional calculus has also been employed in the theory of thermoelasticity. Povstenko [10] has constructed a quasi-static uncoupled thermoelasticity model based on the heat conduction equation with fractional order time derivatives. He has used the Caputo fractional derivative (see [11] for details) and obtained the stress components corresponding to the fundamental solution of a Cauchy problem for the fractional order heat conduction equation in both the one-dimensional and two-dimensional cases. In 2010, a new theory of generalized thermoelasticity in the context of a new consideration of the heat conduction equation with fractional order time derivatives has been proposed by Youssef [12]. The uniqueness of the solution has also been proved in the same work. Youssef and Al-Lehaibi [13] have studied a problem on an elastic half space using this theory. Sherief et al. [14], Ezzat and Fayik [15], Ezzat and El-Karamany [16, 17] and Bachher et al. [18, 19] have also constructed some model in generalized thermoelasticity by using fractional calculus.

Investigation of the interaction between magnetic field and stress and strain in a thermoelastic solid is very important due to its many applications in the field of geophysics, plasma physics and related topics. Especially in nuclear fields, the extremely high temperatures and temperature gradients, as well as the magnetic fields originating inside nuclear reactors, influence their design and operations.

During the second half of 20th century, great attention has been devoted to the study of electromagneto-thermoelastic coupled problems based on the generalized thermoelasticity. The magneto-thermoelastic disturbances generated by a thermal shock in an elastic half-space having a finite conductivity has been investigated by Kaliski and Nowacki [20]. Later Massalas and Dalamangas [21] considered the same problem by taking into account the coupling between the temperature and the strain field. Paria [22, 23] also solved some problems in magneto-elasticity and magneto-thermoelasticity. One can find in [24-34] some other works in generalized magneto-thermoelasticity.

Study of plane thermoelastic and magneto-thermoelastic wave propagation in a non-rotating medium is receiving considerable attention in recent years. It appears that little attention has been paid to the study of propagation of plane thermoelastic waves in a rotating medium using the linear models (L-S, G-L and G-N models) of generalized thermoelasticity. Since most large bodies, like the earth, the moon, and other planets, have an angular velocity, it appears more realistic to study the propagation of plane thermoelastic or magneto-thermoelastic waves in a rotating medium with thermal relaxation. One can see the references [27-31, 35-37] for various study for generalized thermoelastic rotating medium.

In the present paper, the model of the equations of fractional order generalized magneto-thermoelasticity with one relaxation time in a homogeneous isotropic rotating elastic medium is established by using the Caputo time-fractional derivative [14]. The surface of the half-space is taken to be traction free and subjected to a thermal shock. There acts an initial magnetic field parallel to the plane boundary of the half-space. The normal mode analysis [38, 39] technique is applied to obtain the exact expressions for the considered field quantities. The distributions of the considered field variables are represented graphically for a hypothetical material. From the distributions, the wave type heat propagation is found in the medium.

2. Formulation of the problem and governing equations

We shall consider the problem of a homogeneous, isotropic, thermally and electrically conducting elastic half-space $(x\ge 0)$ in two-dimensional space. The $x$-axis is taken as vertically inwards. The whole body is initially at a uniform temperature $T_0$. The surface of the half-space is subjected to a thermal shock that is a function of $y$ and $t$. Thus, all the field variables considered will be functions of the time variable $t$ and of the coordinates $x$ and $y$. Also the considered field quantities are assumed to be vanished as $x\to +\mathrm{\infty }$. The medium is placed in a magnetic field with constant intensity ${\overrightarrow{H}}_0$ acting in the $z$-direction so that ${\overrightarrow{H}}_0=(\mathrm{0,0},H_0)$, where $H_0$ is a constant. Due to the application of this initial magnetic field, there results an induced magnetic field $\overrightarrow{h}$ and an induced electric field $\overrightarrow{E}$. We assume that both $\overrightarrow{h}$ and $\overrightarrow{E}$ are small in magnitude in accordance with the assumptions of the linear theory of thermoelasticity.

The elastic medium is rotating uniformly with an angular velocity $\overrightarrow{\mathrm{\Omega }}=\mathrm{\Omega }\overrightarrow{n}$, where $\overrightarrow{n}$ is a unit vector representing the direction of the axis of rotation. The displacement equation of motion in the rotating frame of reference has two additional terms [36, 37]:

i) Centripetal acceleration, $\overrightarrow{\mathrm{\Omega }}\times (\overrightarrow{\mathrm{\Omega }}\times \overrightarrow{u})$ due to time-varying motion only and

ii) Corioli's acceleration $2\overrightarrow{\mathrm{\Omega }}\times \overrightarrow{\dot{u}}$ where $\overrightarrow{u}$ is the displacement vector. These terms don't appear in non-rotating media.

The simplified linearized Maxwell’s equations of electrodynamics for a homogeneous isotropic perfectly conducting slowly moving elastic medium are:

where $\overrightarrow{H}={\overrightarrow{H}}_0+\overrightarrow{h}$.

All the considered field quantities will depend on spatial coordinate $x,y$ and time $t$, so that for two-dimensional deformation in the $xy$-plane:

The electric intensity vector $\overrightarrow{E}$ is normal to both the magnetic intensity filed and the displacement vector. Thus for ${\overrightarrow{H}}_0=(\mathrm{0,0},H_0)$, $\overrightarrow{E}$ has the components:

Since the current density vector $\overrightarrow{J}$ is parallel to the electric intensity vector $\overrightarrow{E}$, it has the components:

From Eq. (3), we get after linearization:

Now, Eqs. (2) and (5) gives:

From Eqs. (1) and (8) we obtain:

Using Eqs. (8) and (9), we get the components of Lorentz force as:

For a homogeneous isotropic rotating elastic medium placed in a magnetic field with constant intensity ${\overrightarrow{H}}_0$, the displacement equation of motion is:

The heat conduction equation with Caputo time-fractional derivative [14]:

The constitutive equations are given by:

where:

the Riemann-Liouville fractional integral operator $I^{\nu }$ is defined as:

and $F_i$ are the components of the Lorentz force vector $\overrightarrow{F}$. In the above equations, a comma followed by a suffix denotes material derivative and a superposed dot denotes the derivative with respect to time $t$.

The strain tensor $e_{ij}$ has the components:

For two-dimensional deformation in the $xy$-plane, we have:

In order to make the Eqs. (15)-(20) dimensionless, the following non-dimension quantities are introduced:

Making use of Eq. (21) into Eqs. (15)-(20), we get (after suppressing the primes):

Let us introduce the displacement potentials $\phi$ and $\psi$ by the relations function:

By differentiating Eq. (22) with respect to $x$ and Eq. (23) with respect to $y$, then adding, we obtain:

and by differentiating Eq. (22) with respect to $y$ and Eq. (23) with respect to $x$ and subtracting we obtain:

Eq. (24) can be written as:

3. Normal mode analysis

The solution of the physical variables can be decomposed in terms of the normal modes [38, 39] in the following way:

where $u^{\mathrm{*}}\left(x\right)$ etc. is the amplitude of the function $u(x,y,t)$ etc., $i$ is the imaginary unit, $\omega$ (complex) is the time constant and $a$ is the wave number in the $y$-direction.

Substituting from Eq. (33) into Eqs. (30)-(32), we arrive at a system of their homogeneous equations:

where:

Eliminating ${\psi }^{\mathrm{*}}\left(x\right)$ and ${\theta }^{\mathrm{*}}\left(x\right)$ between Eqs. (34)-(36), we obtain the following sixth order differential equation satisfied by ${\phi }^{\mathrm{*}}\left(x\right)$:

Where:

In a similar manner, we get the following equations:

The general solution of the Eq. (38)-(40) can be obtained as:

where $k_j,j=\mathrm{1,2},3$ is the root of the following characteristic equation:

and $A_j,B_j$ and $C_j$ are some parameters depending on $a$ and $\omega$.

Substituting from Eqs. (41)-(43) into Eqs. (34)-(36), we get:

In order to obtain the displacement components $u$ and $v$, Using Eqs. (33), (41) and (42) in Eq. (28), we obtain:

The stress components can be obtained as:

where:

4. Application

The normal mode analysis is, in fact, to look for the solution in the Fourier transformed domain. Assuming that all the relations are sufficiently smooth on the real line such that the normal mode analysis of these functions exist. The initial conditions of the problem are taken as homogeneous i.e., $\theta =u=v=0$ and $\dot{\theta }=\dot{u}=\dot{v}=0$ at $t=0$. In order to determine the parameters $A_j(j=\mathrm{1,2},3)$, we need to consider the boundary conditions at $x=0$ as following:

i) Thermal boundary condition that the surface $x=0$ of the half-space $x\ge 0$ subjected to a thermal shock:

ii) Mechanical boundary condition that the surface $x=0$ of the half-space $x\ge 0$ is traction free:

Substituting from the expressions of considered variables into the above boundary conditions, we can obtain the following system of equations satisfied by the parameters $A_j(j=\mathrm{1,2},3)$:

Solving (53), we obtain the constants $A_j$ as follows:

where $\mathrm{\Delta }=d_{21}(P_2{R}_3-P_3{R}_2)+d_{22}(P_3{R}_1-P_1{R}_3)+d_{23}(P_1{R}_2-P_2{R}_1).$

5. Numerical results and discussions

With a view to illustrating the analytical procedure presented earlier, we consider now a numerical example. The results depict the variation of the amplitudes of displacements, temperature and thermal stresses. For this purpose, the following values of the different physical parameters of the copper like material were chosen [36]:

Let $\omega =\zeta +i\eta$. Then $e^{\omega t}=e^{\zeta t}\left(\mathrm{c}\mathrm{o}\mathrm{s}\eta t+i\mathrm{s}\mathrm{i}\mathrm{n}\eta t\right)$. So for small values of $t$, we can assume $\omega =\zeta .$ The other constants of the problem were taken as: $\zeta =2,\eta =0.3,a=1,f^{\mathrm{*}}=50.$

The computations were carried out for a small value of time $t=0.1$. The real part of the non-dimensional field quantities $\theta (x,y,t)$, the horizontal displacement $u(x,y,t)$, the vertical displacement $v(x,y,t)$ and the stress components ${\sigma }_{xx}(x,y,t)$ and ${\sigma }_{yy}(x,y,t)$ on the plane $y=0$ are computed for two different values of the fractional parameter $\nu =\mathrm{0.1,1.0}$ and two different values of rotation $\mathrm{\Omega }=\mathrm{0.5,0.8}$ respectively for $M=2.5$. The computed numerical results are presented graphically in Figs. 1–10 with respect to a wide range of values of the distance $x(0.0\le x\le 3.0)$. In Figs. 1–5, the dashed-dot line (-.-) when $\nu =1.0$ refer to the generalized magneto-thermoelsticity theory with one relaxation time (LS model) in the presence of rotation whereas the solid line (-) for $\nu =0.1$ refer to the fractional order theory of generalized magneto-thermoelsticity with one relaxation time with rotation. It is also noted that, in Figs. 6-10, the solid line (-) present the curve when $\mathrm{\Omega }=0.5$ and the dashed-dot line (-.-) represent curve for $\mathrm{\Omega }=0.8$ for the fractional order theory of generalized thermoelsticity with one relaxation time in the presence rotation and magnetic field.

Fig. 1Temperature distribution for y=0.0,Ω=0.5,M=2.5

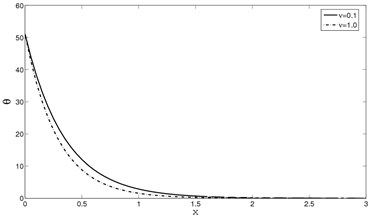
Fig. 2Horizontal displacement (u) distribution for y=0.0,Ω=0.5,M=2.5

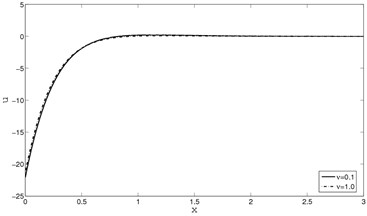
Fig. 3Vertical displacement (v) distribution for y=0.0,Ω=0.5,M=2.5

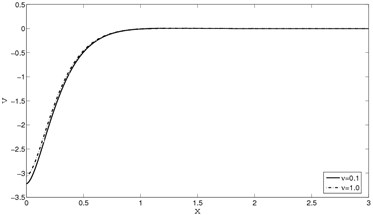
Fig. 4The distribution of stress σxx for y=0.0,Ω=0.5,M=2.5

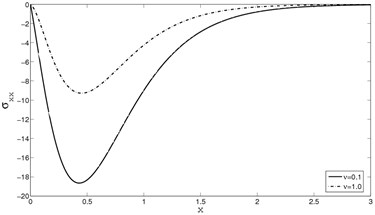
Fig. 5The distribution of stress σyy for y=0.0,Ω=0.5,M=2.5

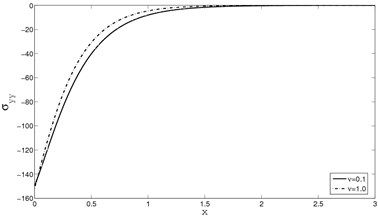
Fig. 6Temperature distribution for y=0.0,ν=0.5,M=2.5

Fig. 7Horizontal displacement (u) distribution for y=0.0,ν=0.5,M=2.5

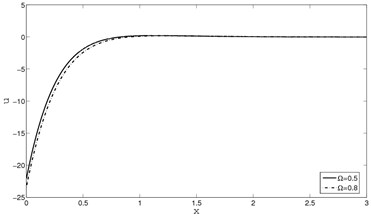
Fig. 8Vertical displacement (v) distribution for y=0.0,ν=0.5,M=2.5

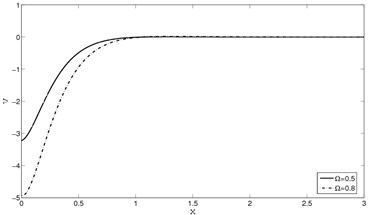
Fig. 9The distribution of stress σxx for y=0.0,ν=0.5,M=2.5

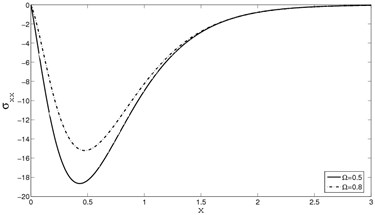
Fig. 10The distribution of stress σyy for y=0.0,ν=0.5,M=2.5

Fig. 113D temperature distribution for y=0.0,Ω=0.5,M=2.5

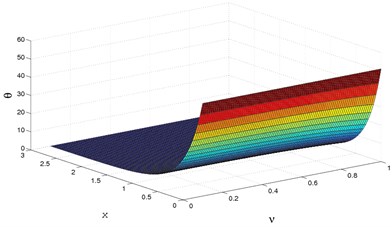
Fig. 123D horizontal displacement (u) distribution for y=0.0,Ω=0.5,M=2.5

Fig. 133D vertical displacement (v) distribution for y=0.0,Ω=0.5,M=2.5

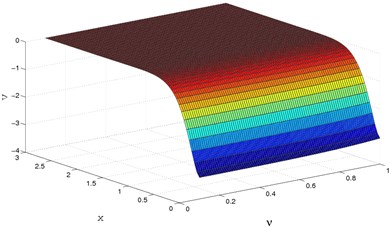
Fig. 143D stress(σxx) distribution for y=0.0,Ω=0.5,M=2.5

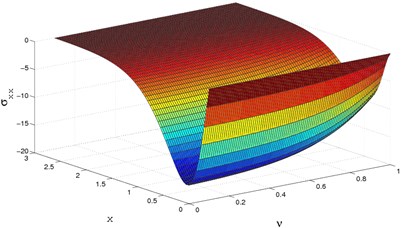
Fig. 153D stress(σyy) distribution for y=0.0,Ω=0.5,M=2.5

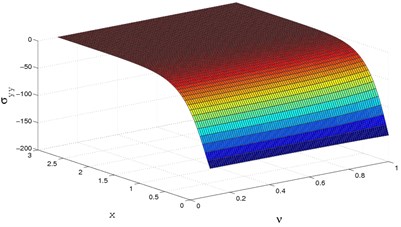
Fig. 163D stress(σxy) distribution for y=0.0,Ω=0.5,M=2.5

From Fig. 1, it is noticed that the temperature decreases with the increase of value of the fractional order parameter $\nu$. In all the cases, the temperature attains maximum value at beginning, and then continuously decreases to zero. Fig. 2 and 3 shows that the fractional order parameter acts to decrease the magnitude of the displacement components $u$ and $v$. Significant changes of the magnitude of $u$ and $v$ can only be noticed in the range $x(0.0\le x\le 0.7)$. From Fig. 4 and 5, it is exhibited that the magnitude of the stresses ${\sigma }_{xx}(x,y,t)$ and ${\sigma }_{yy}(x,y,t)$ decrease with the increase of the fractional order parameter $\nu$. All the curves in Figs. 1-5 converge to zero with the increase of $x$. Figs. 1 and 3 show that the boundary conditions of the problem are satisfied.

Fig. 6 depicts that the rotation $\mathrm{\Omega }$ has no significant effect on the temperature distribution at $t=0.1,y=0,\nu =0.5,M=2.5$. From Fig. 7 and 8, we can see that magnitude of the horizontal displacement $u$ and the vertical displacement $v$ increase with the increasing value of $\mathrm{\Omega }$ and finally goes to zero after $x\ge 1.0$. Fig. 9 presents the variation of stress ${\sigma }_{xx}(x,y,t)$ with respect to $x$ in which we notice that the magnitude of the stress increases to maximum value within the range $0\le x\le 0.5$, then decrease and finally converge to zero. The rotation has decreasing effect on the magnitude of ${\sigma }_{xx}(x,y,t)$. Fig. 10 exhibit the variation of ${\sigma }_{yy}(x,y,t)$ with respect to $x$ for $\mathrm{\Omega }=\mathrm{0.5,0.8}$ on the plane $y=0$. It is seen that the magnitude of the stress ${\sigma }_{yy}(x,y,t)$ starts with a maximum value and then decrease to zero with the increase of the distance $x$. Rotation has a decreasing effect on the magnitude of ${\sigma }_{yy}(x,y,t)$.

Figs. 11-16 display three-dimensional distributions of the non-dimensional temperature $\theta (x,y,t)$, horizontal displacement $u$, vertical displacement $v$ and the stresses ${\sigma }_{xx}(x,y,t)$${\sigma }_{yy}(x,y,t)$ and ${\sigma }_{xy}(x,y,t)$ for wide range of $x(0.0\le x\le 3.0)$ and $\nu (0.1\le \nu \le 1.0)$ for $t=0.1,y=0,M=2.5$. We have noticed that, the increasing of the value of the parameter $\nu$ causes increasing in the speed of the waves propagation of all the studied fields and it vanishes more rapidly.

6. Concluding remarks

The results of the present work present the fractional order generalized magneto-thermoelasticity in a rotating elastic media as a new improvement and progress in the field of generalized thermoelasticity. According to this theory, we have to construct a new classification to all the materials according to its fractional parameter where this parameter becomes new indicator of its ability to conduct the thermal energy. We have seen that the fractional parameter has a decreasing effect on the magnitudes of all the considered physical variables.