Electromagnetic crosstalk characteristics of micron parallel interconnects

2.1. Basic principle of parallel crosstalk interconnection model

As shown in Fig. 1, the interconnection principle model of crosstalk structure was presented. The two interconnection1 and interconnection2 were called interference lines and disturb lines respectively. When the signal passed through the interference lines, crosstalk signals would be generated at both ends of the interference lines. The one end of the interference line which was closer to the interference source was called the “near end”, which is closer to the interference source. And the other end of the interference line was called the “far end”, and the crosstalk generated at the two ends was called the proximal crosstalk and the distal crosstalk respectively.

The crosstalk was shown in Fig. 1 of the capacitive coupling and sensory coupling interference of the excitation signal of the interference line on the interconnect 2. The crosstalk was calculated according to Ohm's law, so there was:

Capacitive current was formed by capacitive coupling between two interconnects:

Fig. 1Crosstalk equivalent circuit diagram of two parallel interconnects

As shown in Fig. 1, there were two independent branch currents from the capacitive coupling current on interconnecting 2:

If Eqs. (3) and (2) were substituted into Eq. (1) to get the computing formula:

2.2. Analysis of distributed RLGC model parameters

Normally, when the low frequency signal was transmitted, the distribution parameters of interconnects need not be considered; When high frequency signal was transmitted or being radiated by high frequency and high energy signals (the frequency was about 30 MHz or more, including microwave frequency band), the electromagnetic effect of parallel crosstalk interconnects would generate circuit distribution parameters, as shown in Fig. 2. The equivalent circuit models of lossless crosstalk coupled interconnect were shown in the follow.

Fig. 2Two equivalent circuit models of lossless crosstalk coupled interconnects

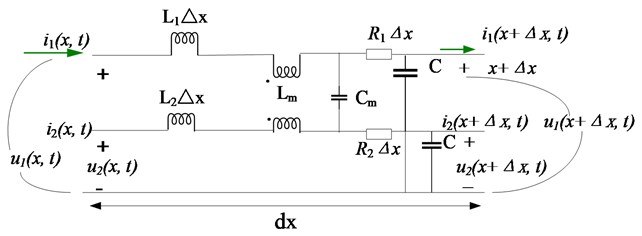
According to Kirchhoff’s law of voltage, the DC resistance voltage divider in high frequency range was neglected. The voltage equations of metal interconnect 1 and interconnect 2 of substrate was as follows:

Similarly, by applying Kirchhoff’s current law, it could be seen that the DC resistance voltage divider in the high frequency range was neglected, and the current equations of the metal interconnects 1 and 2 on the substrates were as follows:

where, $L$ was the unit length inductance, $L_m$ was the unit interconnection coupled inductance, $C$ was the unit capacitance of interconnects, $C_m$ was the unit length coupling capacitance of between interconnects. Then the Eqs. (6-9) could be written in matrix form respectively:

Then:

Then:

If the differential of the Eq. (9) and the Eq. (10) was calculated on both sides, there would have the Eq. (11):

So all the analytic results indicated that the reasonable equivalent circuit could substantially show the impacts of these parasitic capacitances, the attenuation of signal, the noise signal and coupling signal.

3.1. Near and far end of crosstalk strength structure interconnects

When the spacing $D$ of two interconnects was adjusted to 1$W$, 3$W$, 5$W$ and 7$W$, and other parameters remained unchanged, and the crosstalk intensity varied with the spacing of two parallel interconnects as shown in the Fig. 4 and Fig. 5.

Fig. 4Crosstalk intensity varying with two parallel interconnects spacing

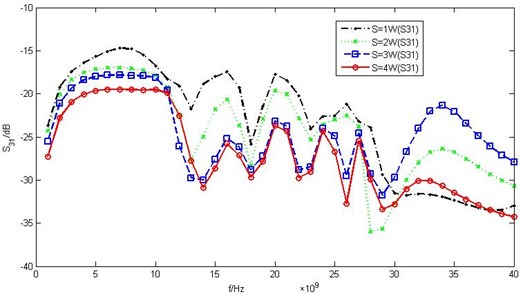
It could be showed from both charts that a linearly increasing trend was increased of the intensity of near-end crosstalk $S_{31}$ and far-end crosstalk $S_{41}$ at low frequencies. When the frequency exceeded 10 GHz, there were periodic oscillations with the increase frequency, and the rapid rise of far-end crosstalk $S_{41}$ was the main factor.

At the same time, when the interconnect spacing increased, the trend of crosstalk change remained unchanged, but the intensity of crosstalk decreased gradually. Obviously, when the line spacing exceeds three times the line width, the far-end crosstalk and the near-end crosstalk do not change much. This showed that the line spacing was no longer the main factor affecting crosstalk, so the interconnect spacing should be increased to three times as much as possible in the design.

Fig. 5Physical test of crosstalk intensity varying with interconnect spacing

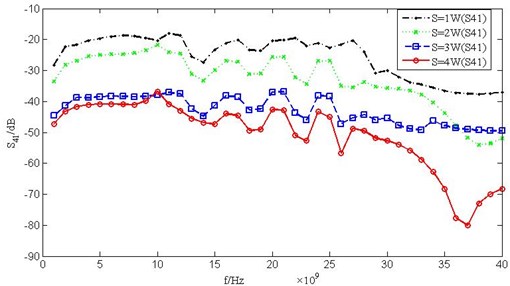
Fig. 6Crosstalk intensity varies with interconnect length

a) Near-end crosstalk S31 varies with interconnect length

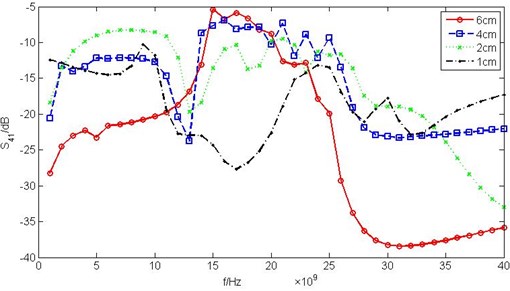
b) Far-end crosstalk S41 varies with interconnect length

3.2. Crosstalk intensity varied with interconnect length

As the interconnect width $W=$100 um and the interconnect spacing $S=$ 100 um, the crosstalk intensity would change as the interconnect length $L$ was 1 cm, 2 cm, 4 cm and 6 cm, respectively, as shown in the Fig. 6(a) As could be seen from Fig. 6(b), the crosstalk intensity increased with the length of interconnect, and for all length of interconnect, with the increase of frequency, the crosstalk intensity presented a “periodic oscillation” characteristic.

In addition, it could be seen from Fig. 6(a) that the longer the interconnect, the higher the oscillation frequency of the near-end crosstalk; from Fig. 6(b), it could be seen that the longer the interconnect, the greater the oscillation amplitude of the near-end crosstalk. Therefore, planning the design of RF circuits, shorter routes should be used to minimize the length of routes.