Vibration diagnostics of transportation structures on railroads

2.1. Materials and equipment

Vibrodynamic software and hardware complex (VPAK) is designed for dynamic testing of structures of artificial structures and serves for measuring dynamic characteristics of bridges under the impact of rolling stock, as well as small impulse impact (Fig. 1). The VPAC consists of: protected industrial laptop with software for data processing and visualization “DYNAMIK” (1); rechargeable battery with capacity of 1200 mAh (2); measuring voltage converter E-14-440D (3), designed to measure DC and AC voltage, for input, output and processing of analog and digital information in measuring devices and systems; connecting shielded cables 50 m long (4); set of seismometers (5) EP-300-compact (three-component seismometers with a liquid calming device) for determination of natural vibration frequencies and amplitude-frequency characteristics (AFC) of bridge spans in vertical (Z), horizontal-transverse (Y) and horizontal-longitudinal (N) directions.

Fig. 1General view of the vibrodynamic hardware-software complex

2.2. Methods of investigation of dynamic effects on girder spans of railway bridges

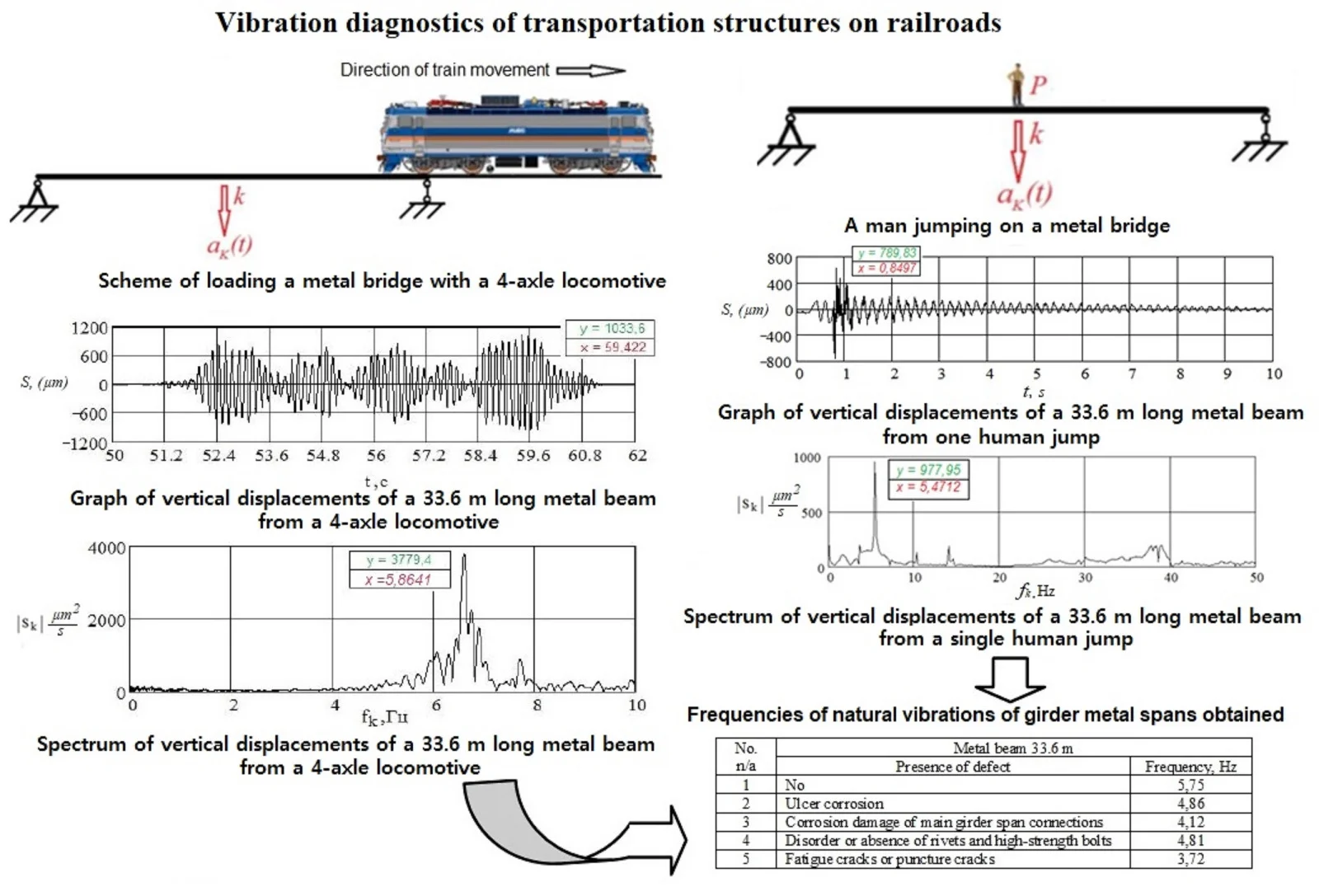
The objects of research were railroad bridges: metal girder bridges 36.6 m (Fig. 2), type of bridge deck – on wooden bars; reinforced concrete girder bridges 6+2×9.4+6 m, type of bridge deck – on crushed stone ballast.

2.3. Results of determination of dynamic characteristics of girder spans of railway bridges

Of particular interest in this research are both functions and amplitude Fourier spectra [17, 18] obtained from the impact on the bridge span structure as a result of one person jumping in the middle of the span.

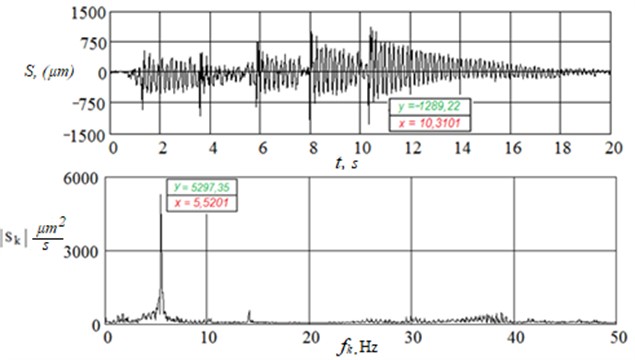
As an example, the amplitude-time and amplitude-frequency dependences of vibrations of the girder metal span of the railroad bridge with a span of 33.6 m across the Sarybulak River, obtained under a small impulse impact from a single jump of a person weighing 80-90 kg in Fig. 3, from five jumps in Fig. 4, where $S$ – displacement, µm; $t$ – time, s; $\left|S_k\right|$ – modulus of the spectral density of displacement, (µm)/s; $f_k$– frequency, Hz [4].

Fig. 2Scheme of the metal bridge span and location of seismometers of the Sarybulak River: 1 and 2 – seismometers installed in the middle of the span; P – load from one person (impulse – jumping)

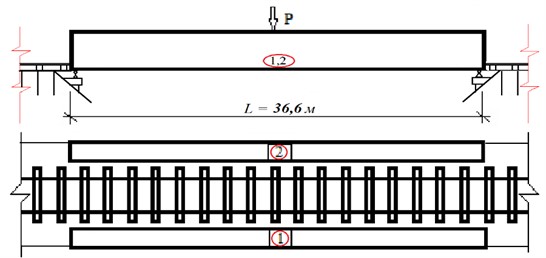
Fig. 3Vertical displacement graph and vertical displacement spectrum in the middle of a 33.6 m metal beam span from a single human jump

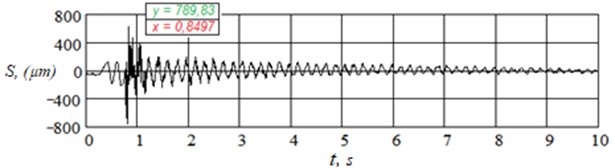
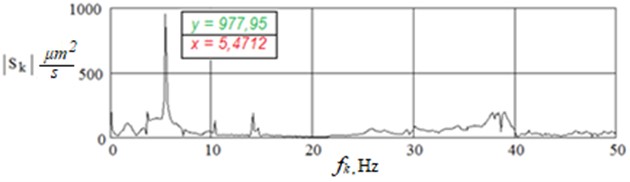
Fig. 4Vertical displacement graph and vertical displacement spectrum in the middle of a 33.6 m metal beam span from five human jumps

2.4. Determination of the relative damping coefficient of metal and reinforced concrete girder bridge spans

In dynamic calculations, the shapes of spectral curves in the region of resonances allow us to determine such a characteristic of the structure as the modal relative damping coefficient. Recall that the relative damping coefficient is equal to the ratio of the real damping to the critical damping.

At present, the relative damping coefficient, rather than the logarithmic decrement of oscillations, is regulated and used in the normative documents on the calculation of structures for seismic effects.

The relative damping coefficient $\xi$ when performing experimental work is determined by the width of the spectral resonance curve at the points of half energy (Fig. 5) using the formula:

Fig. 5Example of resonance curve with indication of points of half energy

This parameter is the modal damping coefficient corresponding to a given frequency and shape of natural oscillations. The frequency interval between half-energy points is often referred to as the width of the system spectrum. The width of the interval between half-energy points determines the property of the system to dissipate energy during oscillations. An increased width of the interval between half-energy points can indicate the presence of cracks in concrete, although this is not the only reason [22].

For example, the relative coefficient for reinforced concrete beams without cracks is 3 %, while in the presence of cracks it is 5-7 %. For a metal beam, a wide spectrum in the resonant frequency region of 7 % was obtained, which indicates that energy dissipation occurs not only in the span structure (in the metal and in the connections of the beam elements), but also in the interaction with the supports and the track structure: $\xi =\frac{\mathrm{\Delta }f}{2\bullet f_0}=\frac{\mathrm{1,05}}{2\bullet \mathrm{7,08}}=\mathrm{0,07}$. More often than not, $\xi$ for steel girder vibrations does not exceed 3 % [23].

2.5. Analysis of natural frequencies of vibrations of girder spans under impulse impact of small masses

The experimental data obtained from the testing of the 36.6 m span metal girder is presented in Table 1.

As a result of the analysis of the conducted tests it was revealed that under the impact of small masses (human jumps) on the girder metal span structure of the bridge over the Sarybulak river, the frequencies of natural vibrations of this span structure are in the range $f_m=$ 5,47-5,52 Hz. These frequencies determine the periods of natural vibrations of metal girder bridge spans, which are standardized by SP [24].

Experimental data obtained during testing of 6 m and 9.4 m reinforced concrete girder spans are given in Table 2.

As a result of the analysis of the conducted tests, it was determined that under the influence of small masses on the reinforced concrete girder bridge spans, the natural frequencies were in the range: for 6 m girders $f_{rc}=$13,29-15,06 Hz, and for 9.4 m beams $f_{rc}=$14,08-15,05 Hz. These frequencies determine, first of all, the period of natural vibrations of a reinforced concrete girder bridge span.

The analysis of the experimental data characterizing the response of the structure to the impact of small masses (human jumps) allowed us to establish the parameters of free vibrations of unloaded spans. The predominant frequencies on the dispersion spectral density plot are identical regardless of the number of jumps, i.e. the frequency of impact, if the time between jumps corresponds to the complete damping of vibrations.

The analysis of experimental data characterizing the response of bridge structures allowed us to establish the parameters of natural vibrations of unloaded spans [25-27].

According to the natural vibrations of girder spans of railroad bridges from a small impulse impact and from the impact of any passing trains, it can be concluded that the frequencies of natural vibrations of structures are close in numerical values. The same situation is observed when determining the relative damping coefficient. Discrepancies between quantitative values do not exceed 5 % for natural frequencies and 7 % for the relative damping coefficient, which is quite acceptable for this kind of research. The results of vibrodiagnostics based on measurements from small pulse impact are close in values to the results obtained in vibrodiagnostics from the impact of any moving load.

Vibrograms of natural vibrations are recorded using special highly sensitive seismometers, which are part of the measuring and computing complex for dynamic testing of structures, installed in the middle of the span on the upper (or lower) chord of one of the metal beams. Decrease of natural frequencies of vibrations can serve as an indicator of technical condition of a structure, which is confirmed by in-situ measurements of vibration parameters of more than 28 artificial structures.

Table 3 shows the results of measurements of natural frequencies of vibrations of metal girders of span structures obtained experimentally. The frequencies are determined for defect-free and defective girder spans.

Further, the results of measurements of natural frequencies for defect-free and defective girder metal and reinforced concrete spans of railroad bridges are given (Table 4), frequencies were determined for two values, before defect elimination – increase of ballast layer under sleeper 0.65 m and after, at normal thickness of ballast under sleeper 0.25 m.

Of practical interest in the assessment of the technical condition is the quantitative assessment of the change in the natural frequency of vibration of reinforced concrete and metal spans depending on the presence of defects accumulated during the operation of the bridge.