An approximate solution of the effective moduli on the composite thin-walled beams

2.1. The model and the equilibrium equations of the composite thin-walled beam

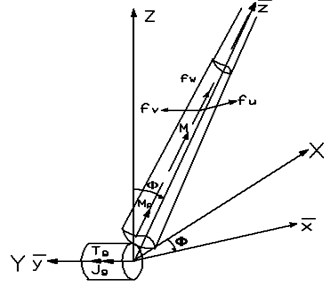
According to the principle of conservation of energy, ignoring the coupling terms, the kinetic equations [12] of the composite thin-walled beam are as follows:

Fig. 1The model of the composite thin-walled beam

where, $U_1$, $U_2$ and $U_3$ are the average displacement in $x$, $y$ and $z$ directions. $\phi$ is the average displacement in twisting direction. The composite stiffness is as follow:

The inertial parameters of the composite thin-walled beam are defined in Eq. (6):

The coefficients of Eq. (5) are defined as follows:

In Eq. (8), $h_k$ and $h_{k-1}$ are the upper and lower surface coordinates respectively in the $k$th lamina. $N$ is the number of layers. [$Q_{ij}]=[T\left]T\right[Q_{ij}\left]\right[T]$ is the erosive eccentric plane stress stiffness matrix. $\left[Q_{ij}\right]$ ($i$, $j=$1, 2, 6) is the erosive orthoaxis plane stress stiffness matrix. The elements in matrices are as follows:

The transformation matrix can be defined in Eq. (10):

2.2. Solution of natural frequencies

The boundary conditions on the cantilever beam are as follows:

It is defined as following:

The general solution of the torsional vibration in Eq. (2) are as follows:

The Eq. (14) can be gained from the boundary conditions in Eq. (11):

The equation of solving the natural frequency can be gained from the second term of the Eq. (14):

The natural frequencies of the torsional vibration can be obtained from the Eq. (16):

The Eq. (14) is substituted in the Eq. (16). Then the torsional natural frequencies are gained from the Eq. (17):

The exprestions and the boundary conditions in longitudinal vibration are the same as those in torsional vibration. Then the longitudinal natural frequencies can be calculated by the the Eq. (18):

The boundary conditions on the cantilever beam in $y$ direction are as follows:

The general solution of the Eq. (4) are as follows:

The Eq. (19) is substituted in the Eq. (20), then the Eq. (21) can be gained:

The natural frequency can be solved, after the Eq. (21) is simplified:

The first four roots of the Eq. (22) are as follows:

when $i\ge 3$, the values are as follows:

The natural frequencies in $y$ direction are obtained in Eq. (25):

The equations and the boundary conditions in $z$ direction are the same with those in $y$ direction. Then the natural frequencies in $z$ direction can be gained as followings:

2.3. Natural frequencies on composite thin-walled beams

The characteristic parameters of the composite thin-walled beams are gained from the reference [13], so the natural frequency can be compared and analyzed. The parameters are $h_0=\text{0.635}\text{mm,}$${\rho }_0=\text{1672}\text{kg/m}\text{3}\text{,}$$E_{11}=\text{25.8}\text{G}\text{p}\text{a}\text{,}$$E_{22}=\text{8.7}\text{G}\text{p}\text{a}\text{,}$$G_{12}=G_{23}=\text{3.5}\text{G}\text{p}\text{a}\text{,}$${\nu }_{12}=$0.34.

The Table 1 shows the natural frequencies of the composite thin-walled box beams. The width of the box beams is $b=$0.32 m, the width-height ratio is $b/a=$5, the length-width ratio is $L/b=$14.37. The thickness of the composite laminate is $h=$10.16 mm. The Table 2 shows that the width-height ratio has effect on the natural frequencies of the composite thin-walled box beams. The Table 3 shows that the natural frequencies of the composite thin-walled box beams will change with different length-width ratios. The natural frequencies of the composite thin-walled circular beams are listed in the Table 4, in which the diameter is $d=$352 mm, the length-diameter ratio is $L/d=$26, and the thickness of the composite laminate is $h=$10.16 mm. The Table 5 shows that the natural frequencies of the composite thin-walled circular beams will vary in the length-diameter ratios. All calculation results of the natural frequencies are compared with that in reference [13]. The numerical deviations are relatively small, so the model and the equilibrium equations can be used to calculate approximately the natural frequencies on the thin-walled composite beam.

3.1. Establishment and solution of the equivalent beam

The equivalent beam is the same with the composite thin-walled beam in the length, the cross section shape and the linear density. It has the independent shear modulus and the orthotropic elastic moduli in $x$, $y$ and $z$ directions. The expressions of the equivalent beam are as follows:

where, $E_x$, $E_y$ and $E_z$ are the orthotropic elastic moduli in $x$, $y$ and $z$ directions. $G$ is the shear modulus of the equivalent beam. $A$ is the cross-sectional area of the equivalent beam. The density of the equivalent box beam is as follows:

The density of the equivalent circular beam is caculated in Eq. (29):

The principle moments of inertia on the equivalent box beam in $y$ and $z$ directions are as follows:

The principle moments of inertia on the equivalent circular beam in $y$ and $z$ directions are as follows:

By using the classical beam theory, the torsional natural frequencies of the cantilever beam are obtained in Eq. (32):

The longitudinal natural frequencies of the equivalent beam are obtained as followings:

The natural frequencies of the equivalent beam in $y$ direction are as follows:

Natural frequencies on the equivalent beam in $z$ direction are obtained as followings:

The paremeters in Eqs. (34) and (35) are calculated:

The effective moduli can be gained by the contrastive analysis of the composite thin-walled beam and the equivalent solid beam. They are only applied to an approximate dynamic analysis on the thin-walled composite beams. The approximate calculation equations are as follows:

3.2. Numerical analysis on the effective moduli

The parameters of the composite thin-walled box beam in Table 6 are chosen as follows. The width is $b=$ 0.32 m. The length-width ratio is $L/b=$14.37. The thickness of the composite laminate is $h=$10.16 mm. The effective moduli on the composite thin-walled box beams are presented in Table 6. When the width-height ratios decrease, the elastic moduli in $x$, $y$ and $z$ directions become small under the layer styles of [0]₁₆ and [90]₁₆, but the shear modulus increases. With the decreasing width-height ratio ratios, the elastic moduli in $x$, $y$ directions and the shear modulus become small under the layer style of [0₂/90₂/45₂/–45₂]_s, but the elastic modulus in $z$ direction increases. When the width-height ratios become small, the elastic modulus in $x$ direction and the shear modulus decrease under the layer style of [45/–45]₈, the elastic modulus in $z$ direction increases, and the elastic moduli in $y$ direction decreases at first, then increases.

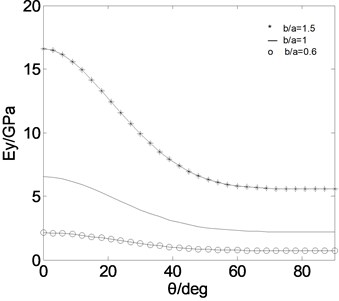
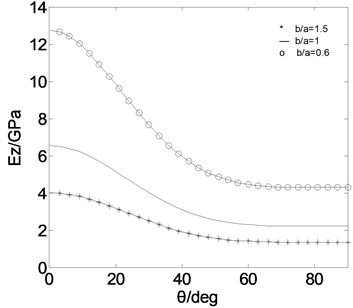
For a CUS configuration, the layer style of skin laminations at the left, right, top and bottom side are all [$\theta$]₁₆, For a CAS configuration, the layer styles of skin laminations are as follows. [$\theta$]₁₆ at top flange, [–$\theta$]₁₆ at bottom flange, and [$\theta$/–$\theta$]₈ at left and right web. Fig. 2 and Fig. 3 show the effective moduli on the composite thin-walled box beams under the CUS and CAS configuration.

The elastic moduli on the composite thin-walled box beams decreases with increasing ply angels in Fig. 2. When the width-height ratios become small, the elastic moduli in $x$ and $y$ directions decrease, but the elastic modulus in $z$ direction increases. Table 6 also shows, when the ply angle comes to zero, the elastic modulus is maximal. In order to improve the elastic moduli of the composite thin-walled beams, adding the ply thickness, adopting small ply angle and large width-height ratio are all good choices.

Fig. 2(d) shows, when $\theta =$ 38° the shear modulus on composite thin-walled box beams is maximal, and becomes small with increasing width-height ratios. Table 6 also shows, when the layer style of skin laminations is [45/–45]₈, the shear moduli is maximal, and is higher than that under CUS configuration. For improving the shearing modulus on the composite thin-walled beams, we can add the ply thickness and the width-height ratio, or adopt the layer style [45/–45]₈. Fig. 3 shows, the effective elastic moduli on the composite thin-walled beams under the CAS and CUS configuration are in substantial agreement. The difference of the shear modulus is obvious.

Fig. 2Effective moduli on composite thin-walled box beams (CUS)

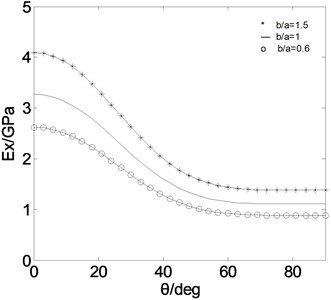
a)

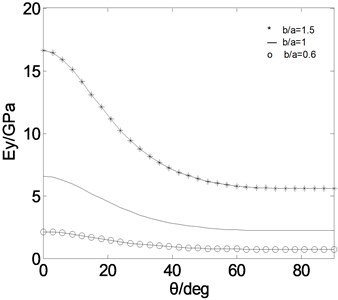
b)

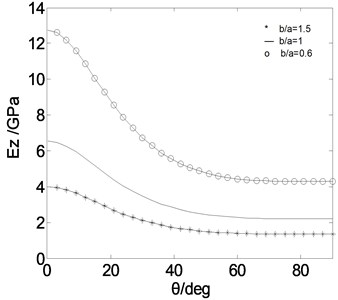
c)

d)

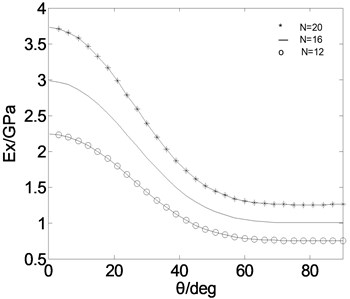
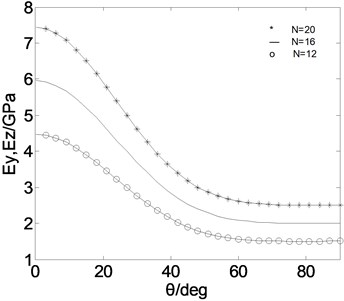
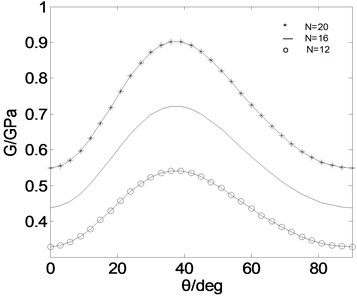
Table 7 shows the different effective moduli under different layer styles when $N=$20, 16, 12. Fig. 4 shows the effective moduli on the composite thin-walled circular beams under the CUS configuration when $N=$20, 16, 12. The parameters of composite thin-walled circular beams are chosen as follows: $d=$352 mm, $L/d=$26, $h_0=$0.635 mm.

Fig. 3Effective moduli on composite thin-walled box beams (CAS)

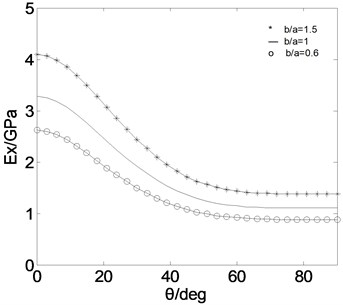
a)

b)

c)

d)

4.1. The example I

There are some expressions of wind turbine blades in reference [14]. Retention of the first and second order Taylor quantity, neglecting higher-order quantity and gravity, ignoring the aerodynamic parameters and the nonlinear terms, the motion equations in $x$, $y$ and $z$ directions can be gained in Eqs. (38), (39) and (40). The typical blade parameters are chosen for carrying out the calculation and analysis of the wind turbine blades. As the cross section of blades is universal, the rectangular blade is selected for numerical simulation. The variable parameters are as follows.

The pre-twist angle $\theta =\text{2}\pi {(s-\text{60})}^2\text{120/360}\text{,}$ the chord length $c=\text{4}-\text{3}s\times \text{2.8/180}\text{,}$ thickness of the blade $b=\text{0.25}c$. $s$ is the distance from the center of rotation. And the pitch angle $\beta =\text{0.035}\text{rad,}$ length of the blade $R=\text{60 m}\text{,}$ rotating speed $\mathrm{\Omega }=\text{0.8 rad/s}\text{.}$ The composite parameters are $h_0=0\text{.635}\text{mm,}$${\rho }_0=\text{1672}\text{kg/m}\text{3}\text{,}$$E_{11}=\text{25.8}\text{G}\text{p}\text{a}\text{,}$$E_{22}=\text{8.7}\text{G}\text{p}\text{a}\text{,}$$G_{12}=G_{23}=\text{3.5}\text{G}\text{p}\text{a}$, ${\nu }_{12}=\text{0.34}$. The composite wind blade is calculated under a CUS configuration. The layer style of skin laminations at the left, right, top and bottom side are all [$\theta$]₉₆. The model of a composite turbine blade is in Fig. 5.

Fig. 4Effective moduli on composite thin-walled circular beams (CUS)

a)

b)

c)

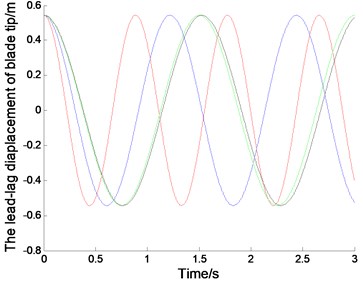
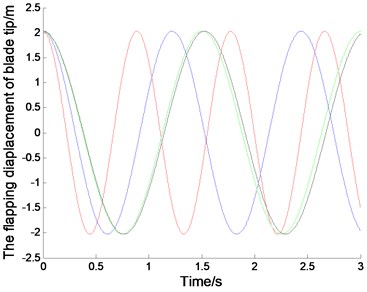
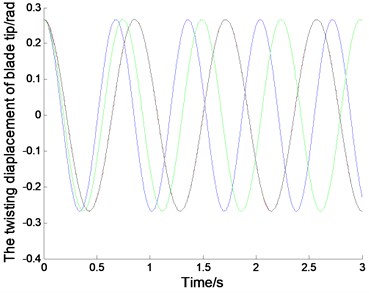
Fig. 6 represents the displacements of the composite blade in the directions of lead-lag, flapping and twisting. The initial displacements of blade tip are $u_6=$0.5432, $v_6=$2.0272, ${\phi }_6=$0.2673. The initial displacements of other node must be selected by the linear vibration mode for the displacement continuity of continuous bar. The simulation of linear system can be achieved by Matlab/Simulink presented in [16-18]. The natural frequencies in Fig. 6 accord with those in Fig. 2. Much dynamic analysis on the composie blade can be made by the classic beam theory according to the approximate solution of the effective moduli:

Fig. 5The model of a composite turbine blade

a)

b)

Fig. 6The displacements of a composite thin-walled box blade (CUS) (the red represents θ= 0°, the blue represents θ= 30°, the green represents θ= 60°, the black represents θ= 90°)

a)

b)

c)

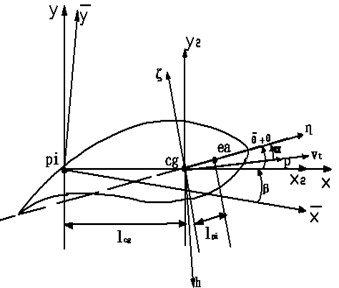
4.2. The example II

The model [15] of a composite thin-walled spinning beam was established in Fig. 7. Neglecting the torsional terms, the two-dimensional motion equations can be gained in Eq. (41). The chosen parameters are: the diameter $d=$352 mm, the length-diameter ratio $L/d=$26. The composite parameters of the circular beam are $h_0=$0.635 mm, ${\rho }_0=$1672 kg/m³, $E_{11}=$25.8 Gpa, $E_{22}=$8.7 Gpa, $G_{12}=G_{23}=$3.5 Gpa, ${\nu }_{12}=$0.34. The composite thin-walled circular beam is calculated under a CUS configuration. The layer style of skin laminations at the left, right, top and bottom side are all [$\theta$]₆:

Fig. 7The model of a composite thin-walled spinning beam

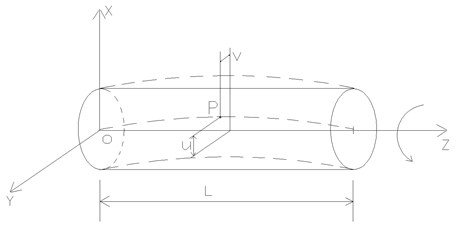
Fig. 8 represents the radial displacements of a composite spinning thin-walled circular beam, when the spinning speed $\mathrm{\Omega }=$0,200 rpm. The initial displacements of blade tip are $u_6=v_6=$0.0727. The initial displacements of other node must be selected by the linear vibration mode for the displacement continuity of continuous bar. The simulation of linear system can be achieved by using a finite difference method [19-20].The natural frequencies in Fig. 8 are agree with those in Fig. 4. More dynamic analysis on the composie beam can be made by the classic beam theory.

Fig. 8The displacement of a composite spinning thin-walled circular beam (CUS) (the red represents θ= 0°, the blue represents θ= 30°, the green represents θ= 60°, the black represents θ= 90°)

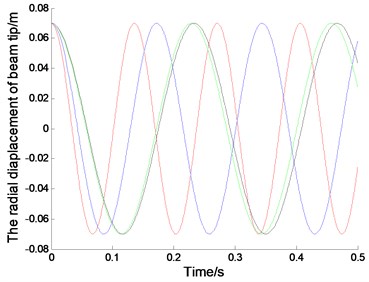
a) $\mathrm{\Omega }=$0 rpm

b) $\mathrm{\Omega }=$ 200 rpm