Non-linear behavior of a Z-source DC/DC converter based on dual-loop control

1. Introduction

As a new type of power converter, a Z-source converter, which can achieve single-stage buck/boost conversion, has been developed [1]; this converter can be potentially applied in the field of new-generation energy because of its wide input voltage range [2-3]. For instance, the Z-source converter switches periodically under the influence of a control system, causing changes in system topology periodically; the voltage and current of a system then change between different stable points. Each kind of topology corresponds to a linear system, but this system is generally a piecewise non-linear system. This characteristic results in a complex nonlinear dynamics behavior of physical quantities, such as bifurcation and chao of a system. These phenomena adverselyaffect the work performance of a system, particularly the critical state of sudden collapse, electromagnetic noise, unknown instability. Thesephenomena are external manifestations of the inherent non-linear behavior of a Z-source converter [4]. A converter functions in a chaotic state and likely results in an unpredicted and uncontrolled system; as such, the performance of this converter is affected seriously. In some cases, a converter is completely unable to function. This condition causes great difficulty in the design and control of a system. Therefore, non-linear dynamics theory can be applied to analyze and understand the non-linear process and characteristics of a system. In this way, engineering practice can be effectively performed.

These complex non-linear phenomena in power electronic systems have prompted many scholars to perform several studies. In the past 20 years, many studies were conducted on the theory of non-linear dynamics, numerical simulation, and circuit experiment to analyze bifurcation and chaos in this kind of systems. These studies have observed quasi-periodic bifurcation, period doubling bifurcation, border collision bifurcation, intermittent bifurcation, chaos, and other non-linear phenomena [5-8] in a DC/DC converter [9-11], DC/AC inverter [12-13] and power factor correction converter (PFC) [14]. Another example is the Z-source converter that exhibits a unique shoot-through stateand achieves single-state buck-boost converting. The non-linear behavior of this converter is more complex than that of traditional inverters. In anotherstudy, the bifurcation and chaos of a Z-source converter under peak-current control (single-loop) [15]. However, dual-loop control can achieve multi-object control simulaneously; as such, dual-loop control has been widely used. In the present study, a Z-source converter under dual-loop control was used as our object, on the basis of the equation of state, the non-linear behavior of the Z-source converter was modeled using a stroboscopic map method, simulated and analyzedto determine the bifurcation and chaos.

2. Principle of Z-source DC/DC converter

Fig. 1 illustrates the circuit diagram of a Z-source DC/DC converter based on current-mode dual-loop control.

In Fig. 1, a two-port network consisting of the split inductors $L_1$ and $L_2$ and the capacitors $C_1$ and $C_2$ connected in an $X$ shape manner is used, to analyze this diagram, we assume that $L_1=L_2=L$ and $C_1=C_2=C$.

Fig. 1Schematic of the Z-source DC/DC converter based on current-mode dual-loop control

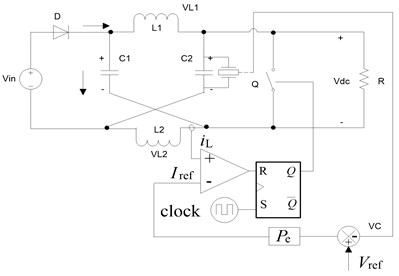
The schematic of a Z-source DC/DC converter based on dual-loop control is shown in Fig. 1, where $V_{in}$ is the input voltage, $V_{ref}$ is the reference voltage, and $P_e$ is the error gain of the output voltage. This system mainly involves a clock signal that controls the turn-on switch. This clock signal also sets the RS flip-flop to 1 at regular intervals. At $Q=$0, the switch is turn-off, and the inductor current $i_L$ increases until the required $I_{ref}$ is reached. Afterward, the inductor inverts the output signal of the at $Q=$ 1. The current reference $I_{ref}$ is produced by amplifying the error between $V_{ref}$ and the actual output voltage. Current-mode dual-loop control can be performed easily and limits the peak current to protect device; therefore, this system can be used in several applications.

On the basis of the shoot-through switch $Q$ states and the diode $D$ states, we can obtain the two working modes (Fig. 2).

Fig. 2DC-link equivalent circuit of the Z-source converter

a) Equivalent circuit in shoot-through mode

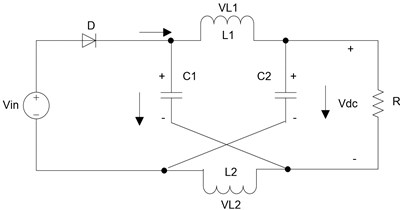
b) Equivalent circuit in non-shoot-through mode

In working mode 1, the shoot-through state is represented as $Q$ on and $D$ off; the state equation is written as follows:

In working mode 2, the non-shoot-through state is represented as $D$ off and $Q$ on; the state equation is expressed as follows:

The state equation derived from Eqs. (1) and (2) is specified as Eq. (3) and Eq. (4), respectively:

where the state vector $\dot{x}$ is defined as $x=\left[\begin{array}{l}{i}_L\\ u_c\end{array}\right]$, $i_L$ is the inductor current of the Z-source network, $u_c$ is the capacitance voltage of the Z-source network, $r_1$ is resistor parasitics of the inductor, $R_1$ is the series resistance of capacitance, $R$ is the load resistance, and $V_{dc}$ is the output voltage:

3. Simulation of the non-linear behavior of a Z-source DC/DC converter

The simulation results (Figs. 3-5) showed that $U_c$ is the capacitance voltage of the Z-source network and $i_L$ is the inductor current of the Z-source network. These figures show the typical waveforms of period-1 state, period-2 state, and chaotic state.

Fig. 3Typical waveforms of period-1 state

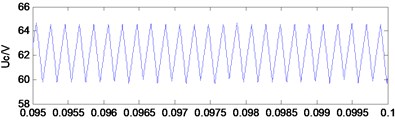
a) Capacitance voltage

c) Phase-plane diagram

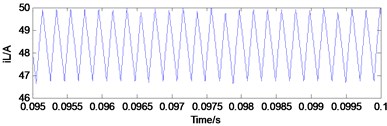
b) Inductor current

4. Dynamic modeling and analysis of a Z-source DC/DC converter

4.1. Stroboscopic mapping model

$d_n$ is the duty cycle of the $n$th period $T$, where $t_n=nT$, $x_n=x\left(nT\right)$; other discrete parameters are defined by similar logic in which the state Eq. (3) and Eq. (4) as well as the discrete model Eq. (6) and Eq. (7) in the nth period are calculated. The discrete mapping equation can be expressed as follows:

5

$x_{n+1}=f\left(x_n,d_n\right),$

6

$x(t_n+d_{n}T)={\varphi }_1\left(d_{n}T\right)x\left(t_n\right)+{\int }_{t_n}^{t_n+d_{n}T}{\varphi }_1(t_n+d_{n}T-\tau )B_1{V}_{in}d\tau$
$\mathrm{}\mathrm{}\mathrm{}\mathrm{}\mathrm{}\mathrm{}={\varphi }_1\left(d_{n}T\right)x\left(t_n\right),\mathrm{}\mathrm{}\mathrm{}$$nT+t_n\le t<nT+d_{n}T),$

7

$x\left(t_{n+1}\right)={\varphi }_2\left({\stackrel{-}{d}}_{n}T\right)x(t_n+d_{n}T)+{\int }_{t_n+d_{n}T}^{t_n+T}{\varphi }_2(t_n+T-\tau )B_2{V}_{in}d\tau$
$\mathrm{}\mathrm{}\mathrm{}\mathrm{}\mathrm{}\mathrm{}={\varphi }_2\left({\stackrel{-}{d}}_{n}T\right)x\left(t_n+d_{n}T\right)+{\int }_0^{{\stackrel{-}{d}}_{n}T}{\varphi }_2\left({\stackrel{-}{d}}_{n}T-\tau \right)B_2{V}_{in}d\mathrm{\tau },\mathrm{}\mathrm{}\mathrm{}\left(nT+d_{n}T\le t<\left(n+1\right)T\right),$

where ${\varphi }_1\left(d_{n}T\right)=e^{A_1{d}_{n}T}$, ${\varphi }_2\left({\stackrel{-}{d}}_{n}T\right)=e^{A_2{\stackrel{-}{d}}_{n}T}$, ${\stackrel{-}{d}}_n=1-d_n$.

Fig. 4Typical waveforms of period-2 state

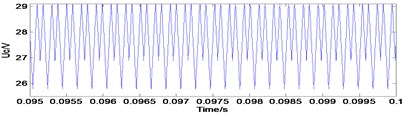
a) Capacitance voltage

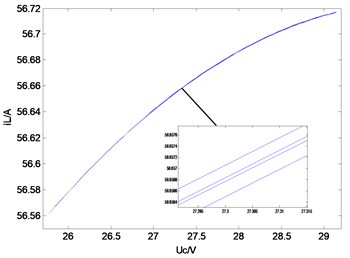
c) Phase-plane diagram

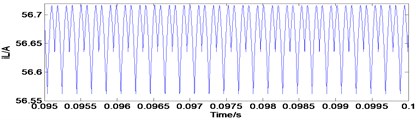
b) Inductor current

Fig. 5Typical waveforms of choatic state

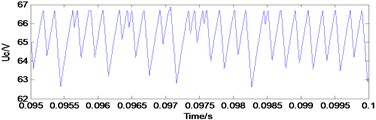
a) Capacitance voltage

c) Phase-plane diagram

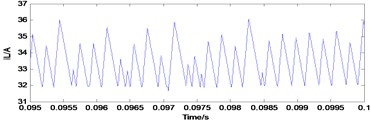
b) Inductor current

$A_1$ and $A_2$ are both invertible matrixes; as a result the integral terms of these equations can be simplified and expressed as follows:

8

$x\left(t_n+d_{n}T\right)={\varphi }_1\left(d_{n}T\right)x_n,$

9

$x\left(t_{n+1}\right)={\varphi }_2\left({\stackrel{-}{d}}_{n}T\right)x\left(t_n+d_{n}T\right)+A_2^{-1}\left({\varphi }_2\left({\stackrel{-}{d}}_{n}T\right)-I\right)B_2{V}_{in}.$

Eq. (8) can be substituted in Eq. (9) and expressed as the discrete mapping Eq. (10):

10

$\begin{array}{c}{x}_{n+1}=f\left(x_n,d_n\right)={\varphi }_2\left({\stackrel{-}{d}}_{n}T\right){\varphi }_1\left(d_{n}T\right)x_n+A_2^{-1}\left({\varphi }_2\left({\stackrel{-}{d}}_{n}T\right)-I\right)B_2{V}_{in}.\end{array}$

The switching function can be derived as follows:

11

$\begin{array}{c}\sigma \left(x_n,d_n\right)=i_L-\left(V_{ref}-u_c\right)\cdot Pe=k_{1}x\left(t_n+d_{n}T\right)-\left[V_{ref}-k_{2}x\left(t_n+d_{n}T\right)\right]\cdot Pe\end{array}$
$\mathrm{}\mathrm{}\mathrm{}\mathrm{}\mathrm{}\mathrm{}=k_1{\varphi }_1\left(d_{n}T\right)x_n-\left[V_{ref}-k_2{\varphi }_1\left(d_{n}T\right)x_n\right]\cdot Pe,$

where $k_1=\left[\text{1,}\text{0}\right]\text{,}$$k_2=\left[\text{0,}\text{1}\right]\text{,}$ and $Pe=\text{100}$ at $\sigma (x_n,d_n)=\text{0;}$ as a result, the state of the converter is altered.

Eq. (10) and Eq. (11) can be simultaneously calculated as a non-linear discrete mapping equation of a Z-source converter.

5. Numerical simulation and result analysis

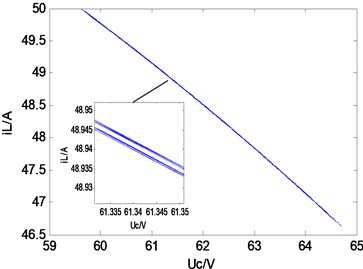
Our simulation is based on the state equations derived in Section 4. We investigated the dynamics of the state variable by using $V_{ref}$ as a bifurcation parameter. The circuit parameters used in our simulation are listed as follows: $C_1=C_2=C=\text{1,000}\text{μF}$, $L_1=L_2=L=\text{1}\text{mH}$, $V_{in}=\text{60}\text{V}$, $R=\text{6}\text{Ω}$, $R_1=\text{0.03}\text{Ω}$, $r_1=\text{0.5}\text{Ω}$, and $f=$10 kHz. We obtained a bifurcation diagram (Fig. 6). Fig. 6 summarizes $u_c$ at the beginning of each switching period as $V_{ref}$ increases.

Fig. 6Bifurcation diagram of uc

Fig. 6 also shows that the system exhibits a period-1 orbit at relatively low $V_{ref}$. This orbit indicates that the system is stable. This orbit should also be stabilized at a widened range. In the Z-source converter under dual-loop control, the period-1 orbit is intermittent which observed from the partially enlarged detail. As $V_{ref}$ increases, the period-2 orbit is observed, and the branch point is approximately $V_{ref}=\text{30}\text{V}$. As $V_{ref}$ increases, chaos occurs. Therefore, Fig. 6 illustrates the path from stability to chaos.

Fig. 7 shows the effect of variations in $V_{ref}$ on the stability of the Z-source converter. The system is initially stable, and the twoJacobian matrixeigenvalues are mapped in the unit circle. As $V_{ref}$ is gradually increased, one of the eigenvalues leaves the unit circle. This result indicates a period-2 bifurcation, rapidly results in instability and chaos. The eigenvalues are listed in Table 1.

Fig. 7Movement of eigenvalues for a dual-loop controlled Z-source converter

Table 1 shows that $\left|{\lambda }_1\right|$ is approximately equal to 1 at $V_{ref}=$30 V. On the basis of numberical calculations, we can obtain the following: at values exceeding $V_{ref}=$30 V, ${\lambda }_1=\text{0.8786,}$ and ${\lambda }_2=-\text{1.0020}$, where $V_{ref}$ is the critical value of the unstable system, the system experiences a bifurcated and chaotic state. At $V_{ref}<$30 V, eigenvalues < 1; as $V_{ref}$ increases, $\left|{\mathrm{\lambda }}_1\right|$ moves toward –1. This result indicates the occurrence of period-2 bifurcation; as $V_{ref}$ continuously increases, $\left|{\lambda }_2\right|$ moves toward 1 at $V_{ref}=$ 50 V, which happens jump. The two eigenvalues change along the real axis, and $\left|{\mathrm{\lambda }}_1\right|$ changes more rapidly than $\left|{\lambda }_2\right|$. If any of these eigenvalues is > 1, the system becomes unstable.

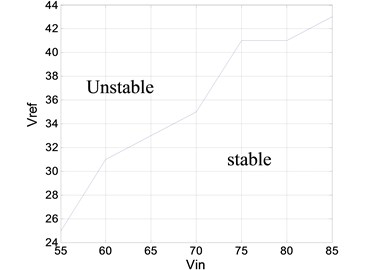
As the system is controlled in period –1, the boundaries of a stable region or an unstable region are presented in this section. $V_{in}$ and $V_{ref}$ are selected as the variations. The operation boundaries (Fig. 8) are derived from the analytical solutions and cycle-by-cycle simulation. The two results complement each other. The operation boundary provides essential design-oriented information in which system parameters are selected systematically.

Fig. 8Stable region boundary with altered parameters

6. Experiment verifications

To verify the theoretical analysis result, we established an experimental circuit prototype of the Z-source converter under dual-loop control (Fig. 9). The main circuit of the Z-source converter is shown in Fig. 9(a) and the control signal is generated using RT-lab equipment [Fig. 9(b)]. All of the parameters are similar to those in Section 4. The experimental waveforms in Fig. 10 are period-1, period-2, and chaos respectively. Fig. 10(a) shows stable period-1 at $V_{ref}=$25 V, Fig. 10(a) shows the stable period-1 at $V_{ref}=$25 V. Fig. 10(b) shows the stable period-2 at $V_{ref}=$32 V. Fig. 10(c) shows the chaos phenomenon. The experimental results are consistent with the theoretical analysis. These results also confirm the correctness of the theoretical analysis. In Fig. 10, the oscilloscope channel-1 displays the Z-source capacitor voltage $u_c$, and channel-2 shows the Z-source inductance current $i_L$.

Fig. 9Experimental platform

a) Main circuit

b) RT-lab controller

Fig. 10Experimental waveform

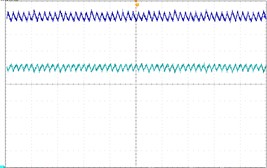
a) Period-1, $V_{ref}=$25 V

b) Period-2, $V_{ref}=$32 V

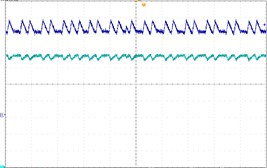
c) Chaos, $V_{ref}=$67 V

7. Conclusions

The Z-source converter can be potentially applied in emerging energy technologies and distributed generation. In this study, the Z-source DC/DC converter is used as the object under dual-loop control. The stroboscopic mapping model is established to analyze the non-linear behavior of the converter. Simulations and experimental results are presented for verification purposes. The researchers of this study expanded the non-linear research fields involving converters; the system provides an important theoretical basis and is of practical significance in engineering.