Influences of planetary gear parameters on the dynamic characteristics – a review

2.1. Mesh phase difference

Substantial researches [13-15, 18, 25] have been conducted on the mesh phase of PGT, which show that a mapping relationship exists between the phase matching and the excitation of PGT.

Jiang [16]studied the effect of the mesh phase of PGT on gear vibration and noise to better understand the application of mesh phase difference on the PGT. This application can reduce the gear vibration and noise levels, and these levels were less susceptible to the influence of the tooth surface mesh coefficient [16] and the tooth profile contact ratio [19, 26]. Chen and Ishibashi [19] designed and built an original PGT testing machine to examine how vibration and noise were affected by different contact ratios and meshing phase difference, and the testing results shown that the noise and vibration levels of PGT with a meshing phase difference can be further reduced by improving gear accuracy and tooth face roughness. Meanwhile, the PGT exists with combination of various mesh stiffness because of the mesh phase difference. This condition may lead to the PGT appearing to have a more dense frequency [17], and create a large difference for each planet gear vibration mode and an uneven load distribution. Thus, the reliability of PGT is reduced.

In the PGT, different mesh phase between the sun-planet and ring-planet mesh has powerful impact on the dynamic response and can have significant benefits in reducing vibration and noise[18, 19]. So a clear understanding of the relations governing the mesh phase is essential. Parker and Lin [13] clarified the mathematical relations for mesh phase for general PGT.

In the PGT, the planet gears are evenly distributed around the sun gear and each planet gear has the same parameters, the condition results in the periodic time-varying mesh stiffness of the sun gear. Through the design of the mesh phase difference, each planet gear acquires a different mesh phase and the effects of the excitation caused by mesh stiffness [20] can be reduced after superposition. Finally, the vibration can be neutralized or eliminated [21]. As shown in Fig. 2, a reasonable mesh phase difference was designed in PGT. Although the time-varying stiffness of each planet gear varies with the mesh periodic, the total mesh stiffness of PGT is a fixed value after the superposition.

Fig. 2Mesh stiffness of PGT [20]

a) Instantaneous mesh stiffness of sun-planets mesh

b) Total mesh stiffness of PGT after superposition

Therefore, the ultimate goal of designing the mesh phase difference is to change the time-varying mesh stiffness of the PGT. Finally, the vibration and noise are reduced.

2.2. Geometric errors

The effects of the geometric errors on the PGT dynamic characteristics have been studied extensively. Cheon and Parker [27] and James [28] studied the effect of manufacturing errors on the PGT dynamic characteristics. Typical errors of manufacturing errors, which include modulations include eccentricities and run-out errors of the gears and the carrier, and tooth-to-tooth spacing, tooth thickness and so forth, might cause modulations of PGT [29]. The combination of a series of incentive disturbance, which caused by the tooth profile errors, mounting errors, and deflections [30], cause the transmission error of PGT so that the vibration and noise are produced. The effects of the tooth profile errors, the eccentricities and the misalignments on the dynamic characteristics of the PGT are summarized in the follow paragraphs.

2.2.1. Tooth profile errors

Generally, tooth profile errors, which are induced by the manufacturing process, result in imperfections of the geometry of the tooth and create deviations from the perfect involute shape [31, 32]. As shown in Fig. 3, the solid lines show the actual tooth profile shapes and the dashed lines indicate the two closest tooth profile shape lines containing the actual tooth profile shape line.

Fig. 3Tooth profile errors [33]

The tooth profile errors may affect the contact quality of the tooth surface and cause the incorrect gear mesh, finally affect the transmission characteristic of the PGT. Moreover, the tooth profile errors can increase the gear mesh frequency amplitude. High values of these errors indicate poorly manufactured gears and tend to have dramatic consequences on the transmission [34]. A tooth profile can product an exciting force $F\left(t\right)$, which represents an amplitude modulation of the gear mesh stiffness signal $K_e\left(t\right)$ by the profile error signal $E_{sn}\left(t\right)$. It is expressed by:

1

$F\left(t\right)=E_{sn}\left(t\right)K_e\left(t\right),$

2

$E_{sn}\left(t\right)={\sum }_{m=1}^{\mathrm{\infty }}{\stackrel{-}{E}}_{sm}\mathrm{s}\mathrm{i}\mathrm{n}\left(2\pi m{f}_{e}t+{\xi }_{sn}\right),$

where ${\stackrel{-}{E}}_{sm}$ represents the profile error modulus, $f_e$ is the mesh frequency, ${\xi }_{sn}$ is the phase difference of sun-planets mesh and $m$ is the multiplicity of eigenvalues.

A previous study [24] suggested the tooth profile errors might be the cause of these variations in rattle amplitude. Another study [23] concluded that the composite profile errors of a gear pair had a stronger effect on the gear noise than the profile errors of a single gear. Moreover, the vibration noise of the gear mesh is correlated not only with the size of the shape errors but also with the direction of the tooth profile pressure angle error. At the meantime, the dynamic characteristics of PGT are affected by the tooth profile errors through affecting the mesh phase.

2.2.2. Eccentricity error

Eccentricity may be introduced during manufacture, or during set-up via shaft misalignment, imperfections in grub screw (set screw) tightness or ill- fitting bearings [22]. Moreover, eccentricity errors generate dynamic excitations and complex modulations which may downgrade the overall dynamic performance. To classic parallel axis gears, PTGs can be very sensitive to eccentricities, even in static conditions, when no central member is allowed to float. Chaari et al. [34] studied the effects of the eccentricity error (run-out error) on PGT dynamic response. He concluded that an exciting force is produced by the eccentricity error, which is expressed by Eq. (3), (4):

3

$F\left(t\right)=e_{sn}\left(t\right)K_e\left(t\right),$

4

$e_{sn}\left(t\right)={\stackrel{-}{e}}_s\mathrm{s}\mathrm{i}\mathrm{n}\left(\frac{2\pi f_e}{{{\rm Z}}_s}t+{\xi }_{sn}\right).$

Gu and Velex [35] concluded that the eccentricity error could lead to contact loss at the critical speed. As far as dynamic tooth loads are considered, eccentricity errors do not introduce additional critical speeds but lead to more complex response spectra with modulation sidebands. As shown in Fig. 4, the eccentricity error increases the gear load ratio, which causes the gear tooth to bear excessive dynamic load and eventually leads to transmission failure. Furthermore, the gear with eccentricity errors, which subjected the varying mesh stiffness, can produce larger load ratio than the gear subjected constant mesh stiffness.

Fig. 4Effect of eccentricity error on gear load [35]

a) Maximum and minimum tooth load ratio of sun/planet gear

b) Maximum load ratio of sun/planet gear with different eccentricity error

2.2.3. Misalignment

The misalignment of PGT varies with time, which results in increasing the vibration, the time-varying contact stress and the probability of the failure of PGT, and reducing the gear life [36]. Litvin [37] reminded that due to misalignment the line contact of tooth surfaces is turned out into point contact. Litvin et al. [38] concluded that misalignment can cause the discontinuous transmission errors, and the bearing contact should be oriented longitudinally to increase the contact ratio. Thus the shift of bearing contact caused by misalignment is reduced. Crowther et al. [36] pointed out that when the bearing clearance is optimal, the contact stress amplitude can be decreased and a mean stress is achieved (Fig. 5). The gears are still misaligned, but this misalignment is now consistent with the rotation and can be corrected with lead slope adjustments.

Fig. 5Planet gears bearing maximum contact stress with carrier rotation after planet gears bearing clearance optimization [36]

Through the analysis of Crowther et al. [36], the misalignment is relative to bearing clearance of PGT, so the misalignment can be reduced by optimizing the bearing clearance.

Vecchiato [39] applied the new ideas of tooth contact analysis to prove that an isostatic PGT is a self-regulated system because of the existence of floating gears. He considered an isostatic planetary gear train is not sensitive to most misalignment and has capable of self-regulation in order to compensate for various alignment errors.

2.3. Tooth profile modification (TPM)

Excessive gear tooth deflection due to applied torque causes undesirable tooth contact patterns and increases gear vibration, noise, and contact stress. TPM is extensively used to compensate for the elastic gear and tooth deflection from the applied torque. Moreover, TPM can improve load distribution of PGT [40], eliminate the tooth elastic deformation, increase wear resistance and reduce gear contact stress. Meanwhile Abousleiman and Velex [7] showed that tip relief greatly reduces dynamic tooth loads and displacement amplitudes by the numerical simulations of the PGT model. Depending on the amount of mesh stiffness fluctuation and the mesh phase, different TPMs are required to minimize the gear vibration [41]. The changing of the sun-planet mesh stiffness with regard to TPM is shown in Fig. 6.

Fig. 6Sun-planet mesh stiffness with TPM

TPM is used to optimize contact patterns, to compensate manufacturing errors, and to reduce gear dynamic destruction. Liu and Parker [42] concluded that for the multimesh gear trains, the dynamic effect of the TPM is commonly correlated with the static transmission error. Furthermore, the TPM of individual mesh gear is correlated with mesh stiffness variations and contact ratios. The gear contact pattern is mainly affected by the TPM and dynamic response [41, 42].

To analyze the actual effect of the TPM on vibration reduction, Wu Y. J. et al. [43] studied the vibration level reduction under different working conditions through an experiment, as illustrated in Fig. 7.

Fig. 7Experimental vibration reduction of TPM

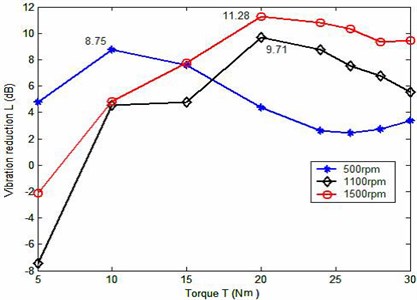
Fig. 6 shows that the effect of the presented TPM is not independent with the working conditions. With the increase of the torque, the effect of the TPM on vibration reduction is different for each speed, but the trends of the vibration reduction are almost the same for different speeds.

The TPM may deviate from the optimal area along with the change of mesh phase during optimization, and considering the interaction between the two gears mesh is necessary. According to Liu and Parker [42],the TPM for the minimal static transmission error (STE) depends only on the load and mesh stiffness of the single mesh. The optimal TPM for the minimal dynamic transmission error (DTE) is determined by mesh stiffness variations, contact ratios, mesh phase, and vibration modes. The TPM for the minimal STE does not necessarily lead to the minimal DTE, and it is proved by Bahk and Parker [41] by experimental verification.

2.4. Mesh stiffness

Gear mesh stiffness is a parametric excitation, and the effect of its dynamic excitation on gear dynamic characteristics is one of the main points of this study. According to Kahraman and Blankenship [44] and Chaari [45] mesh stiffness variation is one of the major sources of gear vibration. The time-varying mesh stiffness is a periodic function caused by the change in the number of contact tooth pairs and the contact positions of the gear teeth [46]. Experiments [47]demonstrated that the large amplitude vibration is induced by parametric instability wherein the gear mesh frequency equals twice the natural frequency (primary instability). Mesh stiffness directly affects the gear deformation and transmission error. Therefore, gear resonance excited by the harmonics of the transmission error fundamentally arises from the mesh stiffness variation [47, 48].

Liang et al. [46] stated that when to evaluate the mesh stiffness of PGT, the Hertzian contact stiffness, bending stiffness, axial compressive stiffness and shear stiffness should be considered. By studying the vibration response of the single-stage ear pair, Tian et al. [49] concluded that for the single-tooth-pair mesh duration, the total mesh stiffness is expressed as:

where subscripts 1 and 2 represent the pinion and the corresponding gear, respectively, $k_h$ is the Hertzian contact stiffness and $k_h=\pi EL/4\left(1-v^2\right)$, $k_b$is the bending stiffness and:

$k_a$ is the axial compressive stiffness and:

$k_s$ is the shear stiffness and:

${\alpha }_2$ is the half tooth angle on the base circle of the external gear, ${\alpha }_1$ is the angle between the force component $F_b$ and the acting force $F$ which can be decomposed into two orthogonal component forces: $F_a$ and $F_b$ [46] (Fig. 8).

For the double-tooth-pair mesh duration, there are two pairs of gears mesh at the same time. The total mesh stiffness is expressed as [49]:

where $i=$ 1 for the first pair of mesh teeth and $i=$2 for the second pair.

According to the above equations, $F_{spn}$ is the dynamic gear mesh forces which can be expressed as [50]:

where $k_{spn}$ and $c_{spn}$ represents the $n$th sun-planet gear mesh stiffness and mesh damping, respectively, and ${\mathrm{\delta }}_{spn}$ denotes the compression of the nth sun-planet mesh spring.

Fig. 8Elastic force on the external gear tooth [46]

The instability of the mesh stiffness of PGT is sensitive to the contact ratio and mesh phase, and the instability can be inhibited by changing the two parameters [51, 52]. Lin and Parker [51, 52] concluded that the parametric excitation from the time-varying mesh stiffness causes instability and severe vibration under certain operating conditions (that is, mesh frequency and stiffness variation amplitude).

2.5. Effects of other factors

In addition to the aforementioned parameters, the amount of planet gears [53], pressure angle [54], tooth width [55], clearances [56, 57], backlash [58] and bearing stiffness [59] also affect the dynamic behavior of the PGT.

For instance, the backlash, which is essential for assembly of the gears [60], namely the clearance, tends to make gear systems exhibit typical nonlinear dynamic behavior, undesirable noise and vibration problems [56, 61, 62]. Furthermore, with the increasing of the backlash, the tooth contact force is increased and the contact time is reduced (Fig. 7) [58]. Litvin et al. [64] developed new types of PGT and the new designs were based on regulating backlash between the gears, which is to achieve almost uniform load distribution.

Fig. 9Gear contact force with different backlash [58]