Wave barriers for the reduction of railway induced vibrations. Analysis in tracks with geometric restrictions

3.1. Real data acquisition

To calibrate and validate the numerical model it was necessary to measure accelerations in two different points in the track. For this reason two different data sets were obtained: one was used to calibrate the model estimating the unknown parameters and the other was employed to validate the results previously obtained. Finally, a model representing the vibrations behavior is obtained. Measured points are 0.2 and 0.7 m distant from the outer side of the rail and over them accelerometers were placed as shown in Fig. 3. Devices employed in the data acquisition were triaxial accelerometers Sequoia Fast Tracer™ with the characteristics indicated in Table 2.

Fig. 3Accelerometers position at 0.2 and 0.7 m from the outer side of the rail

3.2. FEM model implementation

ANSYS Multiphysics/LS-DYNA V.14 software has been used to perform the numerical model. The equation of the system dynamics according to the Rayleigh damping theory is:

In which $\left[M\right]$ is the global mass matrix, $\left[C\right]$ the damping matrix, $\left[K\right]$ the stiffness matrix, $\mathbf{u}$ the displacements vector, $\dot{\mathbf{u}}$ the velocities vector and $\ddot{\mathbf{u}}$ the accelerations vector. The term $\left\{{\mathbf{F}}^{\mathrm{\alpha }}\left(\mathrm{t}\right)\right\}$ represents the external forces exciting the system and inducing the accelerations.

In order to simplify Eq. (1) it is considered that the damping matrix $\left[C\right]$ only contains the stiffness term as in Real et al. [11]. In this manner, it can be considered that:

The global damping $\beta$ coefficient is unknown so it will be also included in the model calibration process to determine its value.

To calculate the vibration response induced by the whole train, the superposition principle is applied. Hence, the response produced by a single axle is calculated and then extended in time taking into consideration the axle distribution in the train and the driving speed. This method has already been used by, among others, El Kacimi [12] to extend the response of a single bogie to the whole train and Real et al. [13] in an analogous case to the studied here.

Finally, it must be stated that the frequency range studied with the model varies between 2 and 50 Hz. This interval was pointed by Griffin (1996) [14] as the most potentially dangerous to people in the track surroundings. In addition, the frequency range limits determine the model dimensions and also the model elements size as explained in [11].

3.3. Model calibration and validation

There exist two unknown parameters in the model: Young modulus of the graded aggregate and the global damping $\beta$ coefficient. To calibrate the model, different combinations of these values are proposed based on previous experiences and taking into account the materials present in the substructure in this case. Different accelerograms obtained from the different combinations are compared with real registers obtained at the point located 0.2 m away from the outer side of the rail. Then, the combination that best fits the real registers is chosen. In Table 3, the final values of the unknown parameters calibrated are shown.

Fig. 4Numerical model validation. Comparison between real registers (black) and model results (gray)

To validate the model, the other set of registers is taken and compared with the data obtained from the numerical model. In Fig. 4, it can be seen how the real and calculated peak values (positive and negative) are very similar and these maximums and minimums in the graph corresponding to the axle passing occur at the same time. Moreover, in both cases three bodies in the graph corresponding to the three bogies of the vehicle are distinguished, being the shape very similar in every case. From this verification it is concluded that a numerical model able to represent the vibrations in this track is available. Subsequently, the suitability of the wave barriers to mitigate the vibrations can be studied.

4.1. Trench parameters selection

Geometrical characteristics of the trenches (width ($W$), depth ($D$) and position relative to the track axis ($L$)) have been traditionally defined depending on the dominant Rayleigh wave length ${\lambda }_{Rdom}$. In order to calculate this wavelength it is necessary to know the Rayleigh waves speed in the ground $V_R$ (that depends on the mechanical properties of the sands displayed in Table 1) and on the dominant frequency $f_{dom}$:

Dominant frequency $f_{dom}$ is linked to the load transmitted by the passing wheel. It can be calculated as the quotient of the vehicle speed and the dimension of the elements containing the nodes in which loads are applied [11]:

Given the values of $V_{train}=$9.72 m/s; $d_{element}=$0.5 m and $V_R=$137 m/s, it is obtained a dominant wavelength ${\lambda }_{Rdom}=$7.05 m.

4.2. Filling materials selection

Open trenches have been traditionally considered more effective than the in-filled ones in both homogeneous soil (Ahmad and Al-Hussaini [15] and Beskos [18]) and layered soil (Çelebi et al. [6] and Real et al. [11]). However, the authors always consider that open trenches are limited because of the required support of the vertical walls of the trench in non cohesive materials or deep trenches. This drawback led to the development of in-filled trenches in which the wall support is ensured. A momentous study was performed by Hung et al. [9] in which vibration due to the quasi-static load was separated from that caused by the dynamic loads. From this consideration, it was deduced that the open trenches were suitable to mitigate the vibrations induced by dynamic loads while in-filled trenches perform better attenuating vibrations caused by the moving static load. To simulate the conditions of the open trenches overcoming the support problem, Massarsch [19] used gas cushions as filling material achieving excellent attenuation results.

For in-filled trenches, the election of the most suitable material has also been discussed by numerous authors. Yang and Hung [8] and Real et al. [11] declared that stiff materials have more mitigation power than soft materials. On the other hand, Çelebi et al. [6] and El Naggar and Chebab [10] pointed that soft materials such as bentonite produce greater isolation. The use of soft filling materials was also studied by Adam and Von Erstorff [17] concluding also that these type works better than stiff materials. In addition, from this research it was evidenced the small influence of the Poisson ratio and the density of the filling material on the mitigation power; for this reason all the filling materials are chosen taking into account their stiffness. Finally, it should be commented the research of Alzawi and El Naggar [20] in which geofoam barriers were discontinuously placed. In this manner, good attenuation results were obtained reducing the amount of filling material and the excavation volume as well.

4.3. Definition of the different scenarios

Due to the large variety of available results in previous publications regarding the different mitigation barrier type (open/in-filled or active/passive) and, in the case of the in-filled trenches, considering different materials, diverse scenarios are analyzed in this study. The support of the walls of the open trenches is provided by sheet piles while in the case of in-filled trenches, two different materials are considered: reinforced concrete and expanded polystyrene (EPS).

As previously said, the material stiffness plays an important role in the isolation power of the trench. For this reason, the materials chosen as filling materials have very different Young modulus: reinforced concrete ($E_C=$2485 MPa) and expanded polystyrene ($E_{EPS}=$ 2 MPa).

According to Ahmad and Al-Hussaini [15], when the Rayleigh wave reaches the trench (either open or in-filled) three different phenomena occur: part of the incident wave is reflected moving back towards the source; another part of the Rayleigh wave is transmitted beyond the trench and finally, due to the scattering power of the trench, part of the incident Rayleigh waves are converted into body waves. These body waves (P and S waves) can either be reflected or transmitted by the trench as in the case of Rayleigh waves. Generally, in-filled trenches allow wave transmission while reflection prevails in open trenches.

In-filled trenches can be classified according to the impedance ratio (IR). This dimensionless parameter gives the relationship between the mechanical properties of the filling material and the soil into which is embedded and has been found a key parameter on mitigation by several authors as explained below:

where ${\rho }_f$ and ${\rho }_s$ are respectively the filling and soil densities and ${\mu }_f$, ${\mu }_s$ the velocity of the Rayleigh waves in each medium. Values to calculate the IR of the different filling alternatives are shown in Table 4.

As Yang and Hung [8] and Hung and Ni [21] demonstrated, the attenuation power of in-filled trenches strongly depends on the filling material. Since results worsen as the IR is closer to 1 the filler must provide impedance ratios far from this value. In the studied cases, an IR of 15.8 is obtained for the concrete filling and an IR equal to 0.009 for the EPS. This fact also explains the different results obtained for the filling material by different authors in section 4.2.

Another consideration deals with the wave transmission through different interfaces in trenches. A broad approximation of the real behavior of the impinging waves may be obtained considering only perpendicular incidence on the interface. In this case, the refraction coefficient that accounts for the transmitted waves from the solid medium $i$ to the solid medium $j$ is defined as [22]:

Being $\rho$ the density and $\mu$ the Rayleigh wave velocity of each medium. These parameters are shown in Table 4. Considering that there exist two interfaces in each trench, the transmission coefficient for concrete as filling material is $B_{Concrete}=\text{0.223}$ and for the EPS filler $B_{EPS}=\text{0.038}$. Although these results have been calculated considering both interfaces for each trench; perpendicular wave incidence; absence of wave mode conversion and neglecting the wave damping into the trench, they may provide an approximated vision of the real behavior of the filling materials as in Real et al. [11]. With these simplifications of the actual performance, it is predicted that EPS would reduce more the amplitude of the incident wave than concrete because of the lower transmission coefficient of the first.

Fig. 6Combined filling materials in 0.5 m wide trenches: a) EPS-CO-EPS and b) CO-EPS-CO

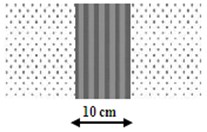
a)

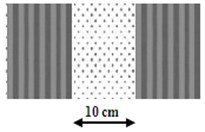
b)

Additionally, combinations of the different filling materials are tested to analyze the effect of the mixed materials on the final results (see Fig. 6). The part of the Rayleigh wave which is transmitted, reflected or converted into body waves is expected to be different from the homogeneous filling cases and therefore the trench behavior altered as well.

Furthermore, all the filling materials as well as the open trenches have been tested in the two possible locations for the trenches: active and passive isolation. The 3D FEM model scheme is shown in Fig. 7.

To evaluate the efficacy of the different alternatives, results obtained from the different scenarios are compared with the accelerations obtained in the checkpoints in the reference case, i.e. without any trench. This comparison is made using the amplitude reduction ratio, which is defined as:

where $A_I$ is the maximum acceleration in each scenario and $A_0$ is the maximum amplitude in the reference case presented in Table 5. Thus, the lower the amplitude reduction ratio $A_{RR}$ is, the better attenuation result is achieved.

Fig. 7Location of the different types of trenches: a) active isolation and b) passive isolation

a)

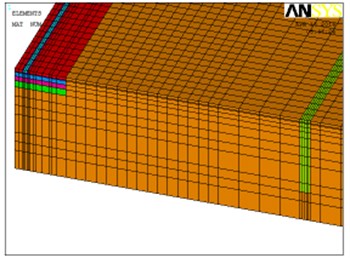
b)

4.4. Discussion of the results

In this section all the results obtained by the FEM model are discussed and justified. The amplitude reduction ratio is calculated for each alternative for the 4 defined checkpoints in the cases of active isolation and passive isolation. Moreover, the accelerograms corresponding to the open trench and the in-filled trenches with concrete and EPS are also presented to provide a better understanding of the wave behavior when a trench is implemented.

4.4.1. Active isolation

As it can be observed in Table 6, the open trench provides the best results in active isolation of the urban area. However, due to wave reflection phenomenon in the interface, an amplification of the vibration is produced in the checkpoint 1. This amplification may have negative effects on the railway infrastructure or even affect the comfort of the passengers. This undesired effect must be specially considered in ballasted tracks where the degradation of the ballast layer may occur as a consequence of the vibrations according to López Pita [23].

Table 6Active isolation ARR results

Active isolation			Checkpoint
		1	2	4
Filling material	Open	1.62	0.10	0.07
	Concrete	0.53	0.46	0.59
	EPS	0.94	0.22	0.23
	EPS-CO-EPS	1.21	0.11	0.17
	CO-EPS-CO	0.58	0.20	0.35

For a better understanding of the dynamic behavior, the calculated accelerograms corresponding to the active isolation have been represented in Fig. 8. In Fig. 8(a), it can be observed how the open trench causes the highest acceleration because of the wave reflection explained before. On the other hand, the concrete absorbs the wave transmitting it to the other side of the trench where the higher accelerations correspond to this type of in-filled trench. Moreover, the lower acceleration at this point corresponds to the open trench since most of the energy has been reflected backwards the track. Trenches filled with EPS provide intermediate results in both checkpoints when compared to concrete filling and the open trench in active isolation.

Fig. 8Calculated accelerograms in checkpoints 1 a) and 2 b) with active isolation

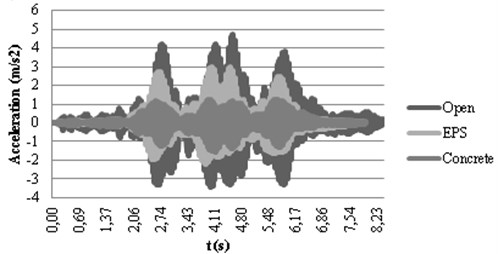
a)

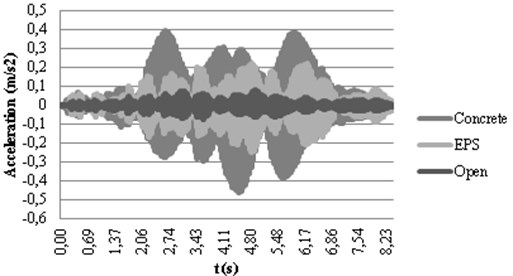
b)

When comparing Fig. 8(a) and (b), it can be seen how the original shape of the accelerogram, where the effect of the different bogies could be observed as in Fig. 4, fades after the trench. This effect is more pronounced in the case of the open trench since the gap totally disrupts the propagation path of the Rayleigh waves and forces the waves to pass beneath the bottom of the trench, altering the vibration response after the trench.

The concrete trench reduces the vibrations about 50 % both in the track and in the urban area, thus achieving an equilibrated solution in both locations. EPS obtains good attenuation results in checkpoint 4 without increasing the accelerations in the track structure. A better solution is achieved by using the combination CO-EPS-CO that drops to 0.154 m/s² the peak acceleration in checkpoint 4, maintaining low levels in the track.

4.4.2. Passive isolation

Passive isolation, i.e. when the trench is placed in the vicinity of the isolated element, globally presents better results than the active isolation. The mitigation power is more marked in the case of open trenches and stiff filling materials (concrete and CO-EPS-CO). However, soft materials (EPS and EPS-CO-EPS) do not yield as good isolation results as the rest of the materials in checkpoint 4. As it happened in the active isolation, wave amplification is produced before the open trench but, in this case, the possible negative effect on the service road is not as dangerous as on the track in the active isolation.

Fig. 9Calculated accelerograms in checkpoints 3 a) and 4 b) with passive isolation

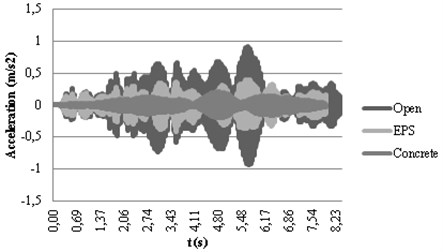
a)

b)

In Fig. 9(a) this effect of amplification for the open trenches in the point 3 can be ascertained since open trenches yield the highest acceleration peak at this point. Results agree with the explained for active isolation regarding the dynamic behavior of the different materials. However, the alteration of the accelerograms shape is more evident in passive isolation. This fact can be explained by the large distance existing between the passive trench and the vibration source where the soil damping mechanisms affect the wave propagation.

In passive isolation, differences obtained in checkpoint 4 for the different filling materials do not vary as much as in the active isolation. An explanation to this fact can be found in the Rayleigh wave propagation path: the Rayleigh wave is completely developed when it reaches the passive trench and a large amount of the energy propagates beneath the bottom of the trench. In the case of the active isolation, the trench is located close to the source and it means that the Rayleigh wave is not yet too deep and propagates in shallow depths. Therefore, in active isolation, the effect of the filling materials is more determining than in the case of passive isolation.

Table 7Passive isolation ARR results

Passive isolation		Checkpoint
		1	3	4
Filling material	Open	0.94	1.42	0.05
	Concrete	0.97	0.43	0.35
	EPS	1.02	0.85	0.42
	EPS-CO-EPS	0.94	0.98	0.53
	CO-EPS-CO	0.94	1.14	0.30